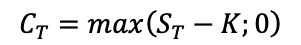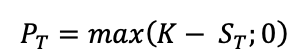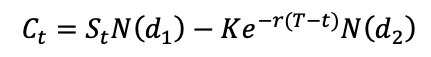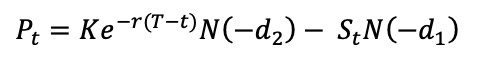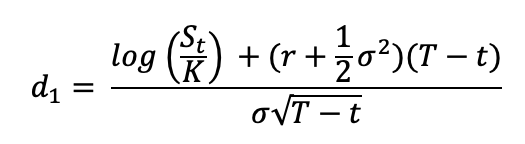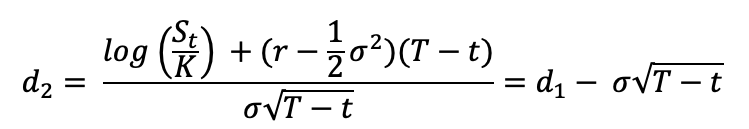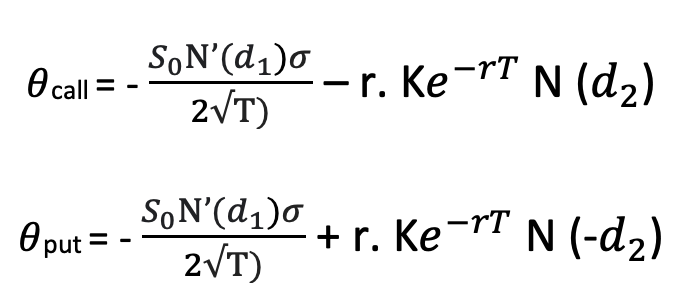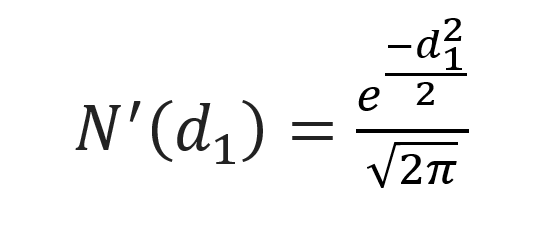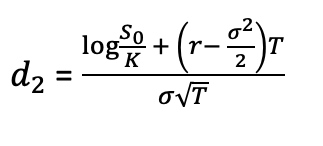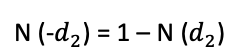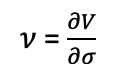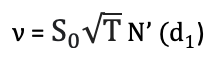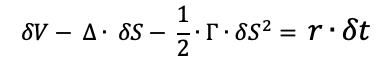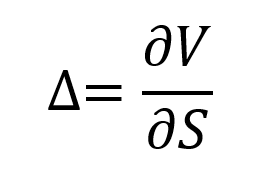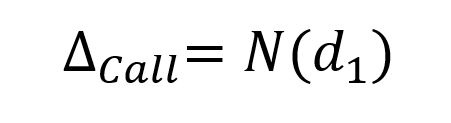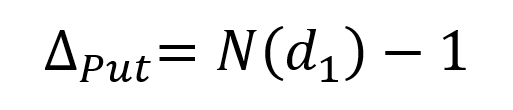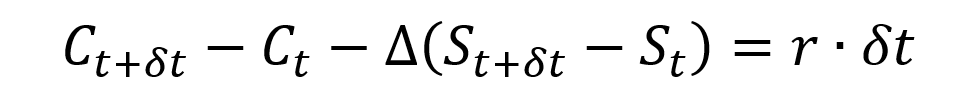Black-Scholes-Merton option pricing model

February 4, 2024March 3, 2022 by Jayati WALIA

In this article, Jayati WALIA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) explains the Black-Scholes-Merton model to price options.

The Black-Scholes-Merton model (or the BSM model) is the world’s most popular option pricing model. Developed in the beginning of the 1970s, this model introduced to the world, a mathematical way of pricing options. Its success was essentially a starting point for new forms of financial derivatives in the knowledge that they could be priced accurately using the ideas and analyses pioneered by Black, Scholes and Merton and it set the foundation for the flourishing of modern quantitative finance. Myron Scholes and Robert Merton were awarded the Nobel Prize for their work on option pricing in 1997. Unfortunately, Fischer Black had died several years earlier but would certainly have been included in the prize had he been alive, and he was also listed as a contributor by Scholes and Merton.

Today, the Black-Scholes-Merton formula is widely used by traders in investment banks to price and hedge option contracts. Options are used by investors to hedge their portfolios to manage their risks.

Assumptions of the BSM Model

As any model, the BSM model relies on a set of assumptions:

The model considers European options, which we can only be exercised at their expiration date.
The price of the underlying asset follows a geometric Brownian motion (corresponding to log-normal distribution for the price at a given point in time).
The risk-free rate remains constant over time until the expiration date.
The volatility of the underlying asset price remains constant over time until the expiration date.
There are no dividend payments on the underlying asset.
There are no transaction costs on the underlying asset.
There are no arbitrage opportunities.

The BSM equation

The value of an option is a function of the price of the underlying stock and its statistical behavior over the life of the option.

A commonly used model is Geometric Brownian Motion (GBM). GBM assumes that future asset price differences are uncorrelated over time and the probability distribution function of the future prices is a log-normal distribution (or equivalently the probability distribution function of the future returns is a normal distribution). The price movements in a GBM process can be expressed as:

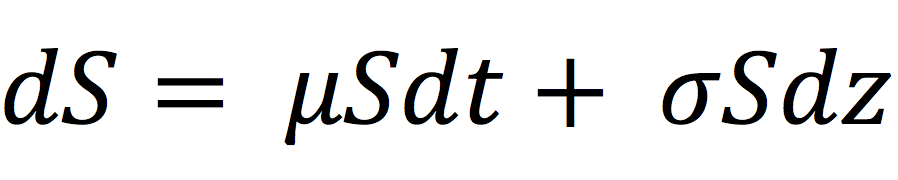
GBM equation

with dS being the change in the underlying asset price in continuous time dt and dX the random variable from the normal distribution (N(0, 1) or Wiener process). σ is the volatility of the underlying asset price (it is assumed to be constant). μdt represents the deterministic return within the time interval with μ representing the growth rate of asset price or the ‘drift’.

Therefore, option price is determined by these parameters that describe the process followed by the asset price over a period of time. The Black-Scholes-Merton equation governs the price evolution of European stock options in financial markets. It is a linear parabolic partial differential equation (PDE) and is expressed as:

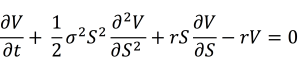
BSM model equation

Where V is the value of the option (as a function of two variables: the price of the underlying asset S and time t), r is the risk-free interest rate (think of it as the interest rate which you would receive from a government debt or similar debt securities) and σ is the volatility of the log returns of the underlying security (say stocks).

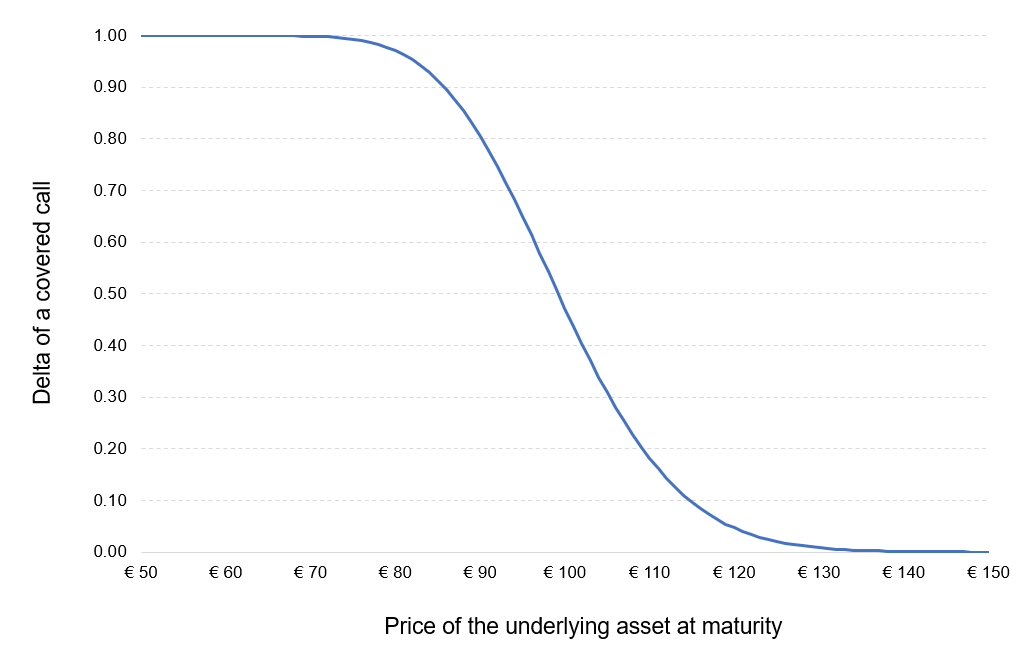
The key idea behind the equation is to hedge the option and limit exposure to market risk posed by the asset. This is achieved by a strategy known as ‘delta hedging’ and it involves replicating the option through an equivalent portfolio with positions in the underlying asset and a risk-free asset in the right way so as to eliminate risk.

Thus, from the BSM equation we can derive the BSM formulae that describe the price of call and put options over their life time.

The BSM formulae

Note that the type of option we are valuing (call or put), the strike price and the maturity date do not appear in the above BSM equation. These elements only appear in the ‘final condition’ i.e., the option value at maturity, called the payoff function.

For a call option, the payoff C is given by:

C_T = max⁡(S_T – K; 0)

For a put option, the payoff is given by:

P_T = max⁡(K – S_T; 0)

The BSM formula is a solution to the BSM equation, given the boundary conditions (given by the payoff equations above). It calculates the price at time t for both a call and a put option.

The value for a call option at time t is given by:

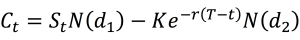
Call option value equation

The value for a put option at time t is given by:

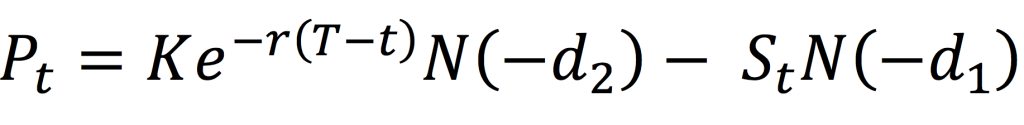
Put option value equation

where

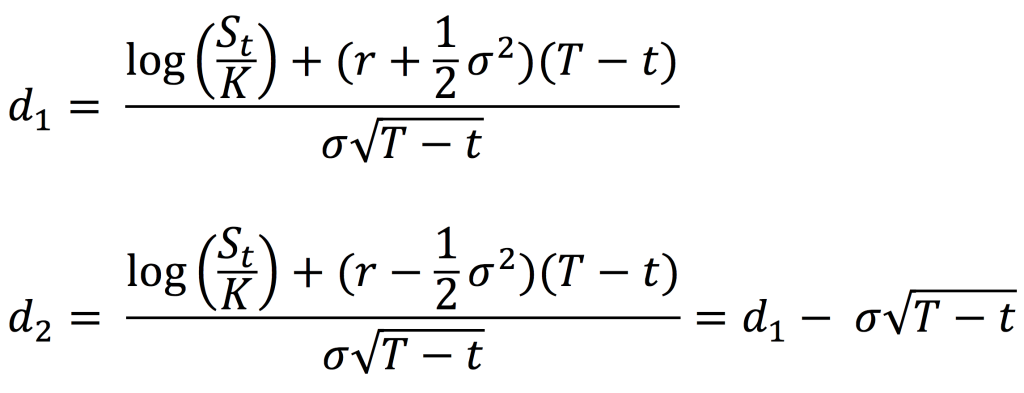
With the notations:
S_t: Price of the underlying asset at time t
t: Current date
T: Expiry date of the option
K: Strike price of the option
r: Risk-free interest rate
σ: Volatility (the standard deviation of the return on the underlying asset)
N(.): Cumulative distribution function for a normal (Gaussian) distribution. It is the probability that a random variable is less or equal to its input (i.e. d₁ and d₂) for a normal distribution. Thus, 0 ≤ N(.) ≤ 1

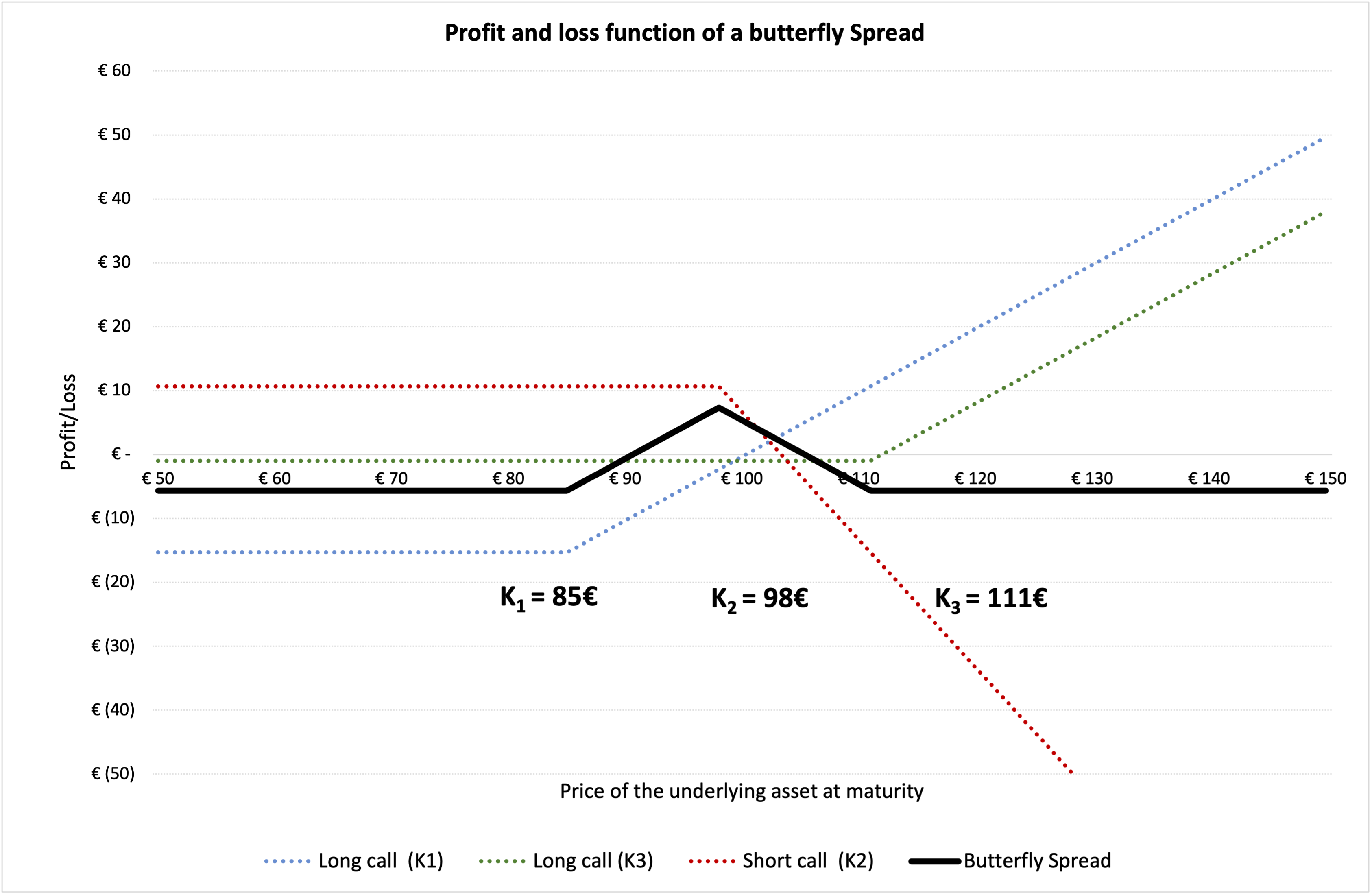
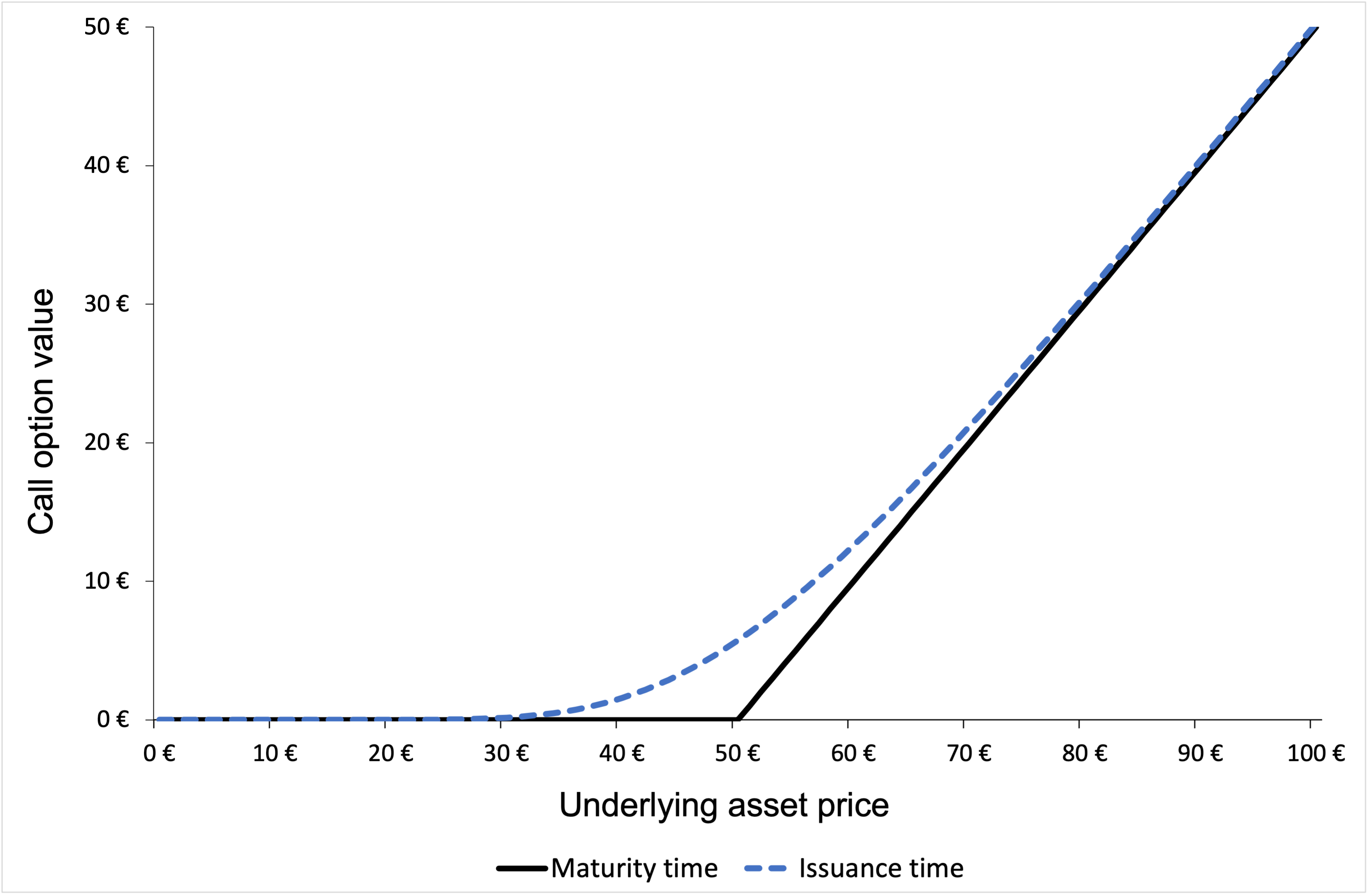
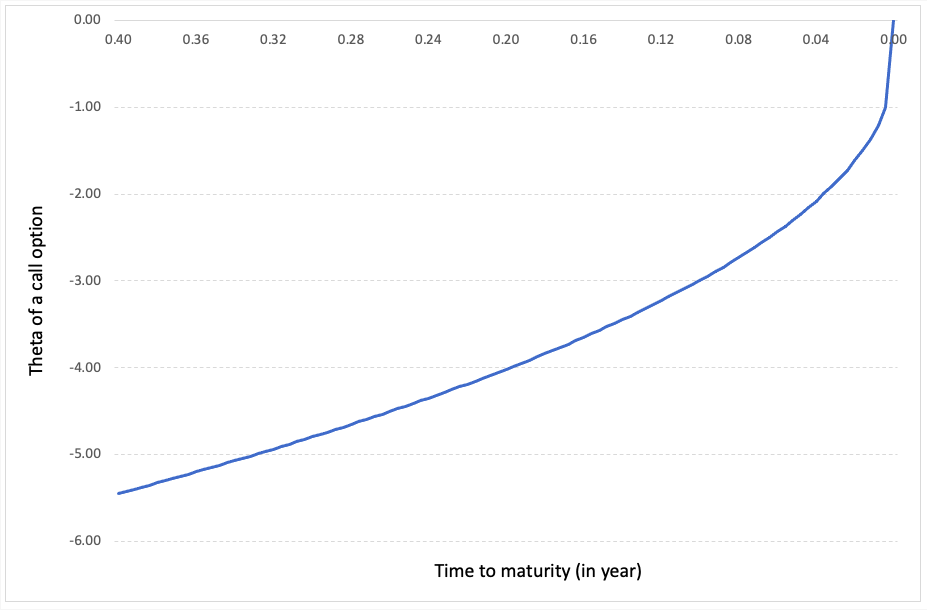
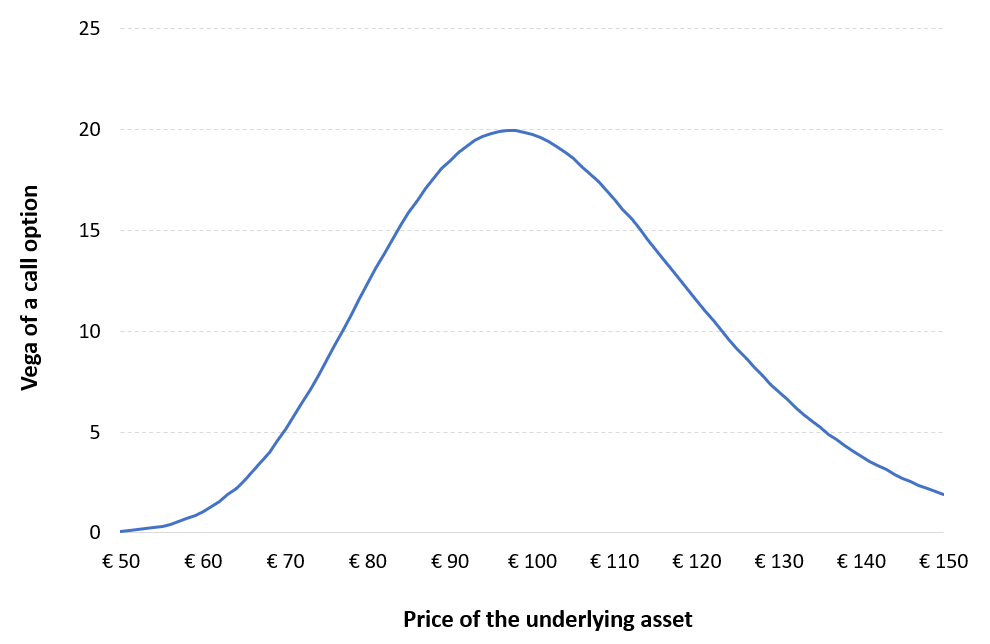
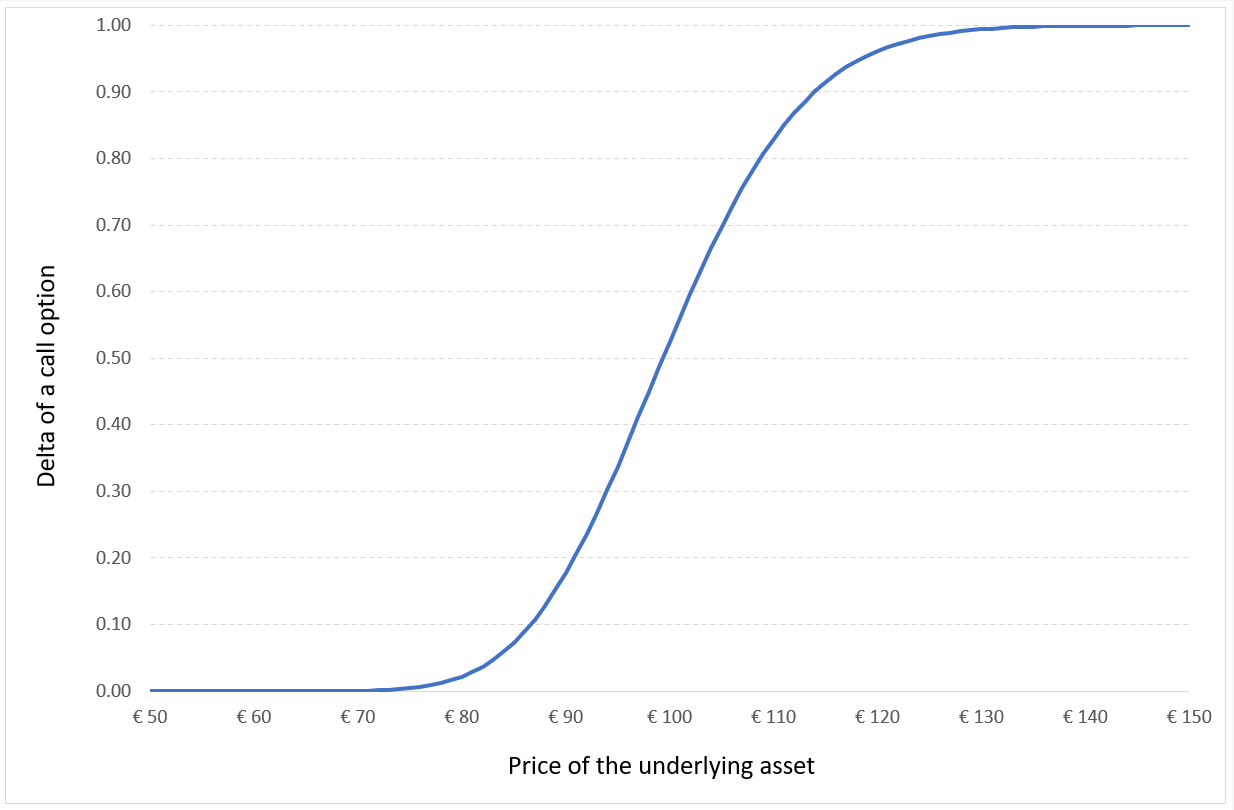
Figure 1 gives the graphical representation of the value of a call option at time t as a function of the price of the underlying asset at time t as given by the BSM formula. The strike price for the call option is 50€ with a maturity of 0.25 years and volatility of 50% in the underlying.

Figure 1. Call option value

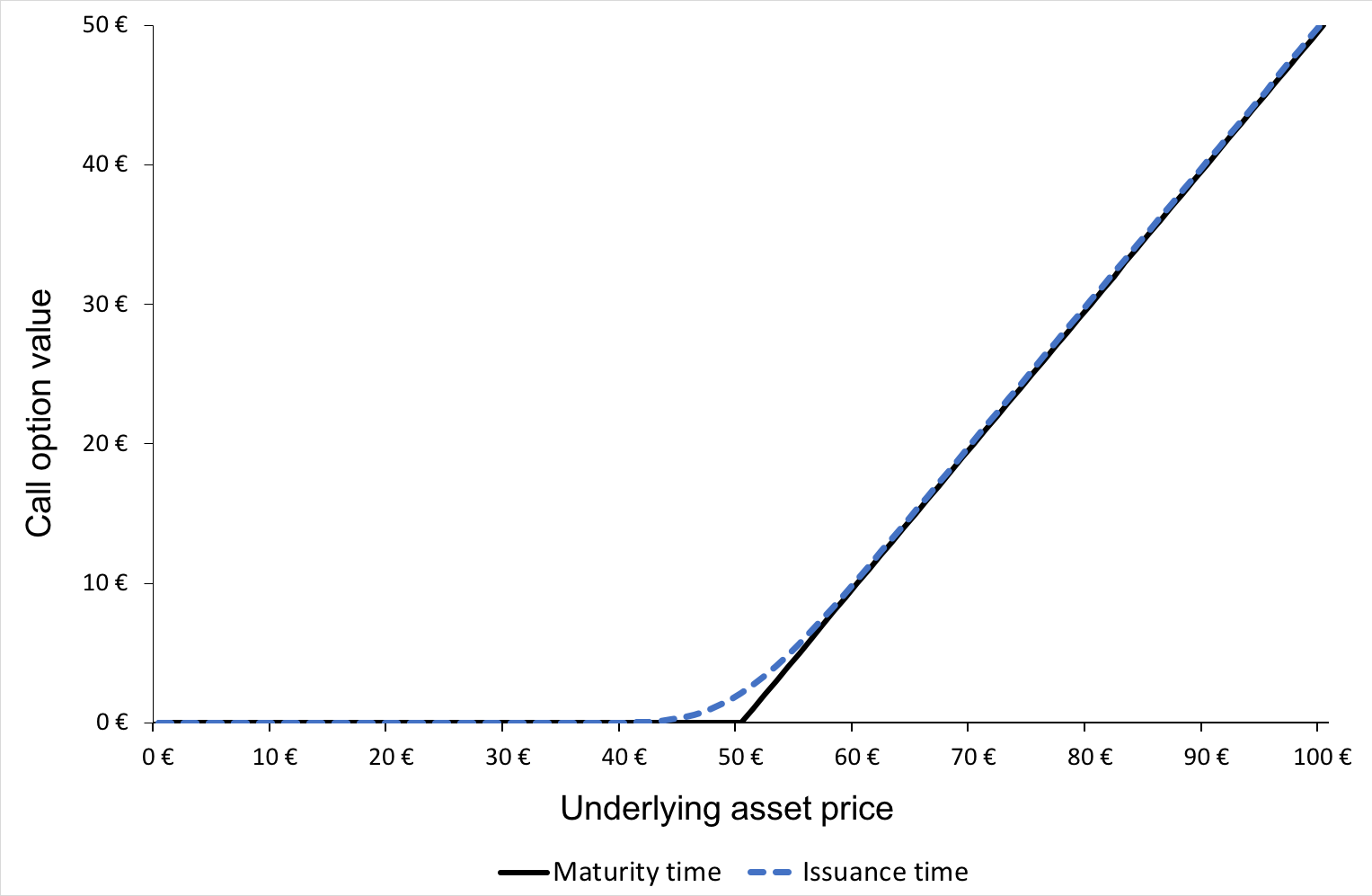
Source: computation by author.

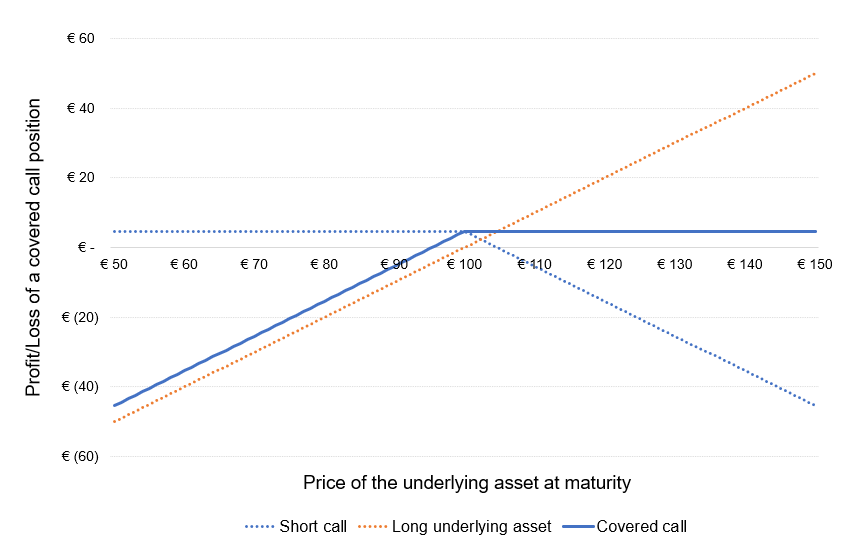
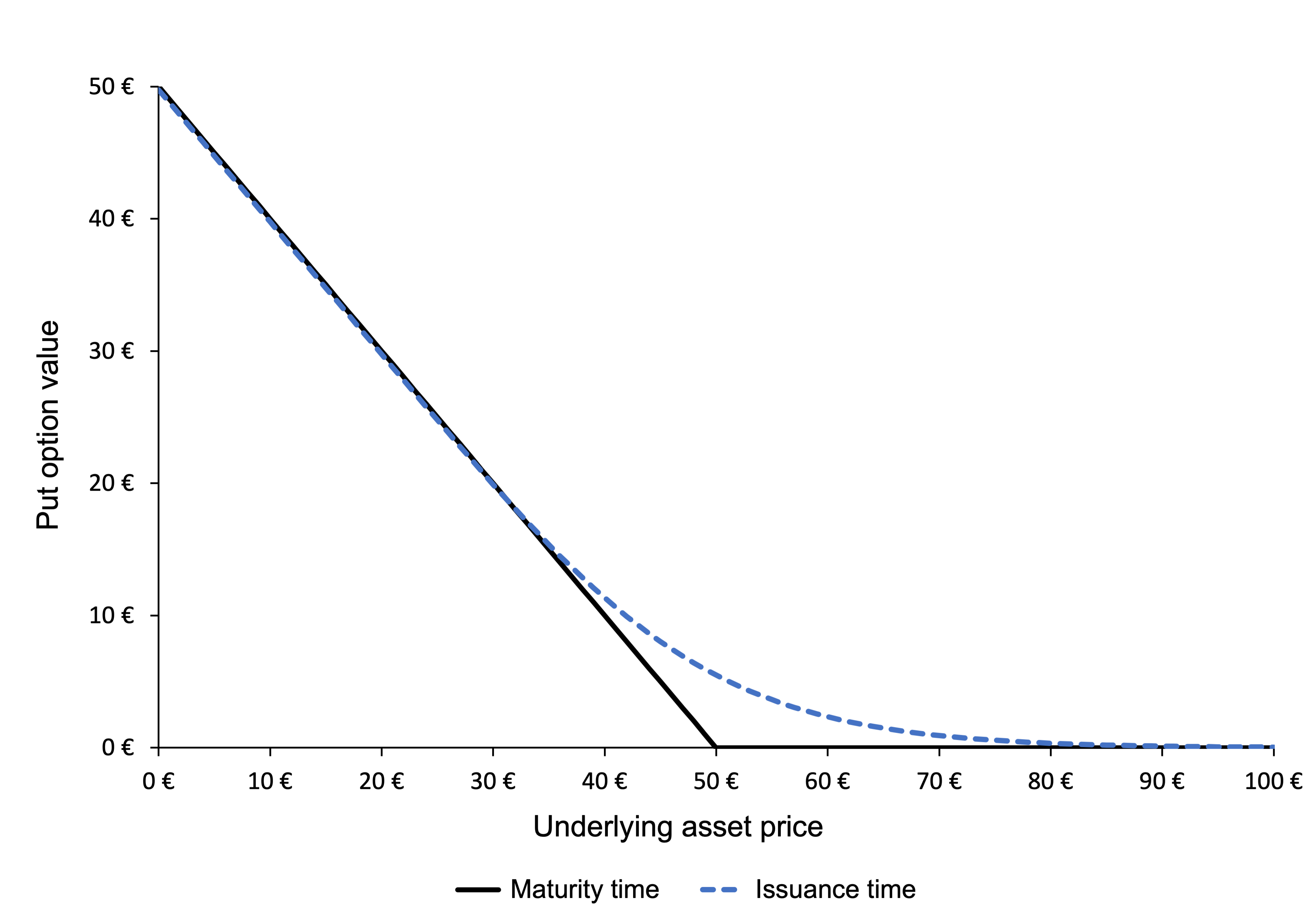
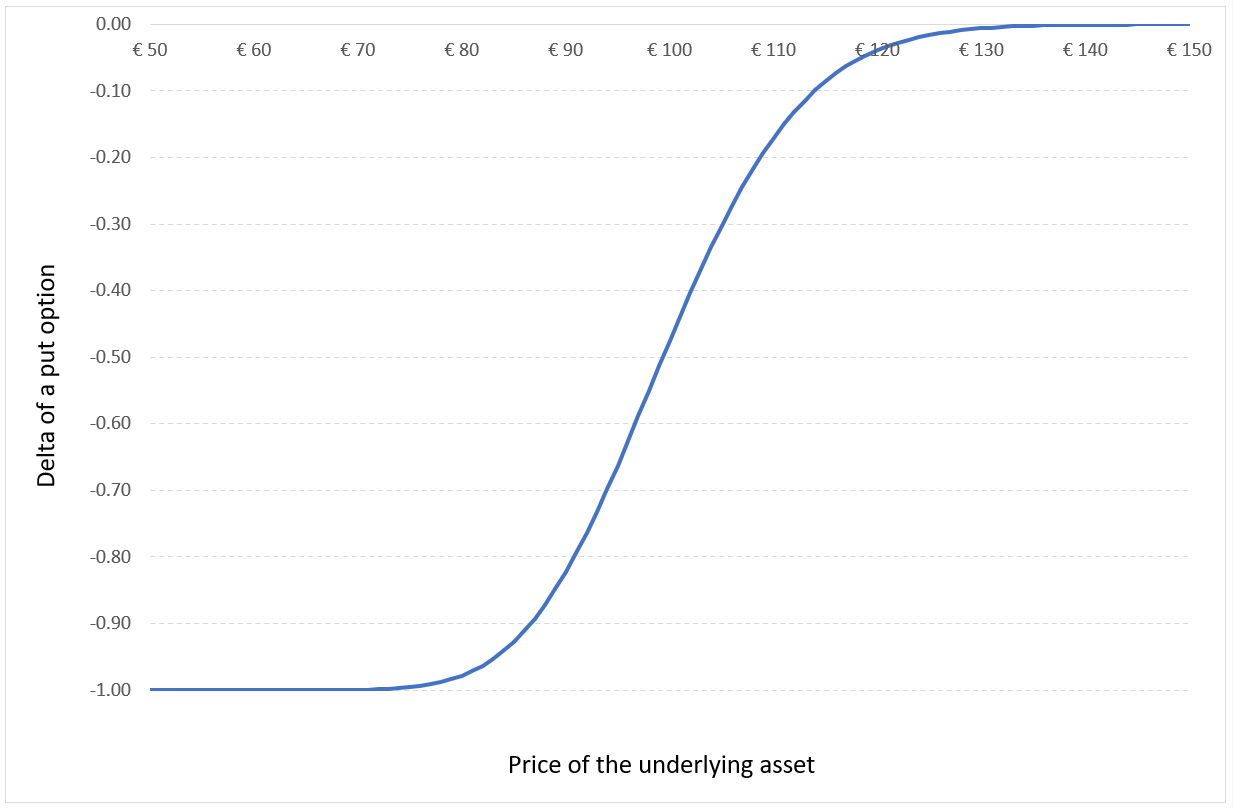
Figure 2 gives the graphical representation of the value of a put option at time t as a function of the price of the underlying asset at time t as given by the BSM formula. The strike price for the put option is 50€ with a maturity of 0.25 years and volatility of 50% in the underlying.

Figure 2. Put option value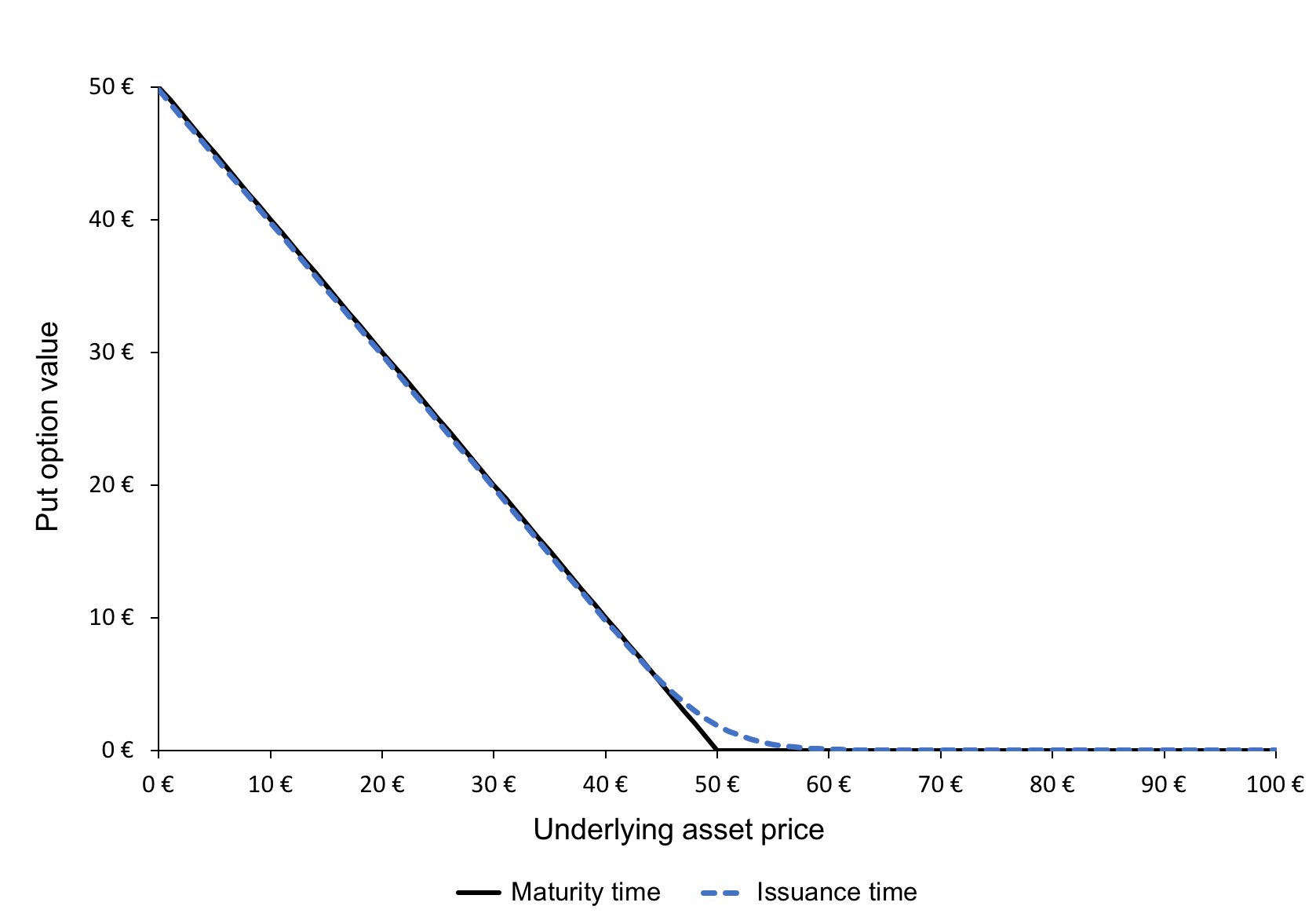
Source: computation by author.

You can download below the Excel file for option pricing with the BSM Model.

Some Criticisms and Limitations

American options

The Black-Scholes-Merton model was initially developed for European options. This is a limitation of the equation for American options which can be exercised at any time before the expiry date. The BSM model would then not accurately determine the option value (an important case when the underlying asset pays a discrete dividend).

Stocks paying dividends

Also, in reality, most stocks pay dividends, and no dividends was an assumption in the initial BSM model, which analysts now eliminated by accommodating the dividend yield in the formula if required.

Constant volatility

Another limitation is the use of constant volatility. Volatility is the measure of risk based on the standard deviation of the return on the underlying asset. In reality the value of an asset will change randomly, not with a specific constant pattern regarding the way it can change.

Finally, the assumption of no transaction cost neglects the liquidity risk in the market since transaction costs are clearly incurred in the real world and there exists a bid-offer spread on most underlying assets. For the most heavily traded stocks, this cost may be low but for others it may lead to an inaccuracy.

Useful resources

Black F. and M. Scholes (1973) The Pricing of Options and Corporate Liabilities The Journal of Political Economy 81, 637-654.

Merton R.C. (1973) Theory of Rational Option Pricing Bell Journal of Economics 4, 141–183.

About the author

The article was written in March 2022 by Jayati WALIA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).

Straddle and strangle strategy

January 12, 2022 by Akshit GUPTA

Straddle and Strangle

This article written by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) presents the strategies of straddle and strangle based on options.

Introduction

In financial markets, hedging is implemented by investors to minimize the risk exposure and maximize the returns for any investment in securities. While hedging does not necessarily eliminate the entire risk for an investment, it does limit or offset any potential losses that the investor can incur.

Option contracts are commonly used by investors / traders as hedging mechanisms due to their great flexibility (in terms of expiration date, moneyness, liquidity, etc.) and availability. Positions in options are used to offset the risk exposure in the underlying security, another option contract or in any other derivative contract. Option strategies can be directional or non-directional.

Directional strategy is when the investor has a specific viewpoint about the movement of an asset price and aims to earn profit if the viewpoint holds true. For instance, if an investor has a bullish viewpoint about an asset and speculates that its price will rise, she/he can buy a call option on the asset, and this can be referred as a directional trade with a bullish bias. Similarly, if an investor has a bearish viewpoint about an asset and speculates that its price will fall, she/he can buy a put option on the asset, and this can be referred as a directional trade with a bearish bias.

On the other hand, non-directional strategies can be used by investors when they anticipate a major market movement and want to gain profit irrespective of whether the asset price rises or falls, i.e., their payoff is independent of the direction of the price movement of the asset but instead depends on the magnitude of the price movement. There are various popular non-directional strategies that can be implemented through a combination of option contracts to minimize risk and maximize returns. In this post, we are interested in straddle and strangle.

Straddle

In a straddle, the investor buys a European call and a European put option, both at the same expiration date and at the same strike price. This strategy works in a similar manner like a strangle (see below). However, the potential losses are a bit higher than incurred in a strangle if the stock price remains near the central value at expiration date.

A long straddle is when the investor buys the call and put options, whereas a short straddle is when the investor sells the call and put options. Thus, whether a straddle is long or short depends on whether the options are long or short.

Market Scenario

When the price of underlying is expected to move up or down sharply, investors chose to go for a long straddle and the expiration date is chosen such that it occurs after the expected price movement. Scenarios when a long straddle might be used can include budget or company earnings declaration, war announcements, election results, policy changes etc.
Conversely, a short straddle can be implemented when investors do not expect a significant movement in the asset prices.

Example

In Figure 1 below, we represent the profit and loss function of a straddle strategy using a long call and a long put option. K₁ is the strike price of the long call i.e., €98 and K₂ is the strike price of the long put position i.e., €98. The premium of the long call is equal to €5.33, and the premium of the long put is equal to €3.26 computed using the Black-Scholes-Merton model. The time to maturity (T) is of 18 days (i.e., 0.071 years). At the time of valuation, the price of the underlying asset (S₀) is €100, the volatility (σ) of the underlying asset is 40% and the risk-free rate (r) is 1% (market data).

Figure 1. Profit and loss (P&L) function of a straddle position.

Source: computation by the author.

You can download below the Excel file for the computation of the straddle value using the Black-Scholes-Merton model.

Strangle

In a strangle, the investor buys a European call and a European put option, both at the same expiration date but different strike prices. To benefit from this strategy, the price of the underlying asset must move further away from the central value in either direction i.e., increase or decrease. If the stock prices stay at a level closer to the central value, the investor will incur losses.

Like a straddle, a long strangle is when the investor buys the call and put options, whereas a short strangle is when the investor sells (issues) the call and put options. The only difference is the strike price, as in a strangle, the call option has a higher strike price than the price of the underlying asset, while the put option has a lower strike price than the price of the underlying asset.

Strangles are generally cheaper than straddles because investors require relatively less price movement in the asset to ‘break even’.

Market Scenario

The long strangle strategy can be used when the trader expects that the underlying asset is likely to experience significant volatility in the near term. It is a limited risk and unlimited profit strategy because the maximum loss is limited to the net option premiums while the profits depend on the underlying price movements.

Similarly, short strangle can be implemented when the investor holds a neutral market view and expects very little volatility in the underlying asset price in the near term. It is a limited profit and unlimited risk strategy since the payoff is limited to the premiums received for the options, while the risk can amount to a great loss if the underlying price moves significantly.

Example

In Figure 2 below, we represent the profit and loss function of a strangle strategy using a long call and a long put option. K₁ is the strike price of the long call i.e., €98 and K₂ is the strike price of the long put position i.e., €108. The premium of the long call is equal to €5.33, and the premium of the long put is equal to €9.47 computed using the Black-Scholes-Merton model. The time to maturity (T) is of 18 days (i.e., 0.071 years). At the time of valuation, the price of the underlying asset (S₀) is €100, the volatility (σ) of the underlying asset is 40% and the risk-free rate (r) is 1% (market data).

Figure 2. Profit and loss (P&L) function of a strangle position.

Source: computation by the author..

You can download below the Excel file for the computation of the strangle value using the Black-Scholes-Merton model.

▶ All posts about Options

▶ Akshit GUPTA Options

▶ Akshit GUPTA The Black-Scholes-Merton model

▶ Akshit GUPTA Option Spreads

▶ Akshit GUPTA Option Trader – Job description

Useful resources

Academic research articles

Black F. and M. Scholes (1973) “The Pricing of Options and Corporate Liabilities” The Journal of Political Economy, 81, 637-654.

Merton R.C. (1973) “Theory of Rational Option Pricing” Bell Journal of Economics, 4, 141–183.

Books

Hull J.C. (2015) Options, Futures, and Other Derivatives, Ninth Edition, Chapter 10 – Trading strategies involving Options, 276-295.

Wilmott P. (2007) Paul Wilmott Introduces Quantitative Finance, Second Edition, Chapter 8 – The Black Scholes Formula and The Greeks, 182-184.

About the author

Article written in January 2022 by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).

Option Spreads

January 7, 2022 by Akshit GUPTA

Option Spreads

This article written by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) presents the different option spreads used to hedge a position in financial markets.

Introduction

In financial markets, hedging is implemented by investors to minimize the risk exposure for any investment in securities. While hedging does not necessarily eliminate the entire risk for an investment, it does limit or offset any potential losses that the investor can incur.

Option contracts are commonly used by traders and investors as hedging mechanisms due to their great flexibility (in terms of expiration date, moneyness, liquidity, etc.) and availability. Positions in options are used to offset the risk exposure in the underlying security, another option contract or in any other derivative contract. Option strategies can be directional or non-directional.

Spreads are hedging strategies used in trading in which traders buy and sell multiple option contracts on the same underlying asset. In a spread strategy, the option type used to create a spread has to be consistent, either call options or put options. These are used frequently by traders to minimize their risk exposure on the positions in the underlying assets.

Bull Spread

In a bull spread, the investor buys a European call option on the underlying asset with strike price K₁ and sells a call option on the same underlying asset with strike price K₂ (with K₂ higher than K₁) with the same expiration date. The investor expects the price of the underlying asset to go up and is bullish about the stock. Bull spread is a directional strategy where the investor is moderately bullish about the underlying asset, she is investing in.

When an investor buys a call option, there is a limited downside risk (the loss of the premium) and an unlimited upside risk (gains). The bull spread reduces the potential downside risk on buying the call option, but also limits the potential profit by capping the upside. It is used as an effective hedge to limit the losses.

Market Scenario

When the price of underlying asset is expected to moderately move up, investors chose to execute a bull spread and the expiration date is chosen such that it occurs after the expected price movement. If the price decreases significantly by the expiration of the call options, the investor loses money by using a bull spread.

Example

In Figure 1 below, we represent the profit and loss function of a bull spread strategy using a long and a short call option. K₁ is the strike price of the long call i.e., €88 and K₂ is the strike price of the short call position i.e., €110. The premium of the long call is equal to €12.62, and the premium of the short call is equal to €1.16 computed using the Black-Scholes-Merton model. The time to maturity (T) is of 18 days (i.e., 0.071 years). At the time of valuation, the price of the underlying asset (S₀) is €100, the volatility (σ) of the underlying asset is 40% and the risk-free rate (r) is 1% (market data).

Figure 1. Profit and loss (P&L) function of a bull spread.

Profit and loss (P&L) function of a bul spread

Source: computation by the author.

You can download below the Excel file for the computation of the bull spread value using the Black-Scholes-Merton model.

Bear Spread

In a bear spread, the investor expects the price of the underlying asset to moderately decline in the near future. In order to hedge against the downside, the investor buys a put option with strike price K₁ and sells another put option with strike price K₂, with K₁ lower than < K₂. Initially, this initial position leads to a cash outflow since the put option bought (with strike price K₁) has a higher premium than put option sold (with strike price K₂) as K₁ is lower than < K₂.

Market Scenario

When the price of underlying asset is expected to moderately move down, investors chose to execute a bear spread and the expiration date is chosen such that it occurs after the expected price movement. Bear spread is a directional strategy where the investor is moderately bearish about the stock he is investing in. If the price increases significantly by the expiration of the put options, the investor loses money by using a bear spread.

Example

In Figure 2 below, we represent the profit and loss function of a bear spread strategy using a long and a short put option. K₁ is equal to the strike price of the short put i.e., €90 and K₂ is equal to the strike price of the long put i.e., €105. The premium of the short put is equal to €0.86, and the premium long put is equal to €7.26 computed using the Black-Scholes-Merton model.

The time to maturity (T) is of 18 days (i.e., 0.071 years). At the time of valuation, the price of the underlying asset (S₀) is €100, the volatility (σ) of stock is 40% and the risk-free rate (r) is 1% (market data).

Figure 2. Profit and loss (P&L) function of a bear spread.

Profit and loss (P&L) function of a bear spread

Source: computation by the author.

You can download below the Excel file for the computation of the bear spread value using the Black-Scholes-Merton model.

Butterfly Spread

In a butterfly spread, the investor expects the price of the underlying asset to remain close to its current market price in the near future. Just as a bull and bear spread, a butterfly spread can be created using call options. In order to profit from the expected market scenario, the investor buys a call option with strike price K₁ and buys another call option with strike price K₃, where K₁ < K₃, and sells two call options at price K₂, where K₁ < K₂ < K₃. Initially, this initial position leads to a net cash outflow.

Market Scenario

When the price of underlying asset is expected to stay stable, investors chose to execute a butterfly spread and the expiration date is chosen such that the expected price movement occurs before the expiration date. Butterfly spread is a non-directional strategy where the investor expects the price to remain stable and close to the current market price. If the price movement is significant (either downward or upward) by the expiration of the call options, the investor loses money by using a butterfly spread.

Example

In Figure 3 below, we represent the profit and loss function of a butterfly spread strategy using call options. K₁ is equal to the strike price of the long call position i.e., €85 and K₂ is equal the strike price of the two short call positions i.e., €98 and K₃ is equal to the strike price of another long call position i.e., €111. The premium of the long call K₁ is equal to €15.332, the premium of the long call K₃ is equal to €0.993 and the premium of the short call K₂ is equal to €5.334 computed using the Black-Scholes-Merton model. The premium of the butterfly spread is then equal to €5.657 (= 15.332 + 0.993 -2*5.334), which corresponds to an outflow for the investor.

The time to maturity (T) is of 18 days (i.e., 0.071 years). At the time of valuation, the price of the (S₀) is €100, the volatility (σ) of stock is 40% and the risk-free rate (r) is 1% (market data).

Figure 3. Profit and loss (P&L) function of a butterfly spread.

Profit and loss (P&L) function of a butterfly spread

Source: computation by the author.

You can download below the Excel file for the computation of the butterfly spread value using the Black-Scholes-Merton model.

Note that bull, bear, and butterfly spreads can also be created from put options or a combination of call and put options.

▶ All posts about options

▶ Gupta A. Options

▶ Gupta A. The Black-Scholes-Merton model

▶ Gupta A. Option Greeks – Delta

▶ Gupta A. Hedging Strategies – Equities

Useful resources

Hull J.C. (2018) Options, Futures, and Other Derivatives, Tenth Edition, Chapter 12 – Trading strategies involving Options, 282-301.

About the author

Article written in January 2022 by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).

Covered call

December 11, 2021 by Akshit GUPTA

Covered Call

This article written by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) presents the concept of covered call used in equities option contracts.

Introduction

Hedging is a strategy implemented by investors to reduce the risk in an existing investment. In financial markets, hedging is an effective tool used by investors to minimize the risk exposure and change the risk profile for any investment in securities. While hedging does not necessarily eliminate the entire risk for any investment, it does limit the potential losses that the investor can incur.

Option contracts are commonly used by market participants (traders, investors, asset managers, etc.) as hedging mechanisms due to their great flexibility (in terms of expiration date, moneyness, liquidity, etc.) and availability. Positions in options are used to offset the risk exposure in the underlying security, another option contract or in any other derivative contract. There are various popular strategies that can be implemented through option contracts to minimize risk and maximize returns, one of which is a covered call.

Covered call

The covered call strategy is a two-part strategy that essentially involves an investor writing a call option on an underlying security while simultaneously holding a long position in the same underlying. This action of buying an asset and writing calls on it at the same time is commonly referred as ‘buy write’. By writing a call option, the investor locks in the price of the underlying asset, thereby enjoying a short-term gain from the premium received.

Market scenario

The covered call is generally ideal if the investor has a neutral or slightly bullish outlook of the market wherein the potential future upside of the underlying asset owned by the investor is limited. This strategy is used by investors when they would prefer booking short-term profits on the assets than to keep holding it.

For instance, consider a ‘buy write’ situation where an investor buys shares of a stock (i.e., holds a long position in the stock) and simultaneously writes call options on them. The investor has a neutral view on the stock and doesn’t expect the price to rise much.
To book a short-term profit and also hedge any minor downsides in the stock price, the investor is writing call options on the stock at a strike price greater than or equal to the current price of the stock (i.e. out-of-the-money or at-the-money call options). The buyer of those call options would pay the investor a premium on those calls, whether or not the option is exercised. This is the covered call strategy in a nutshell.

Let us consider a covered call position with writing at-the money calls. One of following three scenarios may happen:

Scenario 1: the stock price does not change, and calls expire at the money

In this scenario, the market viewpoint of the investor holds correct and the profit from the strategy is the premium earned on the call options. In this case, the option holder does not exercise its call options, and the investor gets to keep the underlying stocks too.

Scenario 2: the stock price rises, and calls expire in the money

In this scenario, since the price of the stock was already locked in through the call, the investor enjoys a short-term profit along with the premium. However, this also poses a risk in case the price of the stock rises substantially because the investor misses out on the opportunity.

Scenario 3: the stock price falls and calls expire out of the money

This is a negative scenario for the investor. There is limited protection from the downside through the premium earned on the call options. However, if the stock price falls below a certain break-even point, the losses for the investor can be considerable since there will be a fall in its underlying position.

Risk profile

In a covered call, the total cost of the investment is equal to the price of the underlying asset minus the premium earned by writing the call. However, the profit potential for the investment is limited and the maximum loss can be significantly high. The risk profile of the position is represented in Figure 1.

Figure 1. Risk profile of covered call position.

Source: computation by the author (based on the BSM model).

You can download below the Excel file for the computation of the Profit or Loss (P&L) function of the underlying position and covered call position.

The delta of the position is equal to the sum of the delta of the long position in the underlying asset (+1) and the short position in the call option (-Δ).

Figure 2 represents the delta of the covered call position as a function of the price of the underlying asset. The delta of the call option is computed with the Black-Scholes-Merton model (BSM model).

Figure 2. Delta of a covered call position.

Source: computation by the author (based on the BSM model).

You can download below the Excel file for the computation of the delta of a protective put position.

Example

An investor holds 100 shares of Apple bought at the current price of $144 each. The total investment is then equal to $14,400. She is neutral about the short-term prospects of the market. In order to gain from her market scenario, she decides to write an at-the-money call option at $144 on the Apple stock (lot size is 100) with a maturity of one month, using the covered call strategy.

We use the following market data: the current price of Appel stock is $144, the implied volatility of Apple stock is 22.79%, and the risk-free interest rate is equal to 1.59%.

Based on the Black-Scholes-Merton model, the price of the call option is $3.87.

Let us consider three scenarios at the time of maturity of the call option:

Scenario 1: stability of the price of the underlying asset at $144

The total cost of the initial investment is the cost of acquiring the Apple stocks ($14,400) minus the premium received on writing the calls ($387 = $3.87*100), which is equal to $14,013, i.e. $14,400 – $387.

As the stock price ($144) is equal to the strike price of the call options ($144), the value of the call options is equal to zero, and the investor earns a profit which is equal to the initial price of the call options (the premium), which is equal to $387.

Scenario 2: an increase in the price of the underlying asset to $155

The total cost of the initial investment is the cost of acquiring the Apple stocks ($14,400) minus the premium on writing the calls ($387 = $3.87*100), which is equal to $14,013, i.e. $14,400 – $387.

As the stock price has risen to $155, the call options are exercised by the option buyer, and the investor will have to sell the Apple stocks at the strike price of $144.

By executing the covered call strategy, the investor earns $387 (i.e. ($144-$144)*100 +$387) but misses the opportunity of earning higher profits by selling the stock at the current market price of $155.

Scenario 3: a decrease in the price of the underlying asset to $142

As the stock price is at $142, the call options are not exercised by the option buyer and the options expire worthless (out of the money).

As the buyer does not exercise the call options, the investor earns a profit which is equal to the price of the call options which is equal to $387. But his net profit decreases by the amount of the decrease in his position in the APPLE stocks which is equal to -$200 (i.e. ($142-$144)*100).

▶ All posts about Options

▶ Akshit GUPTA Options

▶ Akshit GUPTA Option Trader – Job description

▶ Akshit GUPTA The Black-Scholes-Merton model

▶ Akshit GUPTA Protective Put

▶ Akshit GUPTA Option Greeks – Delta

Useful Resources

Research articles

Black F. and M. Scholes (1973) “The Pricing of Options and Corporate Liabilities” The Journal of Political Economy, 81, 637-654.

Merton R.C. (1973) “Theory of Rational Option Pricing” Bell Journal of Economics, 4(1): 141–183.

Books

Hull J.C. (2015) Options, Futures, and Other Derivatives, Ninth Edition, Chapter 10 – Trading strategies involving Options, 276-295.

Wilmott P. (2007) Paul Wilmott Introduces Quantitative Finance, Second Edition, Chapter 8 – The Black Scholes Formula and The Greeks, 182-184.

About the author

Article written in December 2021 by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).

The Black Scholes Merton Model

February 4, 2024August 19, 2021 by Akshit GUPTA

The Black-Scholes-Merton model

This article written by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) presents the Black-Scholes-Merton Model .

Introduction

Options are one of the most popular derivative contracts used by investors to hedge the risks of their portfolios, to optimize the risk profile of their positions and to make profits (or losses) by means of speculation. The value of options is known at maturity date (or expiration date) as it is given by their pay-off functions defined in their contracts. But what is the value of the option at the issuance date or any date between the issuance and the expiration? The Black-Scholes-Merton model allows to answer this question.

The Black-Scholes-Merton model is an continuous-time option pricing model used to determine the fair price or theoretical value for a call or a put option based on variable factors such as the maturity date and the strike price of the option (option characteristics), and the price of underlying asset, the volatility of the price of underlying asset, and the risk-free rate (market data). It is used to determine the price of a European call option, which refers to the option that can only be exercised on the maturity date.

History

The model was first introduced to the world by a paper titled ‘The Pricing of Options and Corporate Liabilities’ by Fischer Black and Myron Scholes and was officially published in spring 1973. Almost around the same time as Black and Scholes, Robert Merton, who was also a colleague of Scholes at MIT Sloan, presented his contributions to the model in another paper named ‘Theory of Rational Option Pricing’, where he coined the name “Black-Scholes model”. Later, Black and Scholes also published empirical tests of the model in their ‘The Valuation of Option Contracts and a Test of Market Efficiency’ paper. For their significant contribution to the world of financial markets, Merton and Black were awarded the prestigious Nobel Prize in Economic Sciences in 1997 (unfortunately Scholes had passed away in 1995 due to which he was ineligible for the Nobel Prize).

In the BSM model, the value of an option depends on the future volatility of the underlying stock rather than on its expected return. The pricing formula is based on the assumption that the price of the underlying asset follows a geometric Brownian motion.

Option pricing with BSM

The BSM model is used to find the theoretical value of a European option. The model assumes that the price of the underlying asset follows a geometric Brownian motion, which implies that the returns on the underlying asset are normally distributed. It is also assumed that there are no arbitrage opportunities, no transaction costs and the risk-free rate remains constant over time.

The BSM formula

The payoffs for a call option and a put option give the value of these options at the maturity date T:

For a call option:

Formula for the payoff of a call option

For a put option:

BSM Formula for the payoff of a put option

The BSM formula gives the price of European put and call options at any date before the maturity date T. The value of European call and put options for a non-dividend paying stock are given by:

For a call option:

BSM formula for the call option

For a put option:

BSM formula for the put option

where,

Formula for the D1 Formula for the D2

The notations used in the above formulae are described as :

S_t: price of the underlying asset at time t
t: current date (or date of calculation of option price)
T: maturity or expiry date of the option
K: strike price of the option
r: risk-free interest rate
σ: volatility (the standard deviation of the return on the underlying asset)
N(.): cumulative distribution function for a normal (Gaussian) distribution (0 ≤ N(.) ≤ 1 )

For a call option, N(+d₂) is the probability that the option will be exercised, and Ke^{(-r(T-t) )} N(+d₂) is what is expected to be paid for the underlying stock if the option is exercised, discounted to today (or the calculation date t).

Similarly, SN(+d₁) is what we can expect to receive from selling the underlying stock, if the option is exercised, also discounted to today (or the calculation date t).

For a put option, N(-d₂) is the probability that the option will be exercised, and Ke^{(-r(T-t) )} N(-d₁ ) is what is expected to be paid for the underlying stock if the option is exercised, discounted to today (or the calculation date t).

Similarly, SN(-d₁ ) is what we can expect to receive from selling the underlying stock, if the option is exercised, also discounted to today (or the calculation date t).

Note that the value of the option given by the BSM formula depends on the maturity date and the strike price of the option (option characteristics), and the price of underlying asset, and the risk-free rate (market data) and the volatility of the price of underlying asset. While the option characteristics are known and the market data are observable, the volatility of the price of underlying asset is the only unknown variable in the formula.

Beyond the formula itself for the option prices, the BSM model also gives a method to manage the option over time (delta hedging) as an option is equivalent (under the assumption of no arbitrage) to a portfolio composed of the underlying asset and risk-free bond.

Example – Call and Put option pricing using Black-Scholes-Merton model

Figure 1 gives the graphical representation of the value of a call option at time t as a function of the price of the underlying asset at time t as given by the BSM formula. The strike price for the call option is 40€ with a maturity of 0.50 years. The price of the underlying asset is 50€ at time t and volatility is 40%. The risk-free rate is assumed to be 1%.

Figure 1. Call option Pricing using BSM formula Covered call
Source: computation by the author (based on the BSM model).

Figure 2 gives the graphical representation of the value of a put option at time t as a function of the price of the underlying asset at time t as given by the BSM formula. The strike price for the put option is 40€ with a maturity of 0.50 years. The price of the underlying asset is 50€ at time t and volatility is 40%. The risk-free rate is assumed to be 1%.

Figure 2. Put option Pricing using BSM formula Covered call
Source: computation by the author (based on the BSM model).

You can download below the Excel file used for the computation of the Call and Put option prices using the BSM Model.

Conclusion

The option-pricing model developed by Black, Scholes and Merton in 1973 provides a way of computing the prices of option contracts and has been widely used by traders since its publication. Following the seminal works by Black, Scholes and Merton, there haven been many extensions of their model, which have broadened its applicability to other instruments such as more complex options and insurance contracts.

Limitations of the BSM model

However, the model is sometimes criticized due to its weaknesses emerging from unrealistic sets of assumptions, which cause errors in estimation and model’s predictions. For instance, the BSM model assumes a constant value for volatility of the price of the underlying asset and also neglects any dividend payments from stocks which is certainly not the case in real life. Also, the model is only applicable to European options and would not be able to accurately determine the value of an American option which can be exercised at any time until the expiry date. Researchers have worked on amending the model to incorporate more realistic assumptions and have concluded that despite the model’s weaknesses, its application is still extremely useful in analyzing option prices.

Useful resources

Academic research

Black F. and M. Scholes (1973) “The Pricing of Options and Corporate Liabilities” The Journal of Political Economy, 81, 637-654.

Hull J.C. (2015) Options, Futures, and Other Derivatives, Ninth Edition, Chapter 15 – The Black-Scholes-Merton model, 343-375.

Merton R.C. (1973) “Theory of Rational Option Pricing” Bell Journal of Economics, 4, 141–183.

Wilmott P. (2007) Paul Wilmott Introduces Quantitative Finance, Second Edition, Chapter 8 – The Black Scholes Formula and The Greeks, 182-184.

About the author

Article written in August 2021 by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).

Option Greeks – Theta

February 4, 2024August 19, 2021 by Akshit GUPTA

Option Greeks – Theta

This article written by Akshit GUPTA (ESSEC Business School, Master in Management, 2019-2022) presents the technical subject of theta, an option Greek used in option pricing and hedging to deal with he passing of time.

Introduction

Theta is a type of option Greek which is used to compute the sensitivity or rate of change of the value of an option contract with respect to its time to maturity. The theta is denoted using the symbol (θ). Essentially, the theta is the first partial derivative of the price of the option contract with respect to the time to maturity of the option contract.

It is shown as:

Formula for the theta

Where V is the value of the option contract and T the time to maturity for the option contract.

Theoretically, as the option contract approaches maturity, the theta of on option contract increases and moves towards zero as the time value or the time value of the option decreases. This is referred to as “theta decay”.

For example, an option contract is trading at a premium of $10 and has a theta of -0.8. Thus, with theta decay, the option price will decrease to $9.2 after one day and further to $6 after five days.

The figure below represent the theta of a call option as a function of the time to maturity:

Figure 1. Theta of a call option as a function of time to maturity.

Source: computation by the author (Model: Black-Scholes-Merton).

Intrinsic and time value of an option contract

Essentially, the price of an option contract consists of two values namely, the intrinsic value and the time value (sometimes called extrinsic value). The intrinsic value in the price of an option contract is the real value or the fundamental value of an option based on the price of the underlying asset at a given point in time.

For example, a call option contract has a strike price of $10 and the underlying asset has a market price of $17. Theoretically, the buyer of a call option can execute the contract and buy the asset at $10 and sell it in the market for $17. He/she can make an immediate profit of $7 if they decide to exercise the option. Thus, the intrinsic value of the option contract is $7.

If the current call option price/premium is $9 in the market and the intrinsic value is $7, then the time value can be calculated as:

Time Value for the theta

Thus, the time value is $9-$7 is equal to $2. The $2 is the time value of an option contract which is determined by the factors other than the price of the underlying asset. As the option approaches maturity, the time value of the option contract declines and tends to zero. The price of an option contract which is at the money or out the money, it consists entirely of the time value as there is no intrinsic value involved.

For example, a call option contract with a strike price of $20, the underlying asset price of $15, and option premium of $3, has a time value equal to the option premium, $3, since the option is out of money.

Calculating Theta for call and put options

The theta for a non-dividend paying stock in a European call and put option is calculated using the following formula from the Black-Scholes Merton model:

Formula for the theta of a call and a put option

Where N’(d₁) represents the first order derivative of the cumulative distribution function of the normal distribution given by:

First_ derivative_Normal_distribution_d1

d₁ is given by:

Formula for d1

d₂ is given by:

Formula for d2

And N(-d₂) is given by:

Formula for -d2

Where S is the price of the underlying asset (at the time of valuation of the option), σ the volatility in the price of the underlying asset, T time to option’s maturity, K the strike price of the option contract and r the risk-free rate of return.

Excel pricer to calculate the theta of an option

You can download below an Excel file for an option pricer (based on the Black-Scholes-Merton or BSM model) which allows you to calculate the theta of a European-style call option.

Example for calculating theta

Let us consider a call option contract with the following characteristics: the underlying asset is an Apple stock, the option strike price (K) is equal to $300 and the time to maturity (T) is of one month (i.e., 0.084 years).

At the time of valuation, the price of the Apple stock (S) is $300, the volatility (σ) of Apple stock is 30% and the risk-free rate (r) is 3% (market data).

The theta of a call option is approximately equal to -0.2636 per trading day.

Using the above example, we can say that after one trading day, the price of the option will decrease by $0.2636 (approximately) due to time decay.

Useful resources

Hull J.C. (2015) Options, Futures, and Other Derivatives, Ninth Edition, Chapter 19 – The Greek Letters, 424–431.

Wilmott P. (2007) Paul Wilmott Introduces Quantitative Finance, Second Edition, Chapter 8 – The Black Scholes Formula and The Greeks, 182-184.

About the author

Article written in August 2021 by Akshit GUPTA (ESSEC Business School, Master in Management, 2019-2022).

Option Greeks – Vega

January 30, 2024August 19, 2021 by Akshit GUPTA

Option Greeks – Vega

This article written by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) explains the technical subject of vega, the option Greek used in option pricing and hedging to take into account the volatility of the underlying asset.

Introduction

Vega is a type of option Greek which is used to compute the sensitivity or rate of change of the value of an option contract with respect to the volatility of the underlying asset. The Vega is denoted using the Greek letter (ν). Essentially, the vega is the first partial derivative of the value of the option contract with respect to the volatility of the underlying asset.

The vega formula for an option is given by

Formula for the gamma

Where V is the value of the option contract and σ is the volatility of the underlying asset.

If the Vega is a very high positive or a negative number, this means that the option price is highly sensitive to the volatility of the underlying asset. The Vega is maximum when the option price is at the money. For example, the strike of an option contract is €100, and the price of the underlying asset is €100. The option is at the money (ATM) and has an intrinsic value of zero. So, the option premium entirely consists of the time value of the option. Thus, the Vega is the highest for at the money option contract since the option value are mostly dependent on the time value (sometimes called the extrinsic value). An increase/decrease in volatility can change the option value significantly for at-the-money options.

Figure 1 below represents the vega of a call option as a function of the price of the underlying asset. The parameters of the call option are a maturity of 3 months and a strike of €100. The market data are a price of the underlying asset between €50 and €150, a volatility of the underlying asset of 40%, a risk-free interest rate of 3% and a dividend yield of 0%.

Figure 1. Vega of a call option as a function of the price of the underlying asset.

Source: computation by the author (Model: Black-Scholes-Merton).

Calculating the vega for call and put options

The vega for a European call or put option is calculated using the following formula:

Formula for the gamma

where

N’(d₁) represents the first order derivative of the cumulative distribution function of the normal distribution given by:

First_ derivative_Normal_distribution_d1

and d₁ is given by:

Formula for d1

where S is the price of the underlying asset (at the time of valuation of the option), σ the volatility in the price of the underlying asset, T time to option’s maturity, K the strike price of the option contract and r the risk-free rate of return.

Example for calculating vega

At the time of valuation, the price of the Apple stock (S) is $300, the volatility (σ) of Apple stock is 30% and the risk-free rate (r) is 3% (market data).

The vega of the call option is approximately equal to 0.3447963.

Using the above value, we can say that due to a 1% change in the volatility of the underlying asset, the price of the option will change approximately by $0.3447.

Excel pricer to calculate the vega of an option

You can download below an Excel pricer (based on the Black-Scholes-Merton or BSM model) to calculate the vega of an option (call or put).

Useful resources

Hull J.C. (2015) Options, Futures, and Other Derivatives, Ninth Edition, Chapter 19 – The Greek Letters, 424–431.

Wilmott P. (2007) Paul Wilmott Introduces Quantitative Finance, Second Edition, Chapter 8 – The Black Scholes Formula and The Greeks, 182-184.

About the author

Article written in August 2021 by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).

Option Greeks – Gamma

February 4, 2024August 8, 2021 by Akshit GUPTA

Option Greeks – Gamma

This article written by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) presents the technical subject of gamma, an option Greek used in option hedging.

Introduction

Gamma is a type of option Greek which is used to compute the sensitivity or rate of change of delta (Δ) of an option contract with respect to a change in the price of the underlying in the option contract (S). The gamma of an option is expressed in percentage terms. Denoted by the Greek letter (Γ), the gamma is defined by

Formula for the gamma of an option

Where (Δ) is the delta of the option and S the price of the underlying asset.

Essentially, the gamma is the second partial derivative of the value of the option contract (V) with respect to the price of the underlying asset (S). It measures the convexity of the value of the option contract with respect to the price of the underlying asset. The gamma then corresponds to

Formula for the gamma of an option

Where V is the value of the option and S the price of the underlying asset.

The gamma of an option contract is at its maximum when the price of the underlying asset is equal to the strike price of the option (an at-the-money option). If the price of the underlying moves deeper in the money or out of the money, the value of the gamma approaches zero.

The gamma as a function of the price of the underlying asset for a call option is given below.

Figure 1. Gamma of a call option.

Source: computation by the author (Model: Black-Scholes-Merton).

Also, if the gamma of the option contract is small, it means that the delta of the option moves slowly with the price of the underlying asset.

Calculating gamma for call and put options

The gamma for European call or put options on a non-dividend paying stock is calculated using the following formula from the Black-Scholes-Merton model is:

Formula for the gamma of a call/put option

Where,N’d₁ represents the first order derivative of the cumulative distribution function of the normal distribution given by:

First_ derivative_Normal_distribution_d1

and d₁ is given by:

Formula for d1.png

Excel pricer to calculate the gamma of an option

You can download below an Excel file for an option pricer (based on the Black-Scholes-Merton or BSM model) which allows you to calculate the gamma of a European-style call option.

Delta-gamma hedging

A trader holding a portfolio of option contracts uses gamma hedging to offset the risks associated with the price movement in the underlying asset by buying and selling the option contracts to maintain a constant delta. Generally, the delta is maintained near or at the zero level to attain delta neutrality. The neutrality in the gamma for the option is required to protect the portfolio’s value against sharp price movements in the price of the underlying asset.

Formula for the gamma hedging of a call option

Limitations of gamma hedging

The limitation of gamma hedging includes the following:

Transaction cost – Gamma hedging requires constantly monitoring the markets and buying or selling the option contracts. Due to this practice of buying and selling frequently, the transaction costs are quite high to execute a gamma hedge. Thus, gamma hedging is an expensive strategy to practice.
Loosing delta neutrality – Whenever a trader executes a gamma hedge and trades in option contracts, it is often accompanied with a move in the portfolio’s delta. Thus, to achieve delta neutrality again, the trader must buy or sell additional quantities of the underlying asset, which is time consuming and comes with a transaction cost.

Useful resources

Hull J.C. (2015) Options, Futures, and Other Derivatives, Ninth Edition, Chapter 19 – The Greek Letters, 424–431.

Wilmott P. (2007) Paul Wilmott Introduces Quantitative Finance, Second Edition, Chapter 8 – The Black Scholes Formula and The Greeks, 182-184.

About the author

Article written in August 2021 by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).

Option Greeks – Delta

February 4, 2024August 5, 2021 by Akshit GUPTA

Option Greeks – Delta

This article written by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) presents the technical subject of delta, an option Greek used in option pricing and hedging.

Introduction

Option Greeks are sophisticated financial metric used by trader to calculate the sensitivity of option contracts to different factors related to the underlying asset including the price of the underlying, its volatility, and time value. The Greeks are used as an effective tool to practice different hedging strategies and eliminate risks in a position. They also help to optimize the options positions at any point in time.

Delta is a type of option Greek which is used to compute the sensitivity or rate of change in price of the option contract with respect to the change in price of the underlying asset. It is denoted by the Greek letter (Δ). The formula for calculating the delta of an option contract is:

Formula for the delta of an option

Where V is the value of the option and S the price of the underlying asset.

For example, if an option on Apple stock has a delta of 0.3, it essentially means that a $1 change in the price of the underlying asset i.e., Apple stock, will lead to a change of $0.3 in the price of the option contract.

When a trader takes a position based on the delta sensitivity of any option contract, it is called delta hedging. The goal is to achieve a delta-neutral portfolio and eliminate the risks associated with movement in the prices of the underlying. Due to the complexity of the tool, delta hedging is generally practiced by professional traders in large financial institutions. In options, the delta of any call option is always positive whereas the delta of a put option is always negative.

Delta formula

Call option

According the Black-Scholes-Merton model, the formula for calculating the delta for a European-style call option on a non-dividend paying stock is given by:

Formula for the delta of a call option

Where N represents the cumulative distribution function of the normal distribution and d₁ is given by:

Formula for d1

Where S is the price of the underlying asset (at the time of valuation of the option), σ the volatility in the price of the underlying asset, T time to maturity of the option, K the strike price of the option, and r the risk-free rate of return.

Put option

According the Black-Scholes-Merton model, the formula for calculating the delta for a European-style put option on a non-dividend paying stock is given by:

Formula for the delta of a put option

Delta as a function of the price of the underlying asset

Call option

The delta as a function of the price of the underlying asset for a European-style call option is represented in Figure 1.

Figure 1. Delta of a call option.

Source: computation by the author (Model: Black-Scholes-Merton).

For a call option, the delta increases from 0 (out-of-the-money option) to 1 (in-the-money option).

Put option

The delta as a function of the price of the underlying asset for a European-style put option is represented in Figure 2.

Figure 2. Delta of a put option.

Source: computation by the author (Model: Black-Scholes-Merton).

For a put option, the delta increases from -1 (in-the-money option) to 0 (out-of-the-money option).

Excel pricer to calculate the delta of an option

You can download below an Excel file for an option pricer (based on the Black-Scholes-Merton or BSM model) which allows you to calculate the delta of a European-style call option.

Delta Hedging

A trader holding an option contract uses delta hedging to offset the risks associated with the price movement in the underlying asset by continuously buying and selling the underlying asset to achieve delta neutrality. This is used by option traders in financial institutions to manage their option book (the delta is computed at the option level and aggregated at the book level) and generate the margin the bank of the option writing activity.

The delta of an option contract keeps on changing as the prices of the underlying and the option contract changes. So, to maintain the delta neutrality the trader must constantly monitor the markets and execute trades to achieve neutrality. The process of continuously buying or selling the underlying asset is called dynamic hedging in options.

At the first order, the change of the value of a delta-hedged call option over the period from t to t+ δt would be equal to the risk-free rate (r) over the period:

Formula for the delta hedging of a call option

Limitations of delta hedging

Although delta hedging is a useful tool to offset the risks associated to the movement in the price of an underlying, it comes with some limitations which are:

Transaction cost

Since delta hedging requires constantly buying or selling the underlying asset, it comes with a high transaction cost. This makes delta hedging an expensive tool to optimize the portfolio against price risk. In practice, traders would adjust their option position from time top time.

Illiquid Markets

When the market for an asset is illiquid, it is difficult to practice delta hedging as the trader will not be able to constantly buy or sell the underlying asset to neutralize the price impact.

Example for calculating delta

At the time of valuation, the price of the Apple stock (S) is $300, the volatility (σ) of Apple stock is 30% and the risk-free rate (r) is 3% (market data).

The delta of a call option is approximately equal to 0.50238.

Using the above value, we can say that due to a $1 change in the price of the underlying asset, the price of the option will change by $0.50238.

Useful resources

Research articles

Black F. and M. Scholes (1973) The Pricing of Options and Corporate Liabilities The Journal of Political Economy, 81, 637-654.

Merton R.C. (1973) Theory of Rational Option Pricing Bell Journal of Economics, 4(1): 141–183.

Books

Hull J.C. (2015) Options, Futures, and Other Derivatives, Ninth Edition, Chapter 19 – The Greek Letters, 424 – 431.

Wilmott P. (2007) Paul Wilmott Introduces Quantitative Finance, Second Edition, Chapter 8 – The Black Scholes Formula and The Greeks, 182-184.

About the author

Article written in August 2021 by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).

Assumptions of the BSM Model

The BSM equation

The BSM formulae

Some Criticisms and Limitations

American options

Stocks paying dividends

Constant volatility

Related posts on the SimTrade blog

Useful resources

About the author

Straddle and Strangle

Introduction

Straddle

Market Scenario

Example

Strangle

Market Scenario

Example

Related Posts

Useful resources

Academic research articles

Books

About the author

Option Spreads

Introduction

Bull Spread

Market Scenario

Example

Bear Spread

Market Scenario

Example

Butterfly Spread

Market Scenario

Example

Related posts

Useful resources

About the author

Covered Call

Introduction

Covered call

Market scenario

Scenario 1: the stock price does not change, and calls expire at the money

Scenario 2: the stock price rises, and calls expire in the money

Scenario 3: the stock price falls and calls expire out of the money

Risk profile

Example

Scenario 1: stability of the price of the underlying asset at $144

Scenario 2: an increase in the price of the underlying asset to $155

Scenario 3: a decrease in the price of the underlying asset to $142

Related Posts

Useful Resources

Research articles

Books

About the author

The Black-Scholes-Merton model

Introduction

History

Option pricing with BSM

The BSM formula

Example – Call and Put option pricing using Black-Scholes-Merton model

Conclusion

Limitations of the BSM model

Related posts on the SimTrade blog

Useful resources

Academic research

About the author

Option Greeks – Theta

Introduction

Intrinsic and time value of an option contract

Calculating Theta for call and put options

Excel pricer to calculate the theta of an option

Example for calculating theta

Related Posts on the SimTrade blog

Useful resources

About the author

Option Greeks – Vega

Introduction

Calculating the vega for call and put options

Example for calculating vega

Excel pricer to calculate the vega of an option