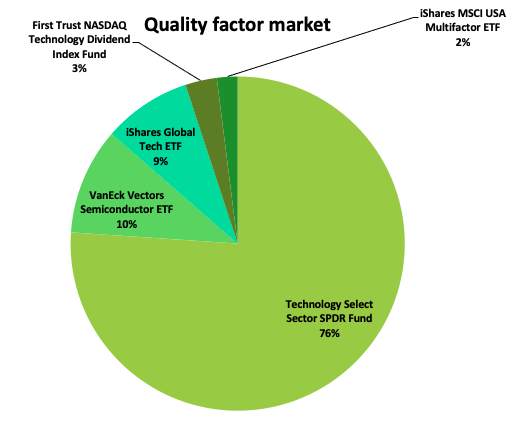
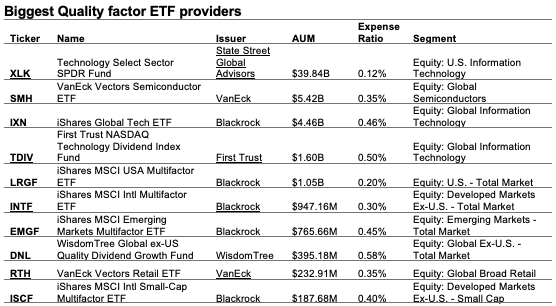
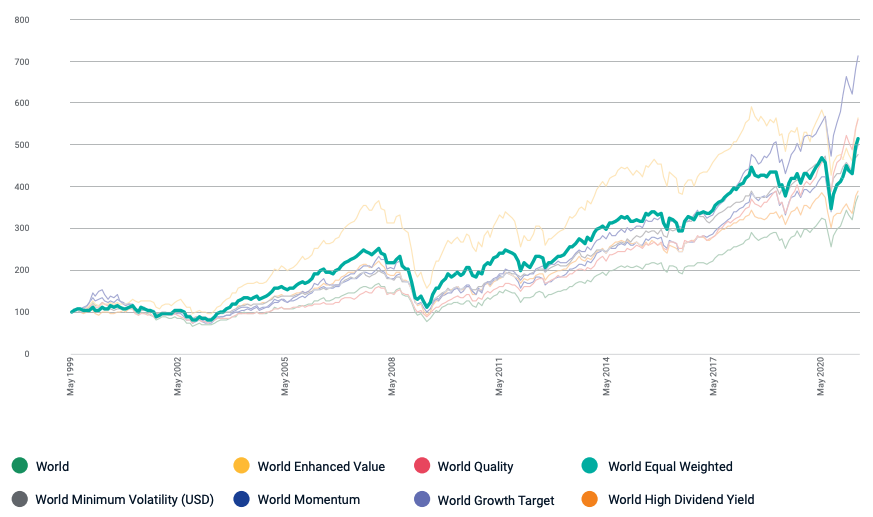
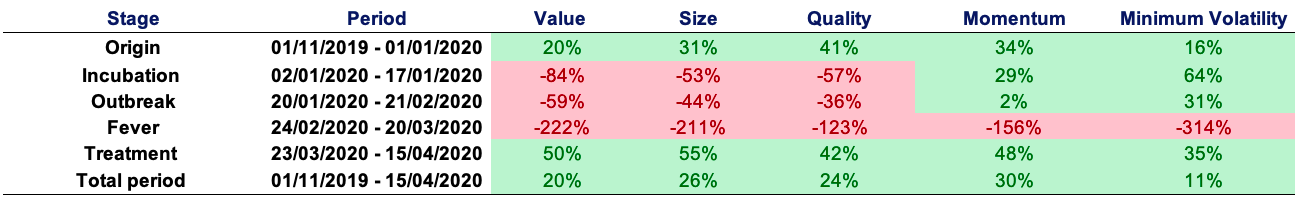
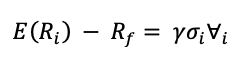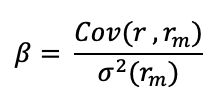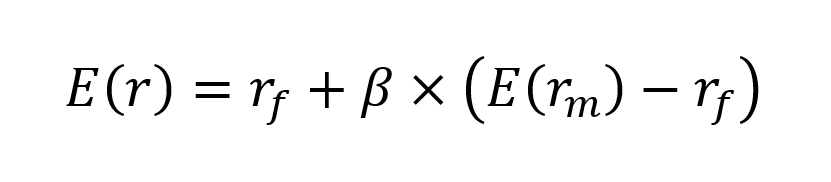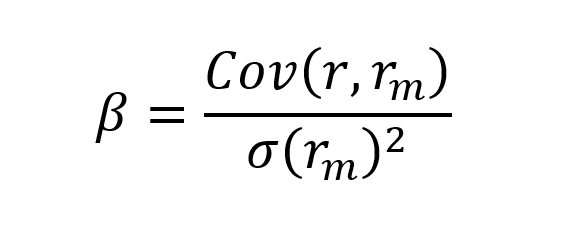Arbitrage Pricing Theory (APT)

April 18, 2025January 2, 2023 by Youssef LOURAOUI

Arbitrage Pricing Theory (APT)

In this article, Youssef LOURAOUI (Bayes Business School, MSc. Energy, Trade & Finance, 2021-2022) presents the concept of arbitrage portfolio, a pillar concept in asset pricing theory.

This article is structured as follows: we present an introduction for the notion of arbitrage portfolio in the context of asset pricing, we present the assumptions and the mathematical foundation of the model and we then illustrate a practical example to complement this post.

Introduction

Arbitrage pricing theory (APT) is a method of explaining asset or portfolio returns that differs from the capital asset pricing model (CAPM). It was created in the 1970s by economist Stephen Ross. Because of its simpler assumptions, arbitrage pricing theory has risen in favor over the years. However, arbitrage pricing theory is far more difficult to apply in practice since it requires a large amount of data and complicated statistical analysis.The following points should be kept in mind when understanding this model:

Arbitrage is the technique of buying and selling the same item at two different prices at the same time for a risk-free profit.
Arbitrage pricing theory (APT) in financial economics assumes that market inefficiencies emerge from time to time but are prevented from occurring by the efforts of arbitrageurs who discover and instantly remove such opportunities as they appear.
APT is formalized through the use of a multi-factor formula that relates the linear relationship between the expected return on an asset and numerous macroeconomic variables.

The concept that mispriced assets can generate short-term, risk-free profit opportunities is inherent in the arbitrage pricing theory. APT varies from the more traditional CAPM in that it employs only one factor. The APT, like the CAPM, assumes that a factor model can accurately characterize the relationship between risk and return.

Assumptions of the APT model

Arbitrage pricing theory, unlike the capital asset pricing model, does not require that investors have efficient portfolios. However, the theory is guided by three underlying assumptions:

Systematic factors explain asset returns.
Diversification allows investors to create a portfolio of assets that eliminates specific risk.
There are no arbitrage opportunities among well-diversified investments. If arbitrage opportunities exist, they will be taken advantage of by investors.

To have a better grasp on the asset pricing theory behind this model, we can recall in the following part the foundation of the CAPM as a complementary explanation for this article.

Capital Asset Pricing Model (CAPM)

William Sharpe, John Lintner, and Jan Mossin separately developed a key capital market theory based on Markowitz’s work: the Capital Asset Pricing Model (CAPM). The CAPM was a huge evolutionary step forward in capital market equilibrium theory, since it enabled investors to appropriately value assets in terms of systematic risk, defined as the market risk which cannot be neutralized by the effect of diversification. In their derivation of the CAPM, Sharpe, Mossin and Litner made significant contributions to the concepts of the Efficient Frontier and Capital Market Line. The seminal contributions of Sharpe, Litner and Mossin would later earn them the Nobel Prize in Economics in 1990.

The CAPM is based on a set of market structure and investor hypotheses:

There are no intermediaries
There are no limits (short selling is possible)
Supply and demand are in balance
There are no transaction costs
An investor’s portfolio value is maximized by maximizing the mean associated with projected returns while reducing risk variance
Investors have simultaneous access to information in order to implement their investment plans
Investors are seen as “rational” and “risk averse”.

Under this framework, the expected return of a given asset is related to its risk measured by the beta and the market risk premium:

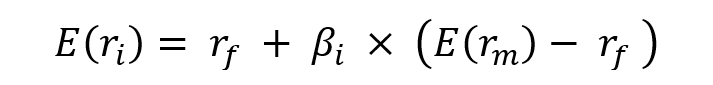
CAPM risk beta relation

Where :

E(r_i) represents the expected return of asset i
r_f the risk-free rate
β_i the measure of the risk of asset i
E(r_m) the expected return of the market
E(r_m)- r_f the market risk premium.

In this model, the beta (β) parameter is a key parameter and is defined as:

CAPM beta formula

Where:

Cov(r_i, r_m) represents the covariance of the return of asset i with the return of the market
σ²(r_m) is the variance of the return of the market.

The beta is a measure of how sensitive an asset is to market swings. This risk indicator aids investors in predicting the fluctuations of their asset in relation to the wider market. It compares the volatility of an asset to the systematic risk that exists in the market. The beta is a statistical term that denotes the slope of a line formed by a regression of data points comparing stock returns to market returns. It aids investors in understanding how the asset moves in relation to the market. According to Fama and French (2004), there are two ways to interpret the beta employed in the CAPM:

According to the CAPM formula, beta may be thought in mathematical terms as the slope of the regression between the asset return and the market return. Thus, beta quantifies the asset sensitivity to changes in the market return.
According to the beta formula, it may be understood as the risk that each dollar invested in an asset adds to the market portfolio. This is an economic explanation based on the observation that the market portfolio’s risk (measured by σ²(r_m)) is a weighted average of the covariance risks associated with the assets in the market portfolio, making beta a measure of the covariance risk associated with an asset in comparison to the variance of the market return.

Mathematical foundations

The APT can be described formally by the following equation

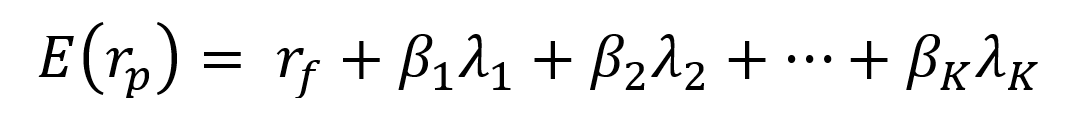
APT expected return formula

Where

E(r_p) represents the expected return of portfolio p
r_f the risk-free rate
β_k the sensitivity of the return on portfolio p to the k^th factor (f_k)
λ_k the risk premium for the k^th factor (f_k)
K the number of risk factors

Richard Roll and Stephen Ross found out that the APT can be sensible to the following factors:

Expectations on inflation
Industrial production (GDP)
Risk premiums
Term structure of interest rates

Furthermore, the researchers claim that an asset will have varied sensitivity to the elements indicated above, even if it has the same market factor as described by the CAPM.

Application

For this specific example, we want to understand the asset price behavior of two equity indexes (Nasdaq for the US and Nikkei for Japan) and assess their sensitivity to different macroeconomic factors. We extract a time series for Nasdaq equity index prices, Nikkei equity index prices, USD/CHY FX spot rate and US term structure of interest rate (10y-2y yield spread) from the FRED Economics website, a reliable source for macroeconomic data for the last two decades.

The first factor, which is the USD/CHY (US Dollar/Chinese Renminbi Yuan) exchange rate, is retained as the primary factor to explain portfolio return. Given China’s position as a major economic player and one of the most important markets for the US and Japanese corporations, analyzing the sensitivity of US and Japanese equity returns to changes in the USD/CHY Fx spot rate can help in understanding the market dynamics underlying the US and Japanese equity performance. For instance, Texas Instrument, which operates in the sector of electronics and semiconductors, and Nike both have significant ties to the Chinese market, with an overall exposure representing approximately 55% and 18%, respectively (Barrons, 2022). In the example of Japan, in 2017 the Japanese government invested 117 billion dollars in direct investment in northern China, one of the largest foreign investments in China. Similarly, large Japanese listed businesses get approximately 18% of their international revenues from the Chinese market (The Economist, 2019).

The second factor, which is the 10y-2y yield spread, is linked to the shape of the yield curve. A yield curve that is inverted indicates that long-term interest rates are lower than short-term interest rates. The yield on an inverted yield curve decreases as the maturity date approaches. The inverted yield curve, also known as a negative yield curve, has historically been a reliable indicator of a recession. Analysts frequently condense yield curve signals to the difference between two maturities. According to the paper of Yu et al. (2017), there is a significant link between the effects of varying degrees of yield slope with the performance of US equities between 2006 and 2012. Regardless of market capitalization, the impact of the higher yield slope on stock prices was positive.

The APT applied to this example can be described formally by the following equation:

APT expected return formula example

Where

E(r_p) represents the expected return of portfolio p
r_f the risk-free rate
β_{p, Chinese FX} the sensitivity of the return on portfolio p to the USD/CHY FX spot rate
β_{p, US spread} the sensitivity of the return on portfolio p to the US term structure
λ_{Chinese FX} the risk premium for the FX risk factor
λ_{US spread} the risk premium for the interest rate risk factor

We run a first regression of the Nikkei Japanese equity index returns onto the macroeconomic variables retained in this analysis. We can highlight the following: Both factors are not statistically significant at a 10% significance level, indicating that the factors have poor predictive power in explaining Nikkei 225 returns over the last two decades. The model has a low R2, equivalent to 0.48%, which indicates that only 0.48% of the behavior of Nikkei performance can be attributed to the change in USD/CHY FX spot rate and US term structure of the yield curve (Table 1).

Table 1. Nikkei 225 equity index regression output.
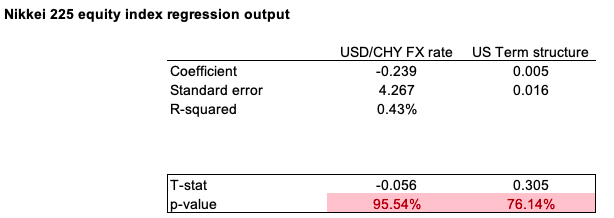
Time-series regression
Source: computation by the author (Data: FRED Economics)

Figure 1 and 2 captures the linear relationship between the USD/CHY FX spot rate and the US term structure with respect to the Nikkei equity index.

Figure 1. Relationship between the USD/CHY FX spot rate with respect to the Nikkei 225 equity index.
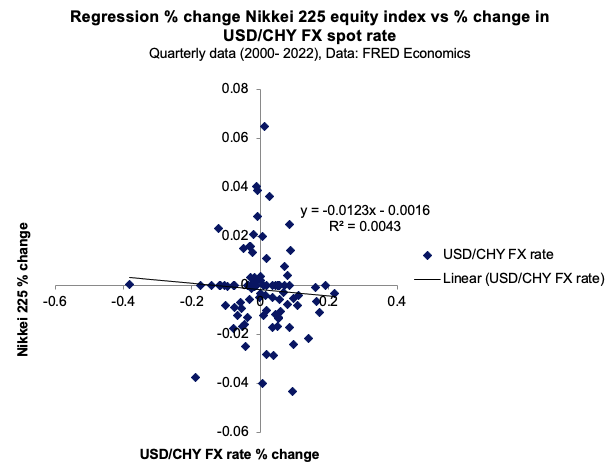
Time-series regression
Source: computation by the author (Data: FRED Economics)

Figure 2. Relationship between the US term structure with respect to the Nikkei 225 equity index.
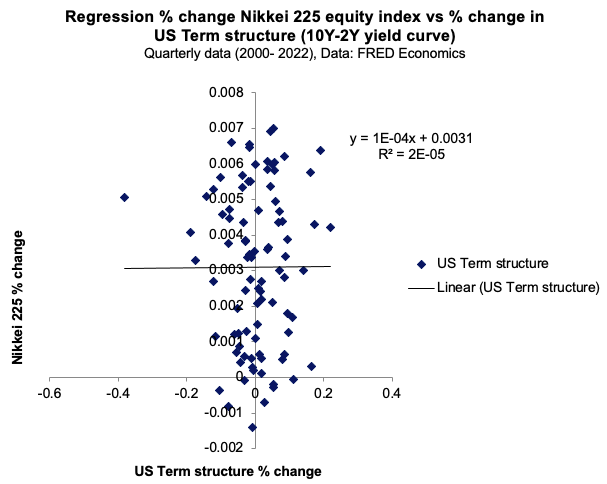
Time-series regression
Source: computation by the author (Data: FRED Economics)

We conduct a second regression of the Nasdaq US equity index returns on the retained macroeconomic variables. We may emphasize the following: Both factors are not statistically significant at a 10% significance level, indicating that they have a limited ability to predict Nasdaq returns during the past two decades. The model has a low R2 of 4.45%, indicating that only 4.45% of the performance of the Nasdaq can be attributable to the change in the USD/CHY FX spot rate and the US term structure of the yield curve (Table 2).

Table 2. Nasdaq equity index regression output.
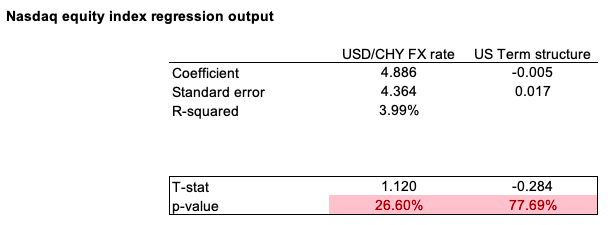
Time-series regression
Source: computation by the author (Data: FRED Economics)

Figure 3 and 4 captures the linear relationship between the USD/CHY FX spot rate and the US term structure with respect to the Nasdaq equity index.

Figure 3. Relationship between the USD/CHY FX spot rate with respect to the Nasdaq equity index.
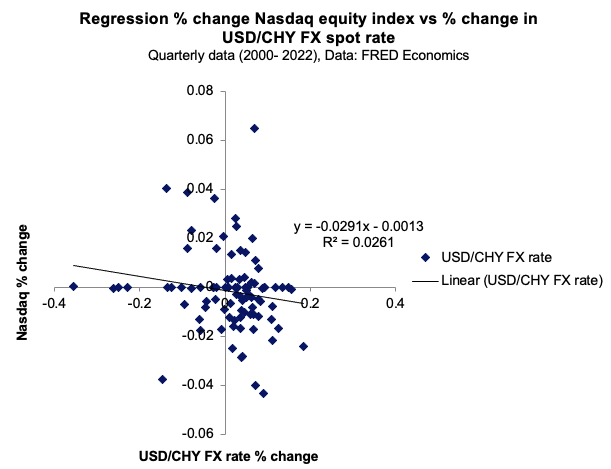
Time-series regression
Source: computation by the author (Data: FRED Economics)

Figure 4. Relationship between the US term structure with respect to the Nasdaq equity index.
Time-series regression
Source: computation by the author (Data: FRED Economics)

Applying APT

We can create a portfolio with similar factor sensitivities as the Arbitrage Portfolio by combining the first two index portfolios (with a Nasdaq Index weight of 40% and a Nikkei Index weight of 60%). This is referred to as the Constructed Index Portfolio. The Arbitrage portfolio will have a full weighting on US equity index (100% Nasdaq equity index). The Constructed Index Portfolio has the same systematic factor betas as the Arbitrage Portfolio, but has a higher expected return (Table 3).

Table 3. Index, constructed and Arbitrage portfolio return and sensitivity table.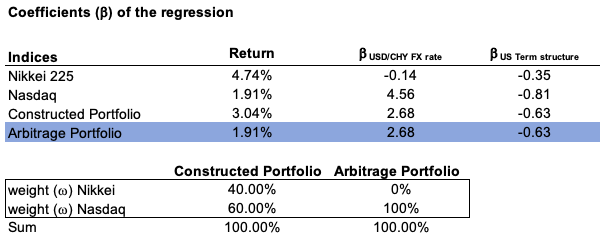 img_SimTrade_portfolio_sensitivity
Source: computation by the author (Data: FRED Economics)

As a result, the Arbitrage portfolio is overvalued. We will then buy shares of the Constructed Index Portfolio and use the profits to sell shares of the Arbitrage Portfolio. Because every investor would sell an overvalued portfolio and purchase an undervalued portfolio, any arbitrage profit would be wiped out.

Excel file for the APT application

You can find below the Excel spreadsheet that complements the example above.

Why should I be interested in this post?

In the CAPM, the factor is the market factor representing the global uncertainty of the market. In the late 1970s, the portfolio management industry aimed to capture the market portfolio return, but as financial research advanced and certain significant contributions were made, this gave rise to other factor characteristics to capture some additional performance. Analyzing the historical contributions that underpins factor investing is fundamental in order to have a better understanding of the subject.

Useful resources

Academic research

Lintner, J. (1965) The Valuation of Risk Assets and the Selection of Risky Investments in Stock Portfolios and Capital Budgets. The Review of Economics and Statistics 47(1): 13-37.

Lintner, J. (1965) Security Prices, Risk and Maximal Gains from Diversification. The Journal of Finance 20(4): 587-615.

Roll, Richard & Ross, Stephen. (1995). The Arbitrage Pricing Theory Approach to Strategic Portfolio Planning. Financial Analysts Journal 51, 122-131.

Ross, S. (1976) The arbitrage theory of capital asset pricing Journal of Economic Theory 13(3), 341-360.

Sharpe, W.F. (1963) A Simplified Model for Portfolio Analysis. Management Science 9(2): 277-293.

Sharpe, W.F. (1964) Capital Asset Prices: A theory of Market Equilibrium under Conditions of Risk. The Journal of Finance 19(3): 425-442.

Yu, G., P. Fuller, D. Didia (2013) The Impact of Yield Slope on Stock Performance Southwestern Economic Review 40(1): 1-10.

Business Analysis

Barrons (2022) Apple, Nike, and 6 Other Companies With Big Exposure to China.

The Economist (2019) Japan Inc has thrived in China of late.

Time series

FRED Economics (2022) Chinese Yuan Renminbi to U.S. Dollar Spot Exchange Rate (DEXCHUS).

FRED Economics (2022) 10-Year Treasury Constant Maturity Minus 2-Year Treasury Constant Maturity (T10Y2Y).

FRED Economics (2022) NASDAQ Composite Index (NASDAQCOM).

FRED Economics (2022) Nikkei Stock Average, Nikkei 225 (NIKKEI225).

About the author

The article was written in January 2023 by Youssef LOURAOUI (Bayes Business School, MSc. Energy, Trade & Finance, 2021-2022).

Black-Litterman Model

November 21, 2021 by Youssef LOURAOUI

In this article, Youssef LOURAOUI (Bayes Business School, MSc. Energy, Trade & Finance, 2021-2022) presents the Black-Litterman model, used to determine optimal asset allocation in a portfolio. The Black-Litterman model takes the Markowitz model one step further: it incorporates an investor’s own views in determining asset allocations.

This article is structured as follows: we introduce the Black-Litterman model. We then present the mathematical foundations of the model to understand how the method is derived. We finish with an example to illustrate how we can implement a Black-Litterman asset allocation in practice.

Introduction

The Black-Litterman asset allocation model, developed by Fischer Black and Robert Litterman in the early 1990’s, is a complex method for dealing with unintuitive, highly concentrated, input-sensitive portfolios produced by the Markowitz model. The most likely reason why more portfolio managers do not employ the Markowitz paradigm, in which return is maximized for a given level of risk, is input sensitivity, which is a well-documented problem with mean-variance optimization.

The Black-Litterman model employs a Bayesian technique to integrate an investor’s subjective views on expected returns for one or more assets with the market equilibrium expected returns (prior distribution) of expected returns to get a new, mixed estimate of expected returns. The new vector of expected returns (the posterior distribution) is a complex, weighted average of the investor’s views and the market equilibrium.

The purpose of the Black-Litterman model is to develop stable, mean-variance efficient portfolios based on an investor’s unique insights that overcome the problem of input sensitivity. According to Lee (2000), the Black-Litterman Model “essentially mitigates” the problem of estimating error maximization (Michaud, 1989) by dispersing errors throughout the vector of expected returns.

The vector of expected returns is the most crucial input in mean-variance optimization; yet, Best and Grauer (1991) demonstrate that this input can be very sensitive in the final result. Black and Litterman (1992) and He and Litterman (1999) investigate various potential projections of expected returns in their search for a fair starting point: historical returns, equal “mean” returns for all assets, and risk-adjusted equal mean returns. They demonstrate that these alternate forecasts result in extreme portfolios, which have significant long and short positions concentrated in a small number of assets.

Mathematical foundation of Black-Litterman model

It is important to introduce the Black-Litterman formula and provide a brief description of each of its elements. In the formula below, the integer k is used to represent the number of views and the integer n to express the number of assets in the investment set (NB: the superscript ’ indicates the transpose and -1 indicates the inverse).

Where:

E[R] = New (posterior) vector of combined expected return (n x 1 column vector)
τ = Scalar
Σ = Covariance matrix of returns (n x n matrix)
P = Identifies the assets involved in the views (k x n matrix or 1 x n row vector in the special case of 1 view)
Ω = Diagonal covariance matrix of error terms in expressed views representing the level of confidence in each view (k x k matrix)
П = Vector of implied equilibrium expected returns (n x 1 column vector)
Q = Vector of views (k x 1 column vector)

Traditionally, personal views are used for prior distribution. Then observed data is used to generate a posterior distribution. The Black-Litterman Model assumes implied returns as the prior distribution and personal views alter it. The basic procedure to find the Black-Litterman model is: 1) Find implied returns 2) Formulate investor views 3) Determine what the expected returns are 4) Find the asset allocation for the optimal portfolio.

Black-Litterman asset allocation in practice

An investment manager’s views for the expected return of some of the assets in a portfolio are frequently different from the the Implied Equilibrium Return Vector (Π), which represents the market-neutral starting point for the Black-Litterman model. representing the uncertainty in each view. Such views can be represented in absolute or relative terms using the Black-Litterman Model. Below are three examples of views stated in the Black and Litterman model (1990).

View 1: Merck (MRK) will generate an absolute return of 10% (Confidence of View = 50%).
View 2: Johnson & Johnson (JNJ) will outperform Procter & Gamble (PG) by 3% (Confidence of View = 65%).
View 3: GE (GE) will beat GM (gm), Wal-Mart (WMT), and Exxon (XOM) by 1.5 percent (Confidence of View = 30%).

An absolute view is exemplified by View 1. It instructs the Black-Litterman model to set Merck’s return at 10%.

Views 2 and 3 are relative views. Relative views are more accurate representations of how investment managers feel about certain assets. According to View 2, Johnson & Johnson’s return will be on average 3 percentage points higher than Procter & Gamble’s. To determine if this will have a good or negative impact on Johnson & Johnson in comparison to Procter & Gamble, their respective Implied Equilibrium returns must be evaluated. In general (and in the absence of constraints and other views), the model will tilt the portfolio towards the outperforming asset if the view exceeds the difference between the two Implied Equilibrium returns, as shown in View 2.

View 3 shows that the number of outperforming assets does not have to equal the number of failing assets, and that the labels “outperforming” and “underperforming” are relative terms. Views that include several assets with a variety of Implied Equilibrium returns are less intuitive, generalizing more challenges. In the absence of constraints and other views, the view’s assets are divided into two mini-portfolios: a long and a short portfolio. The relative weighting of each nominally outperforming asset is proportional to that asset’s market capitalization divided by the sum of the market capitalization of the other nominally outperforming assets of that particular view. Similarly, the relative weighting of each nominally underperforming asset is proportional to that asset’s market capitalization divided by the sum of the market capitalizations of the other nominally underperforming assets. The difference between the net long and net short positions is zero. The real outperforming asset(s) from the expressed view may not be the mini-portfolio that receives the good view. In general, the model will overweight the “outperforming” assets if the view is greater than the weighted average Implied Equilibrium return differential.

Why should I be interested in this post?

Modern Portfolio Theory (MPT) is at the heart of modern finance and its core foundations are structuring the modern investing panorama. MPT has established itself as the foundation for modern financial theory and practice. MPT’s premise is that beating the market is difficult, and those that do it by diversifying their portfolios appropriately and accepting higher-than-average investment risks.

MPT has been around for almost sixty years, and its popularity is unlikely to wane anytime soon. Its theoretical contributions have laid the groundwork for more theoretical research in the field of portfolio theory. Markowitz’s portfolio theory, however, is vulnerable to and dependent on continuing ‘probabilistic’ development and expansion. This article shed light on an enhancement of the initial Markowitz work by going a step further: to incorporate the views of the investors in the asset allocation process.

Useful resources

Academic research

Best, M.J., and Grauer, R.R. 1991. On the Sensitivity of Mean-Variance-Efficient Portfolios to Changes in Asset Means: Some Analytical and Computational Results.The Review of Financial Studies, 315-342.

Black, F. and Litterman, R. 1990. Asset Allocation: Combining Investors Views with Market Equilibrium. Goldman Sachs Fixed Income Research working paper

Black, F. and Litterman, R. 1991. Global Asset Allocation with Equities, Bonds, and Currencies. Goldman Sachs Fixed Income Research working paper

Black, F. and Litterman, R. 1992. Global Portfolio Optimization.Financial Analysts Journal, 28-43.

He, G. and Litterman, R. 1999. The Intuition Behind Black-Litterman Model Portfolios. Goldman Sachs Investment Management Research, working paper.

Idzorek, T.M. 2002. A step-by-step guide to Black-Litterman model. Incorporating user-specified confidence levels. Working Paper, 2-11.

Lee, W., 2000, Advanced theory and methodology of tactical asset allocation. Fabozzi and Associates Publications.

Markowitz, H., 1952. Portfolio Selection. The Journal of Finance, 7(1): 77-91.

Michaud, R.O. 1989. The Markowitz Optimization Enigma: Is Optimized Optimal?. Financial Analysts Journal, 31-42.

Mossin, J. 1966. Equilibrium in a Capital Asset Market. Econometrica, 34(4): 768-783.

Sharpe, W.F. 1963. A Simplified Model for Portfolio Analysis. Management Science, 9(2): 277-293.

Sharpe, W.F. 1964. Capital Asset Prices: A Theory of Market Equilibrium under Conditions of Risk. The Journal of Finance, 19(3): 425-442.

About the author

The article was written in November 2021 by Youssef LOURAOUI > (Bayes Business School, MSc. Energy, Trade & Finance, 2021-2022).

Smart Beta industry main actors

October 7, 2021 by Youssef LOURAOUI

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the main actors of the smart beta industry, which is estimated to represent a cumulative market value of $1.9 trillion as of 2017 and is projected to grow to $3.4 trillion by 2022 (BlackRock, 2021).

The structure of this post is as follows: we begin by presenting an overview of the smart beta industry actors. We will then discuss the case of BlackRock, the 10 trillion dollar powerhouse of the asset management industry, which is the main actor in the smart beta industry segment.

Overview of the market

The asset management sector, which is worth 100 trillion dollars worldwide, is primarily divided into active and passive management (BCG, 2021). While active management continues to dominate the market, passive management’s proportion of total assets under managed (AUM) increased by 4 percentage points between 2008 and 2019, reaching 15%. This market transition is even more dramatic in the United States, where passive management accounted for more than 40% of the total market share in 2019. A new category has arisen and begun to acquire market share over the last decade. Smart beta exchange-traded funds (ETFs) are receiving fresh inflows and are the industry’s fastest-growing ETF product. Various players are entering the market by developing and releasing new products (Deloitte, 2021).

Active funds have demonstrated divergent returns when compared to passive funds, making the cost difference increasingly difficult to justify (Figure 1). The growing market share of passive funds in both the United States and the European Union is putting further pressure on active managers’ fees. When it comes to meeting the demands of investors, both active and passive management has shown shortcomings. Active management funds often fail to outperform their benchmarks because they lack clear indicators, charge expensive fees, and don’t always have clear indicators. As seen in Figure 1, active funds struggle to deliver consistent returns over a prolonged timeframe, as depicted in the European market. In this sense, the active funds success rate is divided by more than half between year one and year three (Deloitte, 2021). Concentration is a problem for passive funds that are weighted by market capitalization.. These limits have prepared the ground for smart beta funds to emerge (Figure 1).

Figure 1. Active funds success rates (% of funds beating their index over X years)

Source: Deloitte (2021).

The smart beta market is dominated by several players who have a strategic position with a large volume of assets under management. Figure 2 compares smart beta actors based on percentage of asset under management (%AUM), one the most important metric in the asset management industry. Some key elements can be drawn for the first figure. BlackRock is the provider with the largest market share, with over 40% of the smart beta industry in the analysis, followed by Vanguard and State Street Global Advisors with 30.66% and 18.44% respectively in this benchmark study underpinning nearly $1 trillion (Figure 2).

Figure 2. % AUM of the biggest Smart Beta ETF providers
Smart_Beta_benchmark_analysis
Source: etf.com (2021).

BlackRock dominance

The main powerhouses of the passive investing industry, BlackRock and Vanguard, are poised to capture the lion’s share of assets in the rapidly rising world of actively managed exchange-traded funds. The conclusion is likely to dissatisfy active fund managers, who have been squeezed by the fast development of passive ETFs in recent years and may have seen the introduction of active ETFs as a chance to fight back and get a piece of the lucrative pie (Financial Times, 2021).

According to a study of 320 institutional investors with a combined $12.9 trillion in assets, institutional investors prefer BlackRock and Vanguard to handle their active ETF investments. The juggernauts were expected to provide the best performance as well as the best value for money. With over a third of the global ETF market capitalization, BlackRock remains the dominant player (The Financial Times, 2021). BlackRock is unquestionably a major force in the ETF business, with an unparalleled market share in both the US and European ETF markets. BlackRock has expanded to become the world’s largest asset manager, managing funds for everyone from pensioners to oligarchs and sovereign wealth funds. It is now one of the largest stockholders in practically every major American corporation — as well as a number of overseas corporations. It is also one among the world’s largest lenders to businesses and governments.

Aladdin, the company’s technological platform, provides critical wiring for large portions of the worldwide investing industry. By the end of June this year, BlackRock was managing a stunning $9.5 trillion in assets, a sum that would be hardly understandable to most of the 35 million Americans whose retirement accounts were managed by the business in 2020. If the current rate of growth continues, BlackRock’s third-quarter reports on October 13 might disclose that the company’s market capitalization has surpassed $10 trillion. It’s expected to have surpassed that mark by the end of the year (FT, 2021). To put this in perspective, it is about equivalent to the worldwide hedge fund, private equity, and venture capital industries combined.

Industry-wide fee cuts had helped BlackRock maintain its dominance in the ETF sector. Its iShares brand is the industry’s largest ETF provider for both passive and actively managed products (CNBC, 2021).

Why should I be interested in this post?

If you are a business school or university undergraduate or graduate student, this content will help you in understanding the various evolutions of asset management throughout the last decades and in broadening your knowledge of finance.

Smart beta funds have become a trending topic among investors in recent years. Smart beta is a game-changing invention that addresses an unmet need among investors: a higher return for lower risk, net of transaction and administrative costs. In a way, these investment strategies create a new market. As a result, smart beta is gaining traction and influencing the asset management industry.

Useful resources

Business analysis

BlackRock, 2021.What is factor investing?

BCG, 2021.The 100$ Trillion Machine: Global Asset Management 2021

CNBC, 2021. What Blackrock’s continued dominance means for other ETF issuers.

Deloitte, 2021. Will smart beta ETFs revolutionize the asset management industry? Understanding smart beta ETFs and their impact on active and passive fund managers

Etf.com, 2021.Smart Beta providers

Financial Times (13/09/2020) BlackRock and Vanguard look set to extend dominance to active ETFs

Financial Times (07/10/2021) The ten trillion dollar man: how Larry Fink became king of Wall St

About the author

The article was written in October 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

MSCI Factor Indexes

October 7, 2021 by Youssef LOURAOUI

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the MSCI Factor Indexes. MSCI is one of the most prominent actors in the indexing business, with approximately 236 billion dollars in assets benchmarked to the MSCI factor indexes.

The structure of this post is as follows: we begin by introducing MSCI Factor Indexes and the evolution of portfolio performance. We then delve deeper by describing the MSCI Factor Classification Standards (FaCS). We finish by analyzing factor returns over the last two decades.

Definition

Factor

A factor is any component that helps to explain the long-term risk and return performance of a financial asset. Factors have been extensively used in portfolio risk models and in quantitative investment strategies, and documented in academic research. Active fund managers use these characteristics while selecting securities and constructing portfolios. Factor indexes are a quick and easy way to get exposure to several return drivers. Factor investing aims to obtain greater risk-adjusted returns by exposing investors to stock features in a systematic way. Factor investing isn’t a new concept; it’s been utilized in risk models and quantitative investment techniques for a long time. Factors can also explain a portion of fundamental active investors’ long-term portfolio success. MSCI Factor Indexes use transparent and rules-based techniques to reflect the performance characteristics of a variety of investment types and strategies (MSCI Factor Research, 2021).

Performance analysis

Understanding portfolio returns is crucial to determining how to evaluate portfolio performance. It may be traced back to Harry Markowitz’s pioneering work and breakthrough research on portfolio design and the role of diversification in improving portfolio performance. Investors did not discriminate between the sources of portfolio gains throughout the 1960s and 1970s. Long-term portfolio management was dominated by active investment. The popularity of passive investment as an alternative basis for implementation was bolstered by finance research in the 1980s. Through passive allocation, investors began to effectively capture market beta. Investors began to perceive factors as major determinants of long-term success in the 2000s (MSCI Factor Research, 2021). Figure 1 presents the evolution of portfolio performance analysis over time: until the 1960s, based on the CAPM model, returns were explain by one factor only: the market return. Then, the market model was used to assess active portfolio with the alpha measuring the extra performance of the fund manager. Later on in the 2000s, the first evaluation model based on the market factor was augmented with other factors (size, value, etc.).

Figure 1. Evolution of portfolio performance analysis.

Source: MSCI Research (2021).

MSCI Factor Index

MSCI Factor Classification Standards (FaCS) establishes a standard vocabulary and definitions for factors so that they may be understood by a wider audience. MSCI FaCS is comprised of 6 Factor Groups and 14 factors and is based on MSCI’s Barra Global Equity Factor Model (MSCI Factor Research, 2021) as shown in Table 1.

Table 1 Factor decomposition of the different factor strategies.

Source: MSCI Research (2021).

The MSCI Factor Indexes are based on well-researched academic studies. The MSCI Factor Indexes were identified and developed based on academic results, creating a unified language to describe risk and return via the perspective of factors (MSCI Factor Research, 2021).

Performance of factors over time

Figure 2 compares the MSCI factor indexes’ performance from 1999 to May 2020. All indexes are rebalanced on a 100-point scale to ensure consistency in performance and to facilitate factor comparisons. Over a two-decade period, smart beta factors have all outperformed the MSCI World index, with the MSCI World Minimum Volatility Index as the most profitable factor which has consistently provided excess profits over the long run while (MSCI Factor research, 2021).

Figure 2. Performance of MSCI Factor Indexes during the period 1999-2017.

Source: MSCI Research (2021).

Individual factors have consistently outperformed the market over time. Figure 2 represents the performance of the MSCI Factor Indexes for the last two decades compared to the MSCI ACWI, which is MSCI’s flagship global equity index and is designed to represent the performance of large- and mid-cap stocks across 23 developed and 27 emerging markets.

It is possible to make some conclusions regarding the performance of the investment factor over the previous two decades by dissecting the performance of the various factorial strategies. The value factor was the one that drove performance in the first decade of the 2000s. This outperformance is characterized by a movement towards more conservative investment in a growing market environment. The dotcom bubble crash resulted in a bear market, with the minimal volatility approach helping to absorb market shocks in 2002. When it comes to the minimal volatility approach, it is evident that it is highly beneficial during moments of high volatility, acting as a viable alternative to hedging one’s stock market exposure and moving into more safe-haven products. Several times of extreme volatility may be recognized, including the dotcom boom, the US subprime crisis, and the European debt crisis as shown in Figure 3.

Figure 3. Table of performance of MSCI Factor Indexes from 1999-2017.

Source: MSCI Research (2021).

Why should I be interested in this post?

If you are a business school or university undergraduate or graduate student, this content will help you in understanding the evolution of asset management throughout the last decades and in broadening your knowledge of finance.

Useful resources

Business analysis

MSCI Factor Research, 2021.MSCI Factor Indexes

MSCI Factor Research, 2021. MSCI Factor Classification Standards (FaCS)

About the author

The article was written in October 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Smart beta 2.0

October 1, 2021 by Youssef LOURAOUI

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the concept of Smart beta 2.0, an enhancement of the first generation of smart beta strategies.

The structure of this post is as follows: we begin by defining smart beta 2.0 as a topic. We then discuss then the characteristics of smart beta 2.0.

Definition

“Smart beta 2.0” is an expression introduced by Amenc, Goltz and Martellini (2013) from the EDHEC-Risk Institute. This new vision of smart beta investment intends to empower investors to maximize the performance of their smart beta investments while managing their risk. Rather than offering solely pre-packaged alternatives to equity market-capitalization-weighted indexes, the Smart beta 2.0 methodology enables investors to experiment with multiple smart beta indexes to create a benchmark that matches their own risk preferences, and by extension increase their portfolio diversification overall.

Characteristics of smart beta 2.0 strategies

The main characteristic of smart beta 2.0 strategies compared to smart beta 1.0 strategies is portfolio diversification.

If factor-tilted strategies (i.e., portfolios with a part specifically invested in factor strategies) do not consider a diversification-based goal, they may result in very concentrated portfolios in order to achieve their factor tilts. Investors have lately started to integrate factor tilts with diversification-based weighting methods to create well-diversified portfolios using a flexible strategy known as Smart beta 2.0 (EDHEC-Risk Institute, 2016).

This method, in particular, enables the creation of factor-tilted indexes that are also adequately diversified by using a diversification-based weighting scheme. Because it combines the smart weighting scheme with the explicit factor tilt (Amenc et al., 2014), this strategy is also known as “smart factor investment”. In order to achieve extra value-added, investors are increasingly focusing on allocation choices across factor investing techniques.

The basic foundation for the smart beta has been substantially outstripped by its success with institutional investors. It is clear that market-capitalization-weighted indices have no counterpart when it comes to capturing market fluctuations (Amenc et al., 2013). Even the harshest detractors of market-capitalization-weighted, in the end, use market-capitalization-weighted indices to assess the success of their own new indexes (Amenc et al., 2013). In fact, because smart beta strategies outperform market-capitalization-weighted indexes, the great majority of investors are likely to pick them. While everyone believes cap-weighted indexes provide the most accurate representation of the market, they do not always provide an efficient benchmark that can be used as a reference for a strategic allocation. It’s worth noting that smart beta 2.0 seeks to close the gap in terms of exposure to factors from the first generation, but it doesn’t guarantee outperformance over market-capitalization-weighted strategies (Amenc et al., 2013).

Why should I be interested in this post?

If you are a business school or university undergraduate or graduate student, this content will help you in understanding the evolution of asset management during the last decades and in broadening your knowledge of finance.

Smart beta funds have become a hot issue among investors in recent years. Smart beta is a game-changing invention that addresses an unmet need among investors: a higher return for lower risk, net of transaction and administrative costs. In a way, these strategies (smart beta 1.0 and then smart beta 2.0) have created a new market. As a result, smart beta is gaining traction and influencing the asset management industry.

Useful resources

Amenc, N., F., Goltz, F., Le Sourd, V., 2016. Investor perception about Smart beta ETF. EDHEC-Risk Institute working paper.

Amenc, N., F., Goltz, F., Martellini, L., 2013. Smart beta 2.0. EDHEC-Risk Institute working paper.

Amenc, N., F., Goltz, F., Martinelli, L., Deguest, R., Lodh, A., Shirbini, E., 2014. Risk Allocation, Factor Investing and Smart Beta: Reconciling Innovations in Equity Portfolio Construction. EDHEC-Risk Institute working paper.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Smart Beta 1.0

September 23, 2021 by Youssef LOURAOUI

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the concept of the smart beta 1.0, the first generation of alternative indexing investment strategies that created a new approach in the asset management industry.

This post is structured as follows: we start by defining smart beta 1.0 as a topic. Finally, we discuss an empirical study by Motson, Clare and Thomas (2017) emphasizing the origin of smart beta.

Definition

The “Smart Beta” expression is commonly used in the asset management industry to describe innovative indexing investment strategies that are alternatives to the market-capitalization-weighted investment strategy (buy-and-hold). In terms of performance, the smart beta “1.0” approach outperforms market-capitalization-based strategies. According to Amenc et al. (2016), the latter have a tendency for concentration and unrewarded risk, which makes them less appealing to investors. In finance, “unrewarded risk” refers to taking on more risk without receiving a return that is commensurate to the increased risk.

When smart beta techniques were first introduced, they attempted to increase portfolio diversification over highly concentrated and capitalization-weighted, as well as to capture the factor premium available in equity markets, such as value indices or fundamentally weighted indices which aim to capture the value premium. While improving capitalization-weighted indices is important, concentrating just on increasing diversity or capturing factor exposure may result in a less than optimal outcome. The reason for this is that diversification-based weighting systems will always result in implicit exposure to certain factors, which may have unintended consequences for investors who are unaware of their implicit factor exposures. Unlike the second generation of Smart Beta, the first generation of Smart Beta are integrated systems that do not distinguish between stock selection and weighting procedures. The investor is therefore required to be exposed to certain systemic risks, which are the source of the investor’s poor performance.

Thus, the first-generation Smart Beta indices are frequently prone to value, small- or midcap, and occasionally contrarian biases, since they deconcentrate cap weighted indices, which are often susceptible to momentum and large growth risk. Furthermore, distinctive biases on risk indicators that are unrelated to deconcentration but important to the factor’s objectives may amplify these biases even further. Indexes that are fundamentally weighted, for example, have a value bias because they apply accounting measures that are linked to the ratios that are used to construct value indexes.

Empirical study: monkeys vs passive mangers

Andrew Clare, Nick Motson, and Steve Thomas assert that even monkey-created portfolios outperform cap-weighted benchmarks in their study (Motson et al., 2017). A lack of variety in cap-weighting is at the foundation of the problem. The endless monkey theory states that a monkey pressing random keys on a typewriter keyboard for an unlimited amount of time will almost definitely type a specific text, such as Shakespeare’s whole works. For 500 businesses, there is an infinite number of portfolio weighting options totaling 100%; some will outperform the market-capitalization-weighted index, while others will underperform. The authors of the study take the company’s ticker symbol and use the following guidelines to create a Scrabble score for each stock:

A, E, I, O, U, L, N, S, T, R – 1 point. D and G both get two points.
B, C, M, P – 3 points ; F, H, V, W, Y – 4 points ; K – 5 points.
J, X – 8 points ; Q, Z – 10 points

The scores of each company’s tickers are then added together and divided by this amount to determine each stock’s weight in the index. As illustrated in Figure 1, the results obtained are astonishing, resulting in a clear outperformance of the randomly generated portfolios compared to the traditional market capitalization index by 1.5% premium overall.

Figure 1. Result of the randomly generated portfolio with the Cass Scrabble as underlying rule compared to market-capitalization portfolio performance.
Scrabble_performance
Source: Motson et al. (2017).

In the same line, the authors produced 500 weights that add up to one using this technique, with a minimum increase of 0.2 percent. The weights are then applied to a universe of 500 equities obtained from Bloomberg in December 2015 (Motson et al., 2017). The performance of the resultant index is then calculated over the next twelve months. This technique was performed ten million times. As illustrated in Figure 2, the results are striking, with smart beta funds outperforming nearly universally in the 10 million simulations run overall, and with significant risk-adjusted return differences (Motson et al., 2017).

Figure 2. 10 million randomly generated portfolios based on a portfolio construction of 500 stocks
Scrabble_performance
Source: Motson et al. (2017).

For performance analysis, the same method was employed, but this time for a billion simulation. This means they constructed one billion 500-stock indexes with weights set at random or as if by a monkey. Figure 9 suggests that the outcome was not accidental. The black line shows the distribution of 1 billion monkeys’ returns in 2016, while the grey line shows the cumulative frequency. 88 percent of the monkeys outperformed the market capitalization benchmark, according to the graph. The luckiest monkey returned 27.2 percent, while the unluckiest monkey returned just 3.83 percent (Motson et al., 2017) (Figure 3).

FFigure 3. Result of one billion randomly simulated portfolios based on a portfolio construction of 500 stocks.
Scrabble_performance
Source: Motson et al. (2017).

Why should I be interested in this post?

If you’re an investor, you’re probably aware that smart beta funds have become a popular topic. Smart beta is a game-changing development that fills a gap in the market for investors: a better return for a reduced risk, net of transaction and administrative costs. These strategies, in a sense, establish a new market. As a result, smart beta is gaining traction and having an impact on asset management.

Useful resources

Academic research

Amenc, N., F., Goltz, F. and Le Sourd, V., 2016. Investor perception about Smart beta ETF. EDHEC Risk Institute working paper.

Amenc, N., F., Goltz, F. and Martinelli, L., 2013. Smart beta 2.0. EDHEC Risk Institute working paper.

Motson, N., Clare, A. & Thomas, S., 2017. Was 2016 the year of the monkey?. Cass Business School research paper.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Alternative to market-capitalization weighting strategies

September 23, 2021 by Youssef LOURAOUI

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the different alternatives developed to the market-capitalization weighting strategy (buy-and-hold strategy).

The structure of this post is as follows: we begin by introducing alternatives to market capitalization strategies as a topic. We then will delve deeper by presenting heuristic-based weighting and optimization-based weighting strategies.

Introduction

The basic rule of applying a market-capitalization weighting methodology for the development of indexes has recently come under fire. As the demand for indices as investment vehicles has grown, different weighting systems have emerged. There have also been a number of recent projects for non-market-capitalization-weighted ETFs. Since the first basic factor weighted ETF was released in May 2000, a slew of ETFs has been released to monitor non-market-cap-weighted indexes, including equal-weighted ETFs, minimal variance ETFs, characteristics-weighted ETFs, and so on. These are dubbed “Smart Beta ETFs” since they aim to outperform traditional market-capitalization-based indexes in terms of risk-adjusted returns (Amenc et al. 2016).

The categorization approach will be the same as Chow, Hsu, Kalesnik, and Little (2011), with the following distinctions: 1) basic weighting techniques (heuristic-based weighting) and 2) more advanced quantitative weighting techniques (optimization-based weighting).

It’s an arbitrary categorization system designed to make reading easier by differentiating between simpler and more complicated approaches.

Heuristic-based weighting strategies

Equal-weighting

The equal weighting method assigns the same weight to each share making up the portfolio (or index)

Where w_i represents the weight of asset i in the portfolio and N the total number of assets in the portfolio.

Because each component of the portfolio has the same weight, equal weighting helps investors to obtain more exposure to smaller firms. Bigger firms will be more represented in the market-capitalization-weighted portfolio since their weight will be larger. The benefit of this technique is that tiny capitalization risk-adjusted-performance tends to be better than big capitalization (Banz, 1981).

In their study, Arnott, Kalesnik, Moghtader, and Scholl (2010) created three distinct indices in terms of index composition. The first group consists of enterprises with substantial market capitalization (as are capitalisation-weighted indices). Each business in the index is then given equal weight. This is how the majority of equally-weighted indexes are built (MSCI World Equal Index, S&P500 Equal Weight Index). The second is to create an index based on basic criteria and then assign equal weight to each firm. The third strategy is a hybrid of the first two. It entails averaging the ranks from the two preceding approaches and then assigning equal weight to the remaining 1000 shares.

Fundamental-weighting

The weighting approach based on fundamentals divides companies into categories based on their basic size. Sales, cash flow, book value, and dividends are all taken into account. These four parameters are used to determine the top 1,000 firms, and each firm in the index is given a weight based on the magnitude of their individual components (Arnott et al., 2005). The portfolio weight of the _ith stock is defined as:

For a fundamental index that includes book value as a consideration, for example, the top 1,000 companies in the market with the most extensive book values are chosen. Firm x_i is given a weight w_i, which is equal to the firm’s book value divided by the total of the index components’ book values.

Fundamental indexation tries to address the following bias: in a cap-weighted index, if the market efficiency hypothesis is not validated and a share’s price is, for example, overpriced (greater than its fair value), the share’s weight in the index will be too high. Weighting by fundamentals will reduce the bias of over/underweighting over/undervalued companies based on criteria like sales, cash flows, book value, and dividends, which are not affected by market opinion, unlike capitalization.

Low beta weighting

Low-beta strategies are based on the fact that equities with a low beta have greater returns than those expected by the CAPM (Haugen and Heins, 1975). A beta of less than one indicates that the share price has tended to grow less than its benchmark index during bullish trends and to decrease less severely during negative trends throughout the observed timeframe. A low-beta index is created by selecting low-beta stocks and then giving each stock equal weight in the index. As a result, it’s a hybrid of a low-beta and an equal-weighting method. On the other side, high beta strategies enable investors to profit from the amplification of favourable market moves.

Reverse-capitalization weighting

The weight of an asset capitalization-weighted index can be defined as:

where MC stands for “Market Capitalization”, and w_i is the weight of asset i in the portfolio.

In a reverse market-capitalization-weighted index, the weight of an asset is defined as:

“Reverse market-capitalization” is abbreviated as RMC. This technique necessitates using a cap-weighted index to execute the approach. RCW methods, like equal-weight or low-beta strategies, are motivated by the fact that small caps have a greater risk-adjusted return than big caps. This sort of indexation requires constant rebalancing (Banz, 1981).

Maximum diversification

This technique aims to build a portfolio with as much diversification as feasible. A diversity index (DI) is employed to achieve the desired outcome, which is defined as the distance between the sum of the constituents’ volatilities and the portfolio’s volatility (Amenc, Goltz, and Martellini, 2013). Diversity weighting is one of the better-known portfolio heuristics that blend cap weighting and equal weighting. Fernholz (1995) defined stock market diversity, D_p, as

where p between (0,1) and x _Market,i is the weight of the _ith stock in the cap-weighted market portfolio, and then proposed a strategy of portfolio weighting whereby portfolio weights are defined as

where i = 1, . . . , N; p between (0,1); and the parameter p targets the desired level of portfolio tracking error against the cap-weighted index.

Optimization-based weighting strategies

The logic of Modern Portfolio Theory (Markowitz, 1952) is followed in Mean-Variance optimization. Theoretically, if we know the expected returns of all stocks and their variance-covariance matrix, we can construct risk-adjusted-performance optimal portfolios. However, these two inputs for the model are difficult to estimate precisely in practice. Chopra and Ziemba (1993) showed that even little inaccuracies in these parameters’ estimates may have a large influence on risk-adjusted-performance.

Minimum Variance

Chopra and Ziemba (1993) adopt the simple premise that all stocks have the same return expectation, based on the fact that stock return expectations are difficult to quantify. As a result of this premise, the best portfolio is the one that minimizes risk. The goal of minimal variance strategies, which have been around since 1990, is to provide a better risk-return profile by lowering portfolio risk without modifying return expectations. The low volatility anomaly justifies this technique. Low-volatility stocks have historically outperformed high-volatility equities. These portfolios are built without using a benchmark as a guide. The portfolio variance minimization equation for a two-asset portfolio is as follows:

In their research on the construction of this type of index, Arnott, Kalesnik, Moghtader and Scholl (2010) found that risk measures that take into account interest rates, oil prices, geographical region, sector, size, expected return, and growth, as calculated by the Northfield global risk model, a model for making one-year risk forecasts, reduce the portfolio’s absolute risk. This method is used in the MSCI World Minimum Volatility Index, which was released in 2008.

Global Minimum Variance, Maximum Decorrelation, and Diversified Minimum Variance are the three types of minimum variance techniques (Amenc, Goltz and Martellini, 2013). However, there are no indexes or exchange-traded funds (ETFs) based on the Maximum Decorrelation and Diversified Minimum Variance methods in actuality; they are still only theoretical notions.

Maximum Sharpe ratio

Because all stocks are unlikely to have the same expected returns, the minimum-variance portfolio—or any practical representation of its concept—is unlikely to have the highest ex-ante Sharpe ratio. Investors must incorporate useful information about future stock returns into a minimum-variance approach to improve it. Choueifaty and Coignard (2008) proposed a simple linear relationship between the expected premium, E(R_i) – R_f, for a stock and its return volatility, sigma_i:

A related portfolio method proposed by Amenc, Goltz, Martellini, and Retkowsky (2010) implies that a stock’s expected returns are linearly related to its downside semi-volatility. They claimed that portfolio losses are more important to investors than gains. As a result, rather than volatility, risk premium should be connected to downside risk (semi-deviation below zero). The EDHEC-Risk Efficient Equity Indices are built around this assumption. Downside semi-volatility can be defined mathematically as

where R_{i, t} is the return for stock _i in period _t.

Maximum Sharpe ratio can be considered as an alternative beta technique that aims to solve the challenges of forecasting risks and returns for a large number of equities.

Why should I be interested in this post?

Smart beta funds have become a hot issue among investors in recent years. Smart beta is a game-changing invention that addresses an unmet need among investors: a higher return for lower risk, net of transaction and administrative costs. In a way, these investment strategies create a new market. As a result, smart beta is gaining traction and influencing the asset management industry.

Useful resources

Academic research

Amenc, Noël, Felix Goltz, Lionel Martellini, and Patrice Ret- kowsky. 2010. “Efficient Indexation: An Alternative to Cap- Weighted Indices.” EDHEC-Risk Institute (February).

Amenc, N., Goltz, F., Le Sourd, V., 2016. Investor perception about Smart beta ETF. EDHEC Risk Institute working paper.

Amenc, N., Goltz, F., Martinelli, L., 2013. Smart beta 2.0. EDHEC Risk Institute working paper.

Arnot, R.D., Hsu, J., Moore, P., 2005. Fundamental Indexation. Financial Analysts Journal, 61(2):83-98.

Arnot, R.D., Kalesnik, V., Moghtader, P., Scholl, S., 2010. Beyond Cap Weight, The empirical evidence for a diversified beta. Journal of Indexes, January, 16-29.

Banz, R., 1981. The relationship between return and market value of common stocks. Journal of Financial Economics. 9(1):3-18.

Chopra, V., Ziemba, W., 1993. The Effect of Errors in Means, Variances, and Covariances on Optimal Portfolio Choice. Journal of Portfolio Management, 19:6-11.

Chow, T., Hsu, J., Kalesnik, V., Little, B., 2011. A Survey of Alternative Equity Index Strategies. Financial Analyst Journal, 67(5):35-57.

Choueifaty, Yves, and Yves Coignard. 2008. Toward Maximum Diversification. Journal of Portfolio Management, vol. 35, no. 1 (Fall):40–51.

Fernholz, Robert. 1995. Portfolio Generating Functions. Working paper, INTECH (December).

Haugen, R., Heins, J., 1975. Risk and Rate of Return of Financial Assets: Some Old Wine in New Bottles. Journal of Financial and Quantitative Analysis, 10(5):775-784.

Markowitz, H., 1952. Portfolio Selection. The Journal of Finance, 7(1):77-91.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Markowitz Modern Portfolio Theory

January 30, 2024September 20, 2021 by Youssef LOURAOUI

Markowitz Modern Portfolio Theory

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents Markowitz’s Modern Portfolio Theory, a pioneering framework for understanding the impact of the number of stocks in a portfolio and their covariance relationships on portfolio diversification.

We begin by presenting Markowitz’s Modern Portfolio Theory (MPT) as the origin of factor investing (market factor). The assumptions of the model are then discussed. We’ll go through some of the model’s fundamental concepts next. We wrap up with a discussion of the concept’s limitations and a general conclusion.

Modern Portfolio Theory

The work conducted by Markowitz is widely acknowledged as a pioneer in financial economics and corporate finance for his theoretical implications and its application in financial markets. In 1990, Markowitz shared the Nobel Prize for his contributions to these domains, which he articulated in his 1952 article “Portfolio Selection” published in The Journal of Finance. His seminal work laid the groundwork for what is now often referred to as ‘Modern Portfolio Theory’ (MPT).

Modern portfolio theory was first introduced by the work of Harry Markowitz in 1952. Overall, the risk component of MPT can be quantified using various mathematical formulations and mitigated through the concept of diversification, which entails carefully selecting a weighted collection of investment assets that collectively exhibit lower risk characteristics than any single asset or asset class. Diversification is, in fact, the central notion of MPT and is predicated on the adage “never put all your eggs in one basket”.

Assumptions of the Markowitz Portfolio Theory

MPT is founded on several market and investor assumptions. Several of these assumptions are stated explicitly, while others are implied. Markowitz’s contributions to MPT in portfolio selection are based on the following basic assumptions:

Investors are rational (they seek to maximize returns while minimizing risk).
Investors will accept increased risk only if compensated with higher expected returns.
Investors receive all pertinent information regarding their investment decision in a timely manner.
Investors can borrow or lend an unlimited amount of capital at a risk-free rate of interest.

Concepts used in the MPT

Risk

Risk is equivalent to volatility in Markowitz’ portfolio selection theory—the larger the portfolio volatility, the greater the risk. Volatility is a term that refers to the degree of risk or uncertainty associated with the magnitude of variations in a security’s value. Risk is the possibility that an investment’s actual return will be less than predicted, which is technically quantified by standard deviation. A larger standard deviation implies a bigger risk and, hence, a larger potential return. If investors are prepared to take on risk, they anticipate earning a risk premium. Risk premium is defined as “the expected return on an investment that exceeds the risk-free rate of return”. The bigger the risk, the more risk premium investors need.”. Riskier investments do not necessarily provide a higher rate of return than risk-free ones. This is precisely why they are hazardous. However, historical evidence suggests that the only way for investors to obtain a better rate of return is to take on greater risk.

Systematic risk

Systematic risk is a type of risk at the macroeconomic level—risk that impacts a large number of assets to varying degrees. Inflation, interest rates, unemployment rates, currency exchange rates, and Gross National Product levels are all instances of systematic risk variables. These economic conditions have a significant influence on practically all securities. As a result, systemic risk cannot be completely eradicated.

Unsystematic risk

Unsystematic risk (or specific risk), on the other hand, is a type of risk that occurs at the micro-level risk factors that influence only a single asset or a small group of assets. It entails a distinct risk that is unrelated to other hazards and affects only particular securities or assets. For instance, Netflix’s poorly accepted adjustment to its planned consumer pricing structure elicited an extraordinarily unfavorable consumer response and defections, resulting in decreased earnings and stock prices. However, it had little effect on the Dow Jones or S&P 500 indexes, or on firms in the entertainment and media industries in general—with the probable exception of Netflix’s largest rival Blockbuster Video, whose value grew dramatically as a result of Netflix’s declining market share. Additional instances of unsystematic risk include a firm’s credit rating, poor newspaper coverage of a corporation, or a strike impacting a specific company. Diversification of assets within a portfolio can greatly minimize unsystematic risk.

Because the returns on various assets are, in fact, connected to some extent, unsystematic risk can never be totally avoided regardless of the number of asset classes pooled in a portfolio. The Markowitz Efficient Frontier is depicted in Figure 1, with all efficient portfolios on the upper line. The efficient frontier is a set of optimal portfolios that offer the best-projected return for a specified level of risk, or the lowest risk for a specified level of return. Portfolios that fall below the efficient frontier are inefficient because they do not generate a sufficient rate of return in relation to the level of risk (Figure 1).

Figure 1. Markowitz Efficient Frontier.

Source: computations by the author.

Risk-return trade-off

The term risk-return trade-off refers to Markowitz’s fundamental theory that the riskier an investment, the larger the necessary potential return (or expected return). Investors will generally retain a hazardous investment only if the predicted return is sufficiently high to compensate them for taking the risk. Markowitz derives a relation between expected return (μ) and variance (σ²_p) captured in the following expression. Refer to the post Implementation of the Markowitz allocation model for a better understanding of the mathematical foundations of this approach:

img_SimTrade_variance_Markowitz_portfolio

where

A, B and C = Optimization parameters
μ = expected return vector

Diversification

The words ‘diversification’ and ‘Diversification Effect’ relate to the correlations between portfolio risk and diversification. Diversification, a tenet of Markowitz’s portfolio selection theory and MPT, is a risk-reduction strategy that entails allocating assets among a variety of financial instruments, sectors, and other asset classes. In more straightforward terms, it refers to the aphorism “don’t put all your eggs in one basket.” If the basket is dropped, all eggs are shattered; if many baskets are used, the likelihood of all eggs being destroyed is significantly decreased. Diversification may be accomplished by investments in a variety of companies, asset types (e.g., bonds, real estate, etc.), and/or commodities such as gold or oil.

Diversification seeks to enhance returns while minimizing risk by investing in a variety of assets that will react differently to the same event (s). For example, whenever there is unfavorable news about the European debt crisis, the stock market typically declines dramatically. Simultaneously, the same news has generally benefited the price of specific commodities, such as gold. As a result, portfolio diversification methods should include not just diverse stocks inside and outside of the same industry, but also diverse asset classes, such as bonds and commodities. The Diversification Effect is a term that relates to the link between portfolio correlations and diversification. When there is an imperfect connection between assets (positive or negative), the diversification effect occurs. It is a critical and successful risk mitigation method since risk mitigation may be accomplished without jeopardizing profits. As a result, any prudent investor who is ‘risk cautious’ will diversify to a certain extent.

Limitation of the model

Despite its monumental theoretical significance, MPT has a slew of opponents who contend that its underlying assumptions and modeling of financial markets are frequently out of step with reality. One could argue that none of them are totally accurate and that each of them undermines MPT to varied degrees. Generally, some of the most common complaints include the following: irrationality of investors, relation between risk and return, treatment of information by investors, limitless borrowing capacity, perfectly efficient markets, and no taxes or transaction costs.

Irrationality of investors

It is assumed that investors are rational and aim to maximize returns while reducing risk. This is contrary to what market participants who become swept up in ‘herd behavior’ investment activity observe. For example, investors frequently gravitate into ‘hot’ industries, and markets frequently boom or burst because of speculative excesses.

Relation between risk and expected return

Increased risk = Increased expected returns. The idea that investors will only take more risk in exchange for higher predicted profits is regularly refuted by investor behavior. Frequently, investing techniques need investors to make a perceived hazardous investment (e.g., derivatives or futures) in order to lower total risk without increasing projected profits significantly. Additionally, investors may have certain utility functions that override worries about return distribution.

Treatment of information by investors

MPT anticipates that investors will get all information pertinent to their investment in a timely and thorough manner. In fact, global markets are characterized by information asymmetry (one party possesses superior knowledge), insider trading, and investors who are just more knowledgeable than others. This may explain why stocks, commercial assets, and enterprises are frequently acquired at a discount to their book or market value.

Limitless Borrowing Capacity

Another critical assumption mentioned previously is that investors have nearly unlimited borrowing capacity at a risk-free rate. Each investor has credit constraints in real-world markets. Additionally, only the federal government may borrow at the zero-interest treasury bill rate on a continuous basis.

Perfectly efficient markets

Markowitz’s theoretical contributions to MPT are predicated on the premise that markets are perfectly efficient (Markowitz, 1952). On the other hand, because MPT is based on asset values, it is susceptible to market whims such as environmental, personal, strategic, or social investment choice factors. Additionally, it ignores possible market failures like as externalities (costs or benefits that are not reflected in pricing), information asymmetry, and public goods (a non-rivalrous and non-excludable item). From another vantage point, centuries of ‘rushes’, ‘booms’, ‘busts’, ‘bubbles’, and ‘market crises’ illustrate that markets are far from efficient.

No Taxes or Transaction Costs

Neither taxes nor transaction costs are included in Markowitz’ theoretical contributions to MPT. To the contrary, genuine investment products are subject to both taxes and transaction costs (e.g., broker fees, administrative charges, and so on), and considering these costs into portfolio selection may certainly affect the optimal portfolio composition.

Conclusion

MPT has become the de facto dogma of contemporary financial theory and practice. The idea of MPT is that beating the market is tough, and those that do do it by diversifying their portfolios properly and taking above-average investing risks. The critical point to remember is that the model is only a tool—albeit the most powerful hammer in one’s financial toolbox. It has been over sixty years since Markowitz introduced MPT, and its popularity is unlikely to decrease anytime soon. His theoretical insights have served as the foundation for more theoretical investigation in the field of portfolio theory. Nonetheless, Markowitz’s portfolio theory is susceptible to and dependent on ongoing ‘probabilistic’ development and expansion.

Why should I be interested in this post?

Modern Portfolio Theory is at the heart of modern finance and its core foundations are structuring the modern investing panorama. MPT has established itself as the foundation for modern financial theory and practice. MPT’s premise is that beating the market is difficult, and those that do it by diversifying their portfolios appropriately and accepting higher-than-average investment risks.

MPT has been around for almost sixty years, and its popularity is unlikely to wane anytime soon. His theoretical contributions have laid the groundwork for more theoretical research in the field of portfolio theory. Markowitz’s portfolio theory, however, is vulnerable to and dependent on continuing ‘probabilistic’ development and expansion.

Useful resources

Academic research

Ang, A., 2013. Factor Investing. Working paper.

Mangram, M.E., 2013. A simplified perspective of the Markowitz Portfolio Theory. Global Journal of Business Research, 7(1): 59-70.

Markowitz, H., 1952. Portfolio Selection. The Journal of Finance, 7(1): 77-91.

Mossin, J. 1966. Equilibrium in a Capital Asset Market. Econometrica, 34(4): 768-783.

Sharpe, W.F. 1963. A Simplified Model for Portfolio Analysis. Management Science, 9(2): 277-293.

Sharpe, W.F. 1964. Capital Asset Prices: A Theory of Market Equilibrium under Conditions of Risk. The Journal of Finance, 19(3): 425-442.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Smart Beta strategies: between active and passive allocation

September 20, 2021 by Youssef LOURAOUI

Smart Beta strategies: between active and passive allocation

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) discusses the topic of smart beta strategies and especially the debate about its position as an active or passive allocation.

Smart beta strategies appear to be in the middle of the polarized asset management industry, which is segmented between active investing based on beating the performance of a given benchmark, and passive investing based on replicating a given benchmark.

This article is structured as follows: we begin by introducing the topic of smart beta strategies. We then discuss the different investing approach and their characteristic. A simple simulation exercise is then presented to understand how an alternative to market-capitalization-weightings indexes leads to different results. We wrap up with a general conclusion of the topic.

Introduction

Smart beta strategies are often found somewhere in the middle between active and passive investment management. In this post, we look at how investors think about this characteristic of smart beta investment strategies.

Passive funds aim at replicating or tracking an index (such as the S&P500 index in the US or the CAC40 index in France for equity markets) use a buy-and-hold strategy to achieve their goal of mimicking the performance of the market index. The beta of a passive fund is very close to the beta of the market index (benchmark).

Active funds are supervised by a portfolio manager that screens the best investments for the fund and time the market to profit from an upside potential. The excess return over the performance of the market index (benchmark) is referred to as alpha.

Smart beta funds are justified by the fact that capitalization-weighted strategies appear to be inefficient. They are based on transparent and rule-based strategies. Investors seek to obtain additional factor betas to enhance their portfolio performance.

While passive investing aims to match the market return, and active strategies rely on the fund manager’s ability to outperform the market, smart beta can be seen as a hybrid of the two approaches, with a passive component in the sense that it tracks one or more factors that are transparent and rule-based, and an active component in which the portfolio is managed, that is to say, rebalanced from time to time. Table 1 describes the main types of funds (passive, active and smart beta) and their respective strategies according to the investment approach and asset allocation methodology, and performance metrics. We also indicate the Greek letter that each strategy.

Table 1. Description of the main types of funds and their respective strategies.

Source: table done by the author.

The passive investing approach

The Efficient Market Hypothesis (EMH) asserts that markets are efficient. The passive investing strategy is built on the concept of “buy-and-hold,” or keeping an investment position for a lengthy period without worrying about market timing or acting on the bought position. This latter technique is frequently implemented through the purchase of exchange-traded funds (ETF) that aim to closely match a given benchmark to produce a performance that is comparable to the underlying index or benchmark. The index might be broad-based, such as the S&P500 index in the US equity market for instance, or more specialized, such as an index that monitors a specific sector or geographical zone.

The active investing approach

Active management is an approach for going beyond matching a benchmark’s performance and instead aiming to outperform it. The alpha may be calculated using the same CAPM model framework, by linking the expected return with the fund manager’s extra return on the portfolio’s overall performance (Jensen, 1968). The search for alpha is done through two very different types of investment approaches: stock picking and market timing.

Stock picking

Stock picking is a method used by active managers to select assets based on a variety of variables such as their intrinsic value, the growth rate of dividends, and so on. Active managers use the fundamental analysis approach, which is based on the dissection of economic and financial data that may impact the asset price in the market.

Market timing

Market timing is a trading approach that involves entering and exiting the market at the right time. In other words, when rising outlooks are expected, investors will enter the market, and when downward outlooks are expected, investors will exit. For instance, technical analysis, which examines price and volume of transactions over time to forecast short-term future evolution, and fundamental analysis, which examines the macroeconomic and microeconomic data to forecast future asset prices, are the two techniques on which active managers base their decisions.

Review of academic literature

Passive investing

We can retrace the foundations of passive investing to the theory of portfolio construction developed by Harry Markowitz. For his theoretical implications, Markowitz’s work is widely regarded as a pioneer in financial economics and corporate finance. For his contributions to these disciplines, which he developed in his thesis “Portfolio Selection” published in The Journal of Finance in 1952. Markowitz received the Nobel Prize in economics in 1990. His groundbreaking work set the foundation for what is now known as ‘Modern Portfolio Theory’ (MPT).

William Sharpe, John Lintner, and Jan Mossin separately developed The Capital Asset Pricing Model (CAPM) as a result of Markowitz past research. The CAPM was a huge evolutionary step forward in capital market equilibrium theory because it enabled investors to appropriately value assets in terms of systematic risk. The portfolio management industry intended to capture the market portfolio return in the late 1970s, defined as a hypothetical collection of investments that contains every kind of asset available in the investment universe, with each asset weighted in proportion to its overall market participation. A market portfolio’s expected return is the same as the market’s overall expected return. But as financial research evolved and some substantial contributions were made, new factor characteristics emerged to capture some additional performance.

Active investing

As fund managers tried strategies to beat the market, financial literature delved deeper into the mechanism to achieve this purpose. Jensen’s groundbreaking work in the early ’70s gave rise to the concept of alpha in the tracking of a fund’s performance to distinguish between the fund’s manager’s ability to generate abnormal returns and the part of the returns due to luck (Jensen, 1968).

Smart beta / factor investing

Smart beta is defined as strategies that aim to address the inefficiencies of market capitalization weight indexation. In the early 2000s, as a result of numerous financial publications delving deeper into various elements that gave additional returns to increase the overall performance of the portfolio (the “Fama-French” papers), smart beta strategies evolved. Fund managers develop investment strategies based on researched factors that provide a time-tested abnormal return in exchange for taking on risk.

Understanding portfolio returns is crucial to determining how to evaluate portfolio performance. It all stems from Harry Markowitz’s groundbreaking work and pioneering research on portfolio construction and the impact of diversification in improving portfolio performance. Throughout the 1960s and 1970s, investors made no distinction between the sources of portfolio returns. Finance research in the 1980s boosted the popularity of passive investment as an alternate basis for implementation. Investors began to successfully capture market beta through passive strategies. In the 2000s, investors began to see factors as major determinants of long-term return (Figure 1).

Figure 1. Overview of the evolution of performance metrics.

Source: MSCI Factor Research (2021).

Grossman and Stiglitz’s research addressed the limitations of passive investment (1980). If the fund manager actively selects assets for his portfolio rather than passively replicating the benchmark, he may get higher abnormal returns. The term “abnormal returns” refers to the disparity between the actual and projected returns. In the financial literature, this “extra return” is referred to as alpha. It is one of the most tracked performance indicators by fund managers. Grossman and Stiglitz establish that there is no such thing as a successful passive investment. Indeed, they said that the benchmark is composed of assets chosen based on certain criteria (capitalization, return, liquidity, and the weight of each asset in the sector), and that “passive investing” is the most cost-effective alternative to active investing.

As pointed out by Jensen (1968), when assembling a portfolio, there are two points to bear in mind. The first point is the fund manager’s ability to foresee the asset’s price movement, and the second point is the fund manager’s capacity to limit investment risk via diversification.

Case study: Comparison of market-capitalization-weighted portfolios and equally-weighted portfolios

The difference between two investment strategies can be evaluated by comparing the weights of the assets of their associated portfolio. Note that over time the weights can evolve with voluntary sales and purchases of the assets. Such divestments and investments refer to the rebalancing of the portfolio.

Buy-and-hold investing is a passive investment strategy in which an investor buys assets and holds them for a long period, independent of market fluctuations. A buy-and-hold investor selects companies but is indifferent to short-term market swings or technical indicators. The buy-and-hold investment strategy corresponds to market-capitalization-weighted portfolios.

The buy-and-hold approach is recommended by several prominent investors, like Warren Buffett, to individuals seeking profitable long-term returns. Buy-and-hold investors outperform active management on average over longer time horizons and after costs. Buy-and-hold investors, on the other hand, may not sell at the greatest price available, according to proponents.

Excel file for market-capitalization-weighted and equally-weighted portfolios

You can download an Excel file with data used for this exercise.

The goal of this exercise is to compare the performance of the two types of investments and to balance the two approaches to obtain a better understanding of each strategy and its market behavior. To be able to homogeneously analyze the underlying assets of the buy and hold strategy as well as the smart beta approach, three stocks have been simulated.

All the price data, number of shares, stock returns, and market-capitalization are all simulated for a more simplistic model. The buy and hold strategy is based on an evenly weighted portfolio. Only the small-cap stock (Stock 1) will have prices fluctuations to analyze the size effect as a driver of returns in a portfolio. A rebalancing exercise is implemented for the smart beta portfolio, no trading nor any related cost for implementing the strategy is applied and thus, don’t reflect the full picture as in financial markets.

Table 2 is made of three components. The first section of the table represents our data for the simulation. Each stock has a different size representing respectively a small, mid, and large-capitalization firm. Market capitalization is obtained through a simple computation by multiplying the number of shares times the price of each share. The second section of the table is the simulation of a market-capitalization-weighted portfolio. The third section represents a smart beta portfolio that uses an equally-weighted weighting indexing (Table 2). Note that with the market-capitalization-weighted portfolio there is a concentration in the stock with the largest market capitalization (due to its high past performance). An equally-weighted portfolio obtained with rebalancing (often associated with smart beta strategies such as growth) would not present such property and show a more diversified portfolio over time. Note that the frequency of rebalancing the portfolio can affect the risk/performance characteristics. Amenc et. al. (2016) show that the Sharpe ratio tends to decrease with a higher frequency for rebalancing.

Table 2. Simulation of a market-capitalization-weighted portfolio and an equally-weighted portfolio.
Smart_beta_simulation_spreadsheet
Source: simulations and calculations by the author.

The simulation unveiled that the market-capitalization-weighted portfolio’s size anomaly failed to capture the outperformance of small-cap stocks, resulting in results that were lower than those of the smart beta equally weighted portfolio, which had a good exposure to small caps (Figure 2). The key point of this simulated model is that the market-cap indexation has a defect related to the concentration of large companies in the profile of small caps which represent a small percentage of the index. The size factor is based on a risk factor that aims to capture the documented outperformance of small-cap firms compared to larger enterprises. With this simulated model, we have proven with a very simple model in the conception that the size anomaly can indeed be a vector of return, as researched in the paper of Banz (1981) which precisely describes this concept on the US equity market (Figure 2).

Figure 2. market-capitalization-weighted portfolio vs equally-weighted portfolio.

Source: simulations and calculations by the author.

One aspect to consider in this case analysis is that one of the possible explanations for this outperformance is that the weights are changed at rebalancing dates rather than allowed to drift with the price fluctuations, which is a clear distinction between cap-weighted indexes and smart beta strategies. Some claim that this rebalancing completely explains the success of smart beta strategies (Amenc et al, 2016). This allegation, however, does not hold up under investigation. An examination of buy-and-hold portfolios vs portfolios rebalanced at various frequencies reveals that whether or not rebalancing improves performance is dependent on the return behavior of the assets in the portfolio. Rebalancing may or may not provide better results than buy-and-hold tactics (Amenc et. al., 2016).

Even if beneficial rebalancing impacts occur, Smart Beta methods may not be able to capture them. Contrary to popular belief, data shows that rebalancing an equal-weighted approach more frequently does not always increase performance. Furthermore, both short- and long-term reversal effects are empirically insignificant in explaining the performance of a wide variety of Smart Beta strategies. Naturally, rebalancing is necessary, especially to maintain diversity and target factor exposures. Rebalancing, on the other hand, is not an experimentally verified source of Smart Beta strategy performance (Amenc et. al., 2016).

Smart beta: passive or active investment strategy?

Smart beta investing is considered a hybrid strategy because it attempts to replicate the performance of a predetermined benchmark without engaging in market timing or stock picking, and an active strategy because investors choose to gain exposure to specific factors (beyond the market factor) by rebalancing the portfolio according to some rules. In practice, smart beta strategies often imply rebalancing to maintain target weights for each factor. In this sense, smart beta strategies are active, or at least more active than the buy-and-hold strategy. However, the rebalancing of portfolios of smart beta strategies is usually done with a predefined rule. In this sense, smart beta strategies are passive, or at least more passive than discretionary investment strategies based on stock picking and market timing.

Why should I be interested in this post?

Smart beta funds have become a hot issue among investors in recent years. Smart beta is a game-changing invention (or just a new marketing idea?) that addresses an unmet need among investors: a higher return for lower risk, net of transaction and administrative costs. In a way, these tactics create a new market. As a result, smart beta is gaining traction and influencing the asset management market.

Useful resources

Academic research

Amenc, N., Ducoulombier, F., Goltz, F. and Ulahel, J., 2016. Ten misconceptions about smart beta. EDHEC Risk Institute Working paper.

Banz, R.W., 1981. The relationship between return and market value of common stocks. Journal of Financial Economics, Volume 9, pp. 3-18.

Fama, E.F., French, K.R., 1992. The cross-section of expected stock returns. The Journal of Finance, 47: 427-465.

Grossman, S., Stiglitz, J., 1980. On the impossibility of Informationally efficient markets. The American Economic Review, 70(3), 393-408.

Jensen, M.C. 1968. The performance of mutual funds from 1945–1964. The Journal of Finance, 23:389-416.

Malkiel, B., 1995. Returns from Investing in Equity Mutual Funds 1971 to 1991. The Journal of Finance, 50(2):549-572.

Business analysis

BlackRock Research, 2021. What is Factor Investing?

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Factor Investing

September 12, 2021 by Youssef LOURAOUI

Factor Investing

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents factor investing, which is an investment approach that focuses on distinct performance drivers across asset classes.

This article is structured as follows: we begin with the early works of factor investing (market factor). We then delve more in detail on the different factors available and their characteristics. We finish with an empirical analysis that aims to capture the performance of factor investing across time.

Early works

In the world of investing, a factor is a persistent driver that helps explain assets’ long-term risk and return properties across asset classes. It is important to understand how factors work to better capture their potential for excess return and reduced risk across asset classes.

As a result of Harry Markowitz’s prior studies, William Sharpe, John Lintner, and Jan Mossin independently developed the Capital Asset Pricing Model (CAPM). The CAPM was a significant evolutionary step forward in capital market equilibrium theory because it allowed investors to value assets correctly in terms of systematic risk that impact all assets (Mangram, 2013). In the CAPM, the factor is the market factor representing the global uncertainty of the market.

Factor investing

As defined by Blackrock (2021), “Factor investing” is an investment strategy that focuses on unique determinants of performance across asset classes. Factor investing may improve portfolio performance and decrease volatility by increasing portfolio diversification. Asset returns are driven by two main types of factors: macroeconomic factors and style factors. Macroeconomic factors capture broad risks across asset classes while style factors explain returns and risk within asset classes.

Considering macroeconomic factors, returns can be influenced by the following macroeconomic variables (BlackRock research, 2021):

Economic growth: exposure to business and market cycles
Real interest rates: sensitivity to interest rate movements
Inflation: exposure to change in price
Credit: default risk from lending to companies
Emerging markets: political and sovereign risk
Liquidity: holding liquid assets.

Considering style factors, returns can be influenced by the following style variables (BlackRock research, 2021):

Value: stocks discounted to relative value
Minimum volatility: stable, lower risk stocks
Momentum: stocks with upward price trends
Quality: financially healthy companies
Size: smaller, high growth companies
Growth: companies that have a rate of growth above the market growth
Yield: companies that have undervalued and stable dividends

Characteristics of a factor

As defined in the work of Ang (2013) a factor must comply with the following characteristics:

A factor must be backed up by scholarly research: factors should have an academic basis. The research should illustrate either compelling logical reasoning or compelling behavioral biases, or both, in order to adequately justify the risk premium (Ang, 2013). Value, momentum, and minimum volatility among other strategies qualify as adequate risk factors under this criterion. New research may find new factors, qualify prior agreement on recognized factors, or even reject factors previously identified, all of which may be used to shape investment strategy.
A factor must have maintained a substantial risk premium in the past and is anticipated to do so in the future: not only should investors understand why the risk premium existed in the past, but they should also have some reason to believe that it will continue to exist in the future (at least in the short run). By definition, factors are systematic–they emerge from risk or behavioral patterns that will likely continue (again, in the short run), even if everyone is aware of the factors and many investors pursue the same factor strategies (no crowding effect).
A factor must be capable of being implemented in liquid, tradable instruments: factor strategies should be very inexpensive, which is best done via the use of liquid securities.

Academic literature on factor investing

Numerous academic studies and years of investing experience have revealed some types of stock, debt, and derivative assets with larger payoffs than the broad market index. Over extended periods of time, equities with low price-to-book ratios (value stocks) outperform those with high price-to-book ratios (growth stocks), creating a value-growth premium (Ang, 2013). Over time, equities with a history of high or positive returns (winners) outperform those with a history of low or negative returns (losers). This is at the heart of momentum strategies, which seeks to get exposure to stocks that have a winning tendency in the upside and downside assuming that they will continue to do well in the short term (Ang, 2013).

Investors seeking downside protection in a turbulent market environment may increase exposure to low volatility strategies, while those comfortable with more risk may choose for higher-return strategies such as momentum. The financial literature has explored deeper to show that some factors have had a long-term impact on returns. These factors contributed to returns for three reasons: an investor’s desire to take on risk, structural obstacles, and the reality that not all investors are not always entirely rational (BlackRock research, 2021). Particular factors yield higher returns as a result of increased risk but may underperform in certain market conditions. Enhanced methods use factors in more sophisticated ways, such as trading across various asset classes and sometimes investing in both long and short positions. These improved factor strategies are used by investors seeking absolute returns or as a supplement to hedge funds and classic active strategies (BlackRock research, 2021).

Securities that are less liquid trade at a discount to their more liquid counterparts and earn a higher average excess return on average. As a result, a premium is charged for illiquidity (Ang, 2013). Bonds with a greater risk of default often have higher average returns, owing to the credit risk premium. Additionally, because investors are ready to pay for protection against periods of extreme volatility, when returns tend to fall, sellers of volatility protection in option markets receive a high rate of return on average (Ang, 2013). As a result, investors can collect the premiums as follows (Ang, 2013):

The value-growth premium is equal to the difference between value and growth stocks.
The momentum premium is equal to the difference between winning and losing stocks.
The illiquidity premium is equal to the difference between the value of illiquid assets and the value of liquid assets.
The credit risk premium is the difference between the return on risky and safe debt.

These are dynamic factors, since they reflect time-varying holdings in securities that fluctuate in value over time. While dynamic factors frequently outperform the market over extended periods of time, they can significantly underperform at select occasions — such as the 2008-2009 financial crisis. While dynamic factors frequently outperform the market over extended periods of time, they can outperform the market significantly at select moments — such as the 2008-2009 financial crisis. In the long term, factor risk premiums exist to compensate investors for experiencing losses during difficult times (Ang, 2013). In the end, the factors are not ideal for everyone due to the inherent risk associated with factor techniques.

Empirical analysis

Hodges et al. (2017) published a study in the Journal of Portfolio Management that looks at the performance of factor funds over a 30-year period and examines the vectors of returns). Figure 1 illustrates the average excess returns (above the MSCI USA Index) of each factor from June 30, 1988 to September 30, 2016. Value, quality, momentum, and size all have positive average returns; momentum and value have the largest annual excess returns of 3.4 percent and 1.5 percent, respectively. Minimum volatility has generated an average return comparable to the market (but with less risk), similar with Ang’s findings (Hodges et al., 2017).

Figure 1. Factor analysis from 1988 – 2016. Average excess return.

Source: Hodges et al. (2017).

Figure 2 plots 12-month moving averages of excess factor returns and demonstrates that, while longrun excess premiums are positive, there is significant temporal variation throughout the sample. For instance, size changes from a negative 12-month mean return of -2.0 percent in 1999 to a positive 12-month mean return of 3.0 percent in the early 2000s.

Figure 2. Smart beta excess returns for the period from 1988 to 2016.

Source: Hodges et al. (2017).

Figure 3 demonstrates that the excess factor returns are not substantially correlated: the lowest correlation is -0.42, while the largest is 0.67, between minimal volatility and size. Notably, momentum and value are negatively connected with a correlation coefficient of -0.22, which is consistent with their well-known negative association (Hodges, et al., 2017).

Figure 3. Correlation analysis of smart beta excess returns for the period from 1988 to 2016.

Source: Hodges et al. (2017).

Why should I be interested in this post?

Numerous equity investors seeking greater returns at a cheaper cost have shifted their focus to factor investing. Active managers in the traditional sense typically make investing decisions based on their research of particular companies and their stocks. By contrast, factor strategies identify the qualities, or factors, that are most likely to beat the market and then invest in stocks that exhibit those characteristics. For instance, the value factor is based on the strategy of investing in companies that are undervalued in comparison to the market, whereas the momentum factor is based in the strategy of investing in equities that have recently seen a price acceleration.

Useful resources

Academic research

Ang, A., 2013. Factor Investing. Working paper.

Hodges, P., Hogan, K., Peterson, J. R., Ang, A., 2017. Factor Timing with Cross- Sectional and Time-Series Predictors. The Journal of Portfolio Management 44(1): 30-43.

Business Analysis

BlackRock research, 2021. What is Factor Investing?

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Origin of factor investing

September 12, 2021 by Youssef LOURAOUI

Origin of factor investing

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the origin of factor investing. A factor is defined as a persistent driver that helps explain assets’ long-term risk and return properties across asset classes.

This article is structured as follows: we begin by presenting Markowitz’s Modern Portfolio Theory (MPT) as the origin of factor investing (market factor). We then explain the Fama-French three-factor models, which is an extension of the CAPM single factor model (market factor). Furthermore, we explain also the Carhart four-factor model and the Fama-French five-factor model that aimed to capture additional factors to the market factor.

Markowitz’s Modern Portfolio Theory: Origin of the factor investing

Factor investing can be retraced to the work of Harry Markowitz in the early 1950s. The most important aspect of Markowitz’s approach was his fundamental finding that an asset’s risk and return should not be evaluated on its own, but rather on how it contributes to the entire risk and return of a portfolio. His dissertation, titled “Portfolio Selection”, was published in The Journal of Finance (1952). Nearly thirty years later, Markowitz shared the Nobel Prize for economics and corporate finance for his MPT contributions to both disciplines. The holy grail of Markowitz’s work is based on his calculation of the variance of a two-asset portfolio computed as follows:

Where:

w and (1-w) represents asset weights of assets A and B
σ² represents the variance of the assets and portfolio
cov(r_A,r_B) represents the covariance of assets A and B.

Capital Asset Pricing Model (CAPM)

William Sharpe, John Lintner, and Jan Mossin separately developed another key capital markets theory as a result of Markowitz’s previous works : the Capital Asset Pricing Model (CAPM). The CAPM was a huge evolutionary step forward in capital market equilibrium theory, since it enabled investors to appropriately value assets in terms of systematic risk, defined as the market risk which cannot be neutralized by the effect of diversification. In his derivation of the CAPM, Sharpe, Mossin and Litner made significant contributions to the concepts of the Efficient Frontier and Capital Market Line. Sharpe, Litner and Mossin seminal contributions would later earn him the Nobel Prize in Economics. The CAPM is based on a set of market structure and investor hypotheses:

There are no intermediaries
There are no limits (short selling is possible)
Supply and demand are in balance
There are no transaction costs
An investor’s portfolio value is maximized by maximizing the mean associated with projected returns while reducing risk variance
Investors have simultaneous access to information in order to implement their investment plans
Investors are seen as “rational” and “risk averse”.

Under this framework, the expected return of a given asset is related to its risk measured by the beta:

Where :

E(r) represents the expected return of the asset
r_f the risk-free rate
β a measure of the risk of the asset
E(r_m) the expected return of the market
E[r_m– r_f]represents the market risk premium.

In this model, the beta (β) parameter is a key parameter and is defined as:

Where:

Cov(r, r_m) represents the covariance of the asset with the market
σ²(r_m) is the variance of market return.

According to the CAPM formula, beta may be thought in mathematical terms as the slope of the regression between the asset return and the market return. Thus, beta quantifies the asset sensitivity to changes in the market return;
According to the beta formula, it may be understood as the risk that each dollar invested in an asset adds to the market portfolio. This is an economic explanation based on the observation that the market portfolio’s risk (measured by 〖σ(r_m)〗^2) is a weighted average of the covariance risks associated with the assets in the market portfolio, making beta a measure of the covariance risk associated with an asset in comparison to the variance of the market return.

Additionally, the CAPM makes a distinction between two forms of risk: systematic and specific risk. Systematic risk refers to the risk posed by the market’s basic structure, its participants, and any and all non-diversifiable elements such as monetary policy, political events, and natural disasters. By contrast, specific risk refers to the risk inherent in a particular asset and so is diversifiable. As a result, the CAPM solely captures systematic risk via the beta measure, with the market’s beta equal to one, lower-risk assets having a beta less than one, and higher-risk assets having a beta larger than one.

Finally, the CAPM’s central message is that when investors invest in a particular security/portfolio, they are rewarded twice: once via the time value of money impact (reflected in the risk-free component of the CAPM equation) and once via the effect of taking on more risk. However, the CAPM is not an empirically sound model, owing to an unnecessarily simplified set of assumptions and problems in establishing validating tests at the model’s first introduction (Fama and French, 2004). Thus, throughout time, the CAPM has been revised and modified to address not just its inadequacies but also to keep pace with financial and economic changes. Sharpe (1990), in his evaluation of the CAPM, cites various examples of revisions to his basic model proposed by other economists and financial experts.

The Fama-French three-factor model

Eugene Fama and Kenneth French created the Fama-French Three-Factor model in 1993 in response to the CAPM’s inadequacy. It contends that, in addition to the market risk component introduced by the CAPM, two more factors affect the returns on securities and portfolios: market capitalization (referred to as the “size” factor) and the book-to-market ratio (referred to as the “value” factor). According to Fama and French, the primary rationale for include these characteristics is because both size and book-to-market (BtM) ratios are related to the economic fundamentals of the business issuing the securities (Fama and French, 1993).

They continue by stating that:

Earnings and book-to-market ratios are inversely associated, with companies with low book-to-market ratios consistently reporting better earnings than those with high book-to-market ratios
Due to a similar risk component, size and average returns are inversely associated. This is based on their observation of the trajectory of small business profits in the 1980s: they suggest that small enterprises experience longer durations of earnings depression than larger enterprises in the event of a recession in the economy in which they operate. Additionally, they noted that smaller enterprises did not contribute to the economic expansion in the mid- and late-1980s following the 1982 recession
Profitability is connected to both size and BtM, and is a common risk factor that emphasizes and explains the positive association between BtM ratios and average returns. As thus, the return on a security/portfolio becomes:

Where :

E(𝑟) is the expected return of the asset/portfolio
𝑟_𝑓 is the risk-free rate
𝛽 is the measure of the market risk of the asset
𝐸(𝑟_𝑀) is the expected return of the market
𝛽_𝑆 is the measure of the risk related to the size of the asset
𝛽_𝑉 is the measure of the risk related to the value of the security/portfolio
𝑆𝑀𝐵 (which stands for “Small Minus Big”) measures the difference in expected returns between small and big firms (in terms of market capitalization)
𝐻𝑀𝐿 (which stands for “High Minus Low”) measures the difference in expected returns between value stocks and growth stock
𝛼 is a regression intercept
𝜖 is a measure of regression error

Both SMB and HML are derived using historical data as well as a mixture of portfolios focused on size and value. Professor French publishes these values on a regular basis on his personal website. Meanwhile, the betas for both the size and value components are derived using linear regression and might be positive or negative. However, the Fama-French three-factor model is not without flaws. Griffin (2002) highlights a significant flaw in the model when he claims that the Fama-French components of value and size are more accurate at explaining return differences when applied locally rather than internationally. As a result, each of the components should be addressed on a nation-by-country basis (as professor French now does on his website, where he specifies the SMB and HML factors for each nation, such as the United Kingdom, France, and so on). While the Fama-French model has gone further than the CAPM in terms of breaking down security returns, it remains an incomplete model with spatially confined interpretation of its additional variables. Efforts have been made over the years to complete this model, with Fama and French adding two more variables in 2015, profitability and investment strategy, and other scholars, like as Carhart (1997), adding a fourth feature, momentum, to the original Three-Factor model.

The Carhart four-factor model

Carhart (1997) extended the Fama-French three-factor model (1993) by adding a fourth factor: momentum. Momentum is defined as the observable tendency for prices to continue climbing or declining following an initial increase or decline. By definition, momentum is an anomaly, as the Efficient Market Hypothesis (EMH) states that there is no reason for security prices to continue growing or declining after an initial change in their value.

While traditional financial theory is unable to define precisely what causes momentum in certain securities, behavioural finance provides some insight into why momentum exists; indeed, Chan, Jegadeesh and Lakonishok (1996) argue that momentum arises from the inability of the majority of investors to react quickly and immediately to new market information and, thus, integrate that information into securities. This argument demonstrates investors’ irrationality when it comes to appraising the value of certain stocks and making investing decisions. Carhart was motivated to incorporate the momentum component into the Fama-French three-factor model since the model was unable to account for return variance in momentum-sorted portfolios (Fama and French, 1996 – Carhart 1997). Carhart incorporated Jegadeesh and Titman’s (1993) one-year momentum variation into his model as a result.

Where the additional component represents:

𝛽_𝑀 is the measure of the risk related to the momentum factor of the security/portfolio
𝑈𝑀𝐷 (which stands for “Up Minus Down”) measures the difference in expected returns between “winning” securities and “losing” securities (in terms of momentum).

As Carhart states in his article, the four-factor model, like the CAPM and the Fama-French Three-Factor, may be used to explain the sources of return on a specific security/portfolio (Carhart, 1997).

The Fama-French five-factor model

Fama and French state in 2014 that the first three-factor model they developed in 1993 does not adequately account for certain observed inconsistencies in predicted returns. As a consequence, Fama and French enhanced the three-factor model by adding two new variables: profitability and investment. The justification for these two factors arises from the theoretical implications of the dividend discount model (DDM), which claims that profitability and investment help to explain the returns achieved from the HML element in the first model (Fama and French, 2015).

Surprisingly, unlike the Carhart model, the new Fama-French model does not incorporate the momentum element. This is mostly because to Fama’s position on momentum. While not denying its existence, Fama thinks that the degree of risk borne by securities in an efficient market cannot fluctuate so dramatically that it justifies the necessity to recognize the momentum factor’s involvement (Fama and French, 2015). According to the Fama-French five-factor model, the return on any security is calculated as follows:

𝛽_P is the measure of the risk related to the profitability factor of the security/portfolio
𝑅𝑀𝑊 (which stands for “Robust Minus Weak”) measures the difference in expected returns between securities that exhibit strong profitability levels (thus making them “robust”) and securities that show inconsistent profitability levels (thus making them “weak”)
𝛽_𝐼 is the measure of the risk related to the investment factor of the asset
𝐶𝑀𝐴 (which stands for “Conservative Minus Aggressive”) measures the difference in expected returns between securities that engage in limited investment activities (thus making them “conservative”) and securities that show high levels of investment activity (thus making them “aggressive”).

To validate the new model, Fama and French created many portfolios with considerable returns disparities due to size, value, profitability, and investing characteristics. Additionally, they completed two exercises:

The first is a regression of portfolio results versus the improved model. This was done to determine the extent to which it explains the observed returns disparities between the selected portfolios
The second is to compare the new model’s performance to that of the three-factor model. This was done to determine if the new five-factor model adequately accounts for the observed returns differences in the old three-factor model. The following summarizes Fama and French’s conclusions about the new model.

The HML component becomes superfluous in terms of structure, since any value contribution to a security’s return can already be accounted by market, size, investment, and profitability factors. Thus, Fama and French advise investors and scholars to disregard the HML effect if their primary objective is to explain extraordinary returns (Fama and French, 2015).

They do, however, argue for the inclusion of all five elements when attempting to explain portfolio returns that display size, value, profitability, and investment tilts. Additionally, the model explains between 69% and 93% of the return disparities seen following the usage of the prior three-factor model (Fama and French, 2015). This new model, however, is not without flaws. Blitz, Hanauer, Vidojevic, and van Vliet (henceforth referred to as BHVV) identified five problems with the new Fama-French five-factor model in their 2016 paper “Five difficulties with the Five-Factor model”.

While two of these issues are related to some of the original Fama-French three factor model’s original factors (most notably the continued existence within the model of the CAPM relationship between market risk and return, as well as the new model’s overall acceptance by the academic community while some of the original factors are still contested), several of the other issues are related to other factors. These concerns include the following (Fama and French, 2015) :

The lack of motion
The new factors introduced lack robustness. The questions here include historical (i.e., will these factors apply to data points before to 1963) and if these aspects also apply to other asset types
The absence of adequate empirical support for the implementation of these Fama and French components

Use of the asset pricing models

All the models presented above are mostly employed in asset management to analyze the performance of an actively managed portfolio and the overall performance of a mutual fund.

Why should I be interested in this post?

Useful resources

Academic research

Blitz, D., Hanauer M.X., Vidojevic M., van Vliet, P., 2018. Five Concerns with the Five-Factor Model, The Journal of Portfolio Management, 44(4): 71-78.

Carhart, M.M. (1997), On Persistence in Mutual Fund Performance. The Journal of Finance, 52: 57-82.

Fama, E.F., French, K.R., 1992. The Cross-Section of Expected Stock Returns. The Journal of Finance, 47: 427-465.

Fama, E.F., French, K.R., 2004. The Capital Asset Pricing Model: Theory and Evidence. Journal of Economic Perspectives, 18(3): 25-46.

Fama, E.F., French, K.R., 2015. A five-factor asset pricing model. Journal of Financial Economics, 116(1): 1-22.

Lintner, J. 1965a. The Valuation of Risk Assets and the Selection of Risky Investments in Stock Portfolios and Capital Budgets. The Review of Economics and Statistics 47(1): 13-37.

Lintner, J. 1965b. Security Prices, Risk and Maximal Gains from Diversification. The Journal of Finance 20(4): 587-615.

Mangram, M.E., 2013. A simplified perspective of the Markowitz Portfolio Theory. Global Journal of Business Research, 7(1): 59-70.

Markowitz, H., 1952. Portfolio Selection. The Journal of Finance, 7(1): 77-91.

Mossin, J. 1966. Equilibrium in a Capital Asset Market. Econometrica 34(4): 768-783.

Sharpe, W.F. 1963. A Simplified Model for Portfolio Analysis. Management Science 9(2): 277-293.

Sharpe, W.F. 1964. Capital Asset Prices: A theory of Market Equilibrium under Conditions of Risk. The Journal of Finance 19(3): 425-442.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Growth Factor

September 5, 2021 by Youssef LOURAOUI

Growth Factor

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the growth factor, which is based on a risk factor that aims to get exposure to firms with high growth potential based on a variety of parameters such as historical profits, sales, and expected earnings.

This article is structured as follows: we begin by defining the growth factor and reviewing academic studies. The MSCI Growth Factor Index, which is well used as a benchmark in the asset management industry, is next presented in terms of performance and risk-return trade-off. We showcase the ETF market for investors looking to profit from the growth factor.

Definition

Eugene Fama and Kenneth French, following the CAPM’s original work, developed the Fama-French Three-Factor model in 1993 to solve the CAPM’s inadequacies. It claims that, in addition to the market risk component of the CAPM, two other factors have an effect on the returns on securities and portfolios: market capitalization (called the “size” factor) and the book-to-market ratio (referred to as the “value” factor). Other factor characteristics were developed to capture some additional performance as financial research advanced and significant contributions were made.

Academic research

The fundamental work of Fama and French may be traced back to the most significant academic works in the factor investing literature. Since the growth factor has a poor academic literature review, we will focus on the work of Fama and French (1993). In response to the CAPM’s limitations, Eugene Fama and Kenneth French developed the Fama-French three-factor model in 1993. It argues that, in addition to the market risk component provided by the CAPM, two additional factors, market capitalization (referred as “size”) and book-to-market ratio (referred as “value”), influence the returns on securities and portfolios. The major rationale for including these attributes, according to Fama and French, is that both size and book-to-market ratios are connected to the economic fundamentals of the firm issuing the securities (Fama and French, 1993).

In 2014, Fama and French claimed that their original three-factor model from 1993 was insufficient to explain certain observed differences in expected returns. As a result, Fama and French expanded their three-factor model to include two more factors: profitability and investment. The theoretical implications of the dividend discount model (DDM), which claim that profitability and investment contribute to the explanation of the returns derived from the High Minus Low premium element in the first model, justify these two aspects (Fama and French, 2015). High Minus Low can be defined as the value premium that accounts for the spread between the return of small capitalization stocks compared to large capitalization stocks.

Active managers have utilized the Growth factor to capture corporate growth possibilities using historical profits, sales, and anticipated earnings, and it has been employed as a possible source of alpha. The impact of unintended exposure, which shows that assets with strong growth can also have high valuations, high volatility, low yield, and bad quality, which can negatively influence portfolio performance, can be a difficulty when using simple selection methods to capture growth (MSCI Factor research, 2021).

Growth investing, often known as capital growth or capital appreciation, has been a prominent investment strategy since the 1950s and is one of active managers’ most intuitive and commonly used investment strategies (MSCI Factor research, 2021). Growth is a well-known investment strategy that, according to risk models, has a strong explanatory power in risk forecasting. In comparison to the MSCI ACWI Index, the pure growth factor has shown an impressive long-term return as well as low or negative correlation with other factors, which may assist diversify a multi-factor portfolio by minimizing short-term cyclicality.

Example of a “growth” stock

Any stock in a firm that is expected to expand at a pace significantly higher than the market average is considered a growth stock. Dividends are seldom paid on these stocks. This is because growth stock issuers are often businesses that seek to reinvest any profits in order to increase growth in the short term. When people buy growth stocks, they expect to profit from capital gains when they sell them later (Investopedia, 2021).

For instance, Amazon Inc. (AMZN) has been regarded as a growth stock for quite some time. It is, and has been for some time, one of the world’s largest companies in 2020. In terms of market value as of July 31, 2021, Amazon is among the top five U.S. stocks.

MSCI Growth Factor Index

MSCI Growth Factor Index accounts for unexpected risks and exposures while also extending the notion of growth at a reasonable price (GARP) to include volatility, yield, and quality (MSCI Factor research, 2021). The impact of unintended exposure, which shows that assets with strong growth can also have high valuations, high volatility, low yield, and bad quality, which can negatively influence portfolio performance, can be a difficulty when using simple selection methods to capture growth. MSCI’s growth target index accounts for unexpected risks and exposures while also extending the notion of growth at a reasonable price (GARP) to include volatility, yield, and quality. Growth at a reasonable price (GARP), a long-held notion among growth investors, aims to avoid overpaying for a stock’s prospective growth. The GARP idea may be expanded by limiting value exposure, ensuring that the long-term premium for growth is not reduced by the unintentional and accidental impact of assets with high values, i.e., negative value exposure.

Performance of the MSCI Growth Factor Index

Figure 1 compares the MSCI Growth Factor Index’s performance to those of other factors from May 1999 to May 2020. All indices are rebalanced on a 100-point scale to ensure consistency in performance and to facilitate factor comparisons.

Figure 1. Performance of the MSCI Growth Factor Index from 1999-2020.
Growth factor performance
Source: MSCI Factor research, 2021.

Over the long run, the MSCI World Growth Index has traditionally delivered excess returns, with a yearly return of 1.41 percent over the MSCI World Index since 1999, as seen above. (MSCI Factor research, 2021).

Risk-return profile of MSCI Growth Factor Index

Figure 2 shows the MSCI Growth Factor Index compared to other factors over the period May 1999 – May 2020 in terms of risk/reward. The risk-return trade-off states that the potential return rises with an increase in risk. Individuals connect low levels of uncertainty about future returns with low potential returns, while high levels of uncertainty or risk are associated with large potential returns. According to the risk-return trade-off, an investor’s money can generate higher returns only if the investor is willing to endure a higher risk of loss and return (Figure 2).

Figure 2. Risk-return profile of MSCI Growth Factor Index compared to a peer group.
Growth factor risk return
Source: MSCI Factor research, 2021.

Growth stocks are defined as firms that are projected to expand their sales, profits, or margins faster than the industry or market average. The growth factor may provide value to a multi-factor portfolio by mitigating short-term cyclicality and providing asset managers with diversity and a stable source of premia. MSCI developed the Growth Target Index, based on Barra’s equity index model characteristics, through an optimization process that captures the growth component while limiting unwanted exposures that might erode the growth premium (MSCI Factor research, 2021).

ETFs for the growth factor

Let us recall that an Exchange-Traded Fund (ETF) is an investment vehicle that seeks to mirror the performance of a benchmark like an equity index and is traded on a continuous basis during the day like stocks. By investing in ETFs, an investor gains access to a plethora of diversification options through several asset classes (equity, bonds, currency, commodity, real estate, etc.).

In terms of proportion of assets under management, Figure 3 depicts the total ETF distribution among the leading suppliers of growth factor ETFs. Despite the lack of a real monopoly, the market is more equally distributed.

It’s worth mentioning the ARK Innovation ETF, which accounts for almost a third of the entire growth ETF market that was nominated. This ETF invests on biotech, robotics, artificial intelligence, blockchain, and finance technology, among other areas. It’s a thematically focused fund that invests in a limited number of high-growth companies and makes large swings in them.

The fund’s top 10 holdings make up nearly half of the overall portfolio. The company’s largest investment is Tesla (TSLA), which accounts for about 11% of its assets, followed by Square (SQ), Teladoc Health (TDOC), and Roku (ROKU), which account for 6.5 percent, 6.3 percent, and 5.5 percent, respectively. The top 10 companies include Zillow Group (Z), Zoom Video Communications (ZM), Baidu (BIDU), Shopify (SHOP), Spotify Technology (SPOT), and Exact Sciences (EXAS). The ARK Innovation ETF (ticker: ARKK) had a 153 percent return in 2020 (etf.com, 2021).

Figure 3. Growth factor ETF market.
Growth factor market share
Source: etf.com (2021).

Table 1 gives more detailed information about the biggest growth factor ETF providers: the asset under management (AUM), expense ratio (ER) and the segment for the investments.

Table 1. Ranking of the biggest Growth ETF providers.
Growth factor actors
Source: etf.com (2021).

Why should I be interested in this post?

If you are an undergraduate or graduate student in a business school or at the university, you may have seen in your 101 finance course the CAPM related to the market factor. This post makes aware of the existence of another risk factor priced by the market.

If you are an investor, you may consider adding an exposure to growth factor to enhance the overall portfolio return.

Useful resources

Academic research

Fama, E.F., French, K.R. 1992. The Cross-Section of Expected Stock Returns. The Journal of Finance, 47: 427-465.

Fama, E.F., French, K.R., A five-factor asset pricing model, Journal of Financial Economics, 116(1): 2015, 1-22.

Mangram, M.E., 2013. A simplified perspective of the Markowitz Portfolio Theory. Global Journal of Business Research, 7(1): 59-70.

Business analysis

etf.com, 2021. Biggest Growth ETF providers.

MSCI Investment Research, 2021. Factor Focus: Growth.

Investopedia, 2021. Growth Stock.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Quality Factor

September 5, 2021 by Youssef LOURAOUI

Quality Factor

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the quality factor, which is based on a risk factor that aims to get exposure to businesses with long-term business plans and competitive advantages.

This article is structured as follows: we begin by defining the quality factor and reviewing academic studies. The MSCI Quality Factor Index, which is well used as a benchmark in the asset management industry, is next presented in terms of performance and risk-return trade-off. We showcase the ETF market for investors looking to profit from the quality factor.

Definition

In the world of investing, a factor is any characteristic that helps explain an asset’s long-term risk and return performance. In the late 1970s, the portfolio management industry’s objective was to capture the market return on a portfolio. As a result of Markowitz and Tobin’s earlier research, William Sharpe, John Lintner, and Jan Mossin independently developed the Capital Asset Pricing Model (CAPM). Because it enabled investors to properly value assets in terms of systematic risk, the CAPM was a significant evolutionary step forward in the theory of capital market equilibrium (Mangram, 2013).

The quality factor is based on a risk factor that aims to get exposure to businesses with long-term business plans and competitive advantages. It can also be defined as the attributes for which investors are prepared to pay a premium (Hsu et al., 2019).

Academic research

The long-term outperformance of the quality factor over the market is well documented in the financial literature. Eugene Fama and Kenneth French added two quality-related components to their distinctive three-factor model (firm size, business value, and market risk): profitability and asset growth. Numerous active strategies have prioritized quality growth in their premium selection and portfolio construction processes. In 2012, Robert Novy-Marx published an essay proving that profitability and stability were just as useful as traditional value measures for assessing returns (MSCI Factor research, 2021).

Asness et al. (2018) propose a valuation model that illustrates how stock prices should increase if qualitative qualities such as profitability, growth, and safety improve. They demonstrate experimentally that high-quality stocks do fetch a premium on average, but not by a huge margin (Asness et al., 2018). Perhaps as a result of this perplexingly little influence of quality on price, high-quality stocks provide appealing risk-adjusted returns. Indeed, in the United States and 24 other countries, a factor that invests in high-quality companies and shorts low-quality companies generates significant risk-adjusted returns. The price of quality fluctuates throughout time, reaching a low point during the internet bubble, and a low price of quality suggests that QMJ will give a high rate of return in the future. Analysts’ price targets and earnings predictions indicate that systemic errors in return and earnings expectations are occurring as a result of quality issues (Asness et al., 2018).

MSCI Quality Factor Index

MSCI Factor Indexes are rule-based, transparent indexes that target equities with favorable factor qualities, as determined by academic discoveries and empirical outcomes, and are designed for easy implementation, replicability, and usage in both standard indexed and active portfolios. The MSCI Quality Factor Index measures the quality factor using three fundamental variables (MSCI Factor research, 2021) :

Return on equity – a measure of a company in generating profits
Debt to equity – a measure of a company’s leverage
Earnings variability – a measure of how smooth earnings growth has been.

Quality is a “defensive” component, which means that it has historically benefited during periods of economic recession (MSCI Factor research, 2021). The quality factor has aided in explaining the performance of equities with low debt, steady profits, and a high profit margin.

Performance of the MSCI Quality Factor Index from

Figure 1 compares the MSCI Quality Factor Index’s performance to those of other factors from May 1999 to May 2020. All indices are rebalanced on a 100-point scale to ensure consistency in performance and to facilitate factor comparisons

Figure 1. Performance of the MSCI Quality Factor Index from 1999-2020.

Source: MSCI Factor research (2021).

The MSCI Quality Factor Index has traditionally outperformed the MSCI World Index in the long term, with a 1.98 percent annual return over the MSCI World Index since 1999, as seen below (MSCI Factor research, 2021).

Risk-return profile of MSCI Quality Factor Index

Figure 2 shows the MSCI Quality Factor Index compared to other factors over the period May 1999 – May 2020 in terms of risk/reward. The risk-return trade-off states that the potential return rises with an increase in risk. Individuals connect low levels of uncertainty about future returns with low potential returns, while high levels of uncertainty or risk are associated with large potential returns. According to the risk-return trade-off, an investor’s money can generate higher returns only if the investor is willing to endure a higher risk of loss as shown in Figure 2.

Figure 2. Risk-return profile of MSCI Yield Factor Index compared to a peer group.

Source: MSCI Factor research (2021).

Behavior of the MSCI Quality Factor Index during the Covid-19 crisis

The Covid-19 crisis has not only caused significant social and economic suffering, but it had also an impact on financial markets. To study the behavior of the factors during the Covid-19 crisis, we compute the return of the MSCI Factor indexes during the different stages of the crisis. The MSCI Factor indexes are: value, size, quality, momentum and minimum volatility. Following Pagano et al. (2020) and Hasaj and Sherer (2021), we decompose the Covid-19 crisis into five stages: origin, incubation, outbreak, fever, and treatment. Each stage is described below.

Origin (01/11/2019 – 01/01/2020): the first instances are reported in Wuhan, China.
Incubation (02/01/2020 – 17/01/2020): during this phase, the number of patients began to rise at a faster rate, raising concerns about the disease’s severity.
Outbreak (20/01/2020 – 21/02/2020): the number of cases rose to the point that the World Health Organization (WHO) decided that this illness may pose a major threat to the world’s population, and the pandemic was proclaimed.
Fever (24/02/2020 – 20/03/2020): markets are extremely volatile, owing to government restrictions aimed at flattening the infection curve, with the decision to impose a lockdown in numerous nations as the most notable measures, among others.
Treatment (23/03/2020 – 15/04/2020): most of this turnaround occurs between March and June 2020, which corresponds with the start of good news about the discovery and widespread use of the vaccine.

Table 1 gives the performance of the MSCI factor indexes during the different stages of the Covid-19 crisis. Performance is measured by the return computed on the time-period of each stage, and then annualized for comparison across the different stages. We use data are from Thomson Reuters.

Table 1. Performance of the MSCI factor indexes during the Covid-19 crisis.

Performance_MSCI_Factor_Indexes_COVID-19_Crisis

Source: computation by the author. Data source: Thomson Reuters.

A conclusive statement can be made based on our analysis. The quality component was the strongest performer throughout the COVID crisis’s inception in late 2020 and during the fever phase, when severe limitations were implemented, resulting in a collapsing market.

ETFs to capture the Quality factor

Figure 3 illustrates the overall ETF distribution of the major providers of quality factor ETFs in terms of percentage of asset under management. By examining the market overview for quality factor investments, we can observe SPDR dominance in this factor investing market, with 76.07%, representing more than three quarters of the overall quality factor ETF market.

Figure 3. Quality factor ETF market.

Source: etf.com (2021).

Table 2 gives more detailed information about the biggest quality factor ETF providers: the asset under management (AUM), expense ratio (ER) and the segment for the investments.

Table 2. Ranking of the biggest Quality ETF providers.

Source: etf.com (2021).

Why should I be interested in this post?

If you are an undergraduate or graduate student at a business school or university, you may have encountered the CAPM in your 101 finance course. This post raises awareness of the presence of another market-priced risk factor.

If you are an investor, you may wish to consider increasing your exposure to the quality factor in order to boost your portfolio’s total return.

Useful resources

Academic research

Clifford S. Asness & Andrea Frazzini & Lasse Heje Pedersen, 2019. “Quality minus junk,” Review of Accounting Studies, 24(1): 34-112.

Hasaj, M., Sherer, B., 2021. Covid-19 and Smart-Beta: A Case Study on the Role of Sectors. EDHEC-Risk Institute Working paper, 1-35.

Mangram, M.E., 2013. A simplified perspective of the Markowitz Portfolio Theory. Global Journal of Business Research, 7(1): 59-70.

Pagano, M., Wagner, C., Zechner, J., 2020. Disaster Resilience and Asset Prices, Working paper.

Business analysis

etf.com, 2021. Biggest Quality ETF providers.

MSCI Investment Research, 2021. Factor Focus: Quality.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Size Factor

April 4, 2024September 5, 2021 by Youssef LOURAOUI

Size Factor

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the size factor, which is based on a risk factor that aims to capture the documented outperformance of small-cap firms compared to larger enterprises.

This article is structured as follows: we begin by defining the size factor and reviewing academic studies. The MSCI Size Factor Index, which is well used as a benchmark in the asset management industry, is next presented in terms of performance, risk-return trade-off, and behavior during the Covid-19 crisis. We showcase the ETF market for investors looking to profit from the size factor.

Definition

The Size factor has captured the long-run proclivity of small-cap firms to outperform larger enterprises. The work of Banz (1981) adds another piece to the growing puzzle. It evaluates the link between a firm’s overall market value and its return on common shares. The findings indicate that, on average, small businesses common stock generated greater risk-adjusted returns than large firms’ common stock throughout the 1936 – 1975 period (Banz, 1981). This impact is referred to as the “size effect”.

Academic research

Rolf Banz, a Ph.D. candidate at the University of Chicago at that time, found the size factor in US stocks in 1981. The size effect’s proponents provide many explanations for it. Banz stated that it is the result of a weakness in the capital asset pricing model (CAPM, the typical approach for forecasting risk and return on stock investments) or a lack of information regarding businesses that receive minimal analyst attention. After economists Eugene Fama and Kenneth French incorporated size as a critical component of their renowned three-factor model, size research exploded (MSCI Factor research, 2021).

Empirical studies

According to academic literature, the single-period capital asset pricing model (henceforth CAPM) postulates a straightforward linear connection between a security’s projected return and market risk. While direct testing has proved inconclusive, emerging evidence supports the possibility of other asset price variables.

For the period 1936-1977, Litzenberger and Ramaswamy (1979) demonstrate a substantial positive association between dividend yield and return on common stocks. Basu (1977) establishes a link between price-earnings ratios and risk-adjusted returns (Banz, 1981). He interprets his findings as evidence of market inefficiency; however, market efficiency tests are frequently conducted in conjunction with tests of the efficient market hypothesis and a particular equilibrium connection. Thus, some of the abnormalities ascribed to a lack of market efficiency may easily be the consequence of model misspecification. However, because the study’s findings are not based on a particular theoretical equilibrium model, it is impossible to clearly establish whether market value matters in and of itself or whether it is only a proxy for undiscovered actual extra elements linked with market value (Banz, 1981).

According to the data given in this paper, the CAPM is misspecified. Over a forty-year period, tiny NYSE businesses have generated considerably higher risk-adjusted returns than large NYSE enterprises (Banz, 1981). This size impact is not linear in market proportion (or market proportion log) but is most evident for the sample’s smallest companies. Additionally, the impact is not very stable over time. A comparison of the ten-year subperiods reveals significant variations in the magnitude of the size factor’s coefficient (Banz, 1981).

Such an impact has no theoretical basis. Banz asserts that we don’t even know if the factor is size itself or if size is only a proxy for one or more genuine but unknown factors that are linked with size (Banz, 1981). However, it is feasible to make certain hypotheses and even debate some aspects for which size is a proxy. Reinganum’s (1980) recent study has ruled out one obvious candidate: the price-earnings (P/E) ratios. He discovers that the P/E effect, as reported by Basu (1977), vanishes when he controls for size for both NYSE and AMEX stocks, but that there is a significant size effect even when he controls for the P/E ratio, implying that the P/E ratio effect is a proxy for the size effect and not the other way around (Banz, 1981).

Naturally, there are still a vast number of potential elements to evaluate. Thus, a lack of knowledge about small businesses results in less diversification and thus greater returns on ‘undesirable’ small business stocks (Banz, 1981). It may be tempting to use the size effect as the basis for a theory of mergers – big businesses may pay a premium for small firms’ shares because they can discount the same cash flows at a lower discount rate. Naturally, this may turn out to be total nonsense if it is demonstrated that size is only a proxy. While this informal model fits the empirical data, it is only speculation. The size effect occurs, but its cause is unknown. It should be regarded with caution until an answer is found (Banz, 1981).

MSCI Size Factor Index

MSCI Factor Indexes are rules-based, transparent indexes that target equities with favorable factor qualities, as determined by academic discoveries and empirical outcomes, and are designed for easy implementation, replicability, and usage in both standard indexed and active portfolios. The MSCI Equal Weighted Indexes tend to favor smaller cap firms. At each rebalance date, index components are weighted equally, thereby eliminating the influence of that constituent’s price (high or low) from the index. Size is a “pro-cyclical” element, which means it has historically benefited from periods of economic boom.

For decades, institutional investing has included a size premium. It has been a key component of several factor-based indexes during the last few years. MSCI Equal Weighted Indexes tend to favor smaller sized firms in comparison to the benchmark parent index (MSCI Factor research, 2021). At each rebalancing date, index components are weighted equally, thereby eliminating the influence of a constituent’s price (high or low) on the index.

Performance of the MSCI Size Factor Index

Figure 1 compares MSCI World Equal Weighted Index (Size factor) performance to those of other factors from May 1999 to May 2020. All indices are rebalanced on a 100-point scale to ensure consistency in performance and to facilitate factor comparisons.

Figure 1. Performance of the MSCI Size Factor Index from 1999-2020.

Source: MSCI Factor research (2021).

Over the long term, the MSCI World Equal Weighted Index (Size factor) has traditionally provided excess returns, with an annual return of 1.54 percent over the MSCI World Index since 1999 (MSCI Factor research, 2021).

Risk-return profile of MSCI Size Factor

Figure 2 shows the MSCI World Equal Weighted Index (Size factor) compared to other factors over the period May 1999 – May 2020 in terms of risk/reward. The risk-return trade-off states that the potential return rises with an increase in risk. Individuals connect low levels of uncertainty about future returns with low potential returns, while high levels of uncertainty or risk are associated with large potential returns. According to the risk-return trade-off, an investor’s money can generate higher returns only if the investor is willing to endure a higher risk (Figure 2).

Figure 2. Risk-return profile of MSCI Size Factor Index compared to a peer group.

Source: MSCI Factor research (2021).

Behavior of the MSCI Size Factor Index during the Covid-19 crisis

Origin (01/11/2019 – 01/01/2020): the first instances are reported in Wuhan, China.
Incubation (02/01/2020 – 17/01/2020): during this phase, the number of patients began to rise at a faster rate, raising concerns about the disease’s severity.
Outbreak (20/01/2020 – 21/02/2020): the number of cases rose to the point that the World Health Organization (WHO) decided that this illness may pose a major threat to the world’s population, and the pandemic was proclaimed.
Fever (24/02/2020 – 20/03/2020): markets are extremely volatile, owing to government restrictions aimed at flattening the infection curve, with the decision to impose a lockdown in numerous nations as the most notable measures, among others.
Treatment (23/03/2020 – 15/04/2020): most of this turnaround occurs between March and June 2020, which corresponds with the start of good news about the discovery and widespread use of the vaccine.

Table 1. Performance of the MSCI factor indexes during the Covid-19 crisis.

Source: computation by the author. Data source: Thomson Reuters.

According to an examination of more than one year worth of market data, the size factor underperformed throughout the study period, most notably during the period of economic stress in the financial markets caused by the Covid-19 crisis. Given the crisis’s unprecedented severity, lockdown essentially shut down small and medium-sized firms, which finally suffered a period of catastrophic financial hardship, culminating in a non-negligible number of chain bankruptcies in the hardest-hit industries. This may help to explain why the Fever phase is the lowest-returning for the size factor. As the crisis progressed and governments spent billions on an accommodating monetary strategy to stimulate demand and re-establish healthy growth, size outperformed in the time after the pandemic’s fever phase (Figure 3).

ETFs to capture the Size factor

Figure 3 illustrates the overall ETF distribution of the major providers of size factor ETFs in terms of percentage of asset under management. By examining the market overview for size factor investments, we can observe Blackrock and Vanguard dominance in this factor investing market, with 53.40% and 37.27% respectively, representing 90.67% of the overall size factor ETF market.

Figure 3. Size factor ETF market.

Source: etf.com (2021).

Table 2 gives more detailed information about the biggest size factor ETF providers: the asset under management (AUM), expense ratio (ER) and the segment for the investments.

Table 2. Ranking of the biggest Size ETF providers.

Source: etf.com (2021).

Why should I be interested in this post?

If you are an investor, you may consider adding an exposure to size factor to enhance the overall portfolio return.

Useful resources

Academic research

Banz, R.W., 1981. The relationship between return and market value of common stocks. Journal of Financial Economics, 9: 3-18.

Basu, S., 1977. Investment performance of common stocks in relation to their price-earnings ratios: A test of Efficient Market Hypothesis. The Journal of Finance, 32: 663-682.

Fama, E.F., French, K.R. 1992. The Cross-Section of Expected Stock Returns. The Journal of Finance, 47: 427-465.

Fama, E.F., French, K.R., 2015. A five-factor asset pricing model. Journal of Financial Economics, 116(1): 1-22.

Hasaj, M., Sherer, B., 2021. Covid-19 and Smart-Beta: A Case Study on the Role of Sectors. EDHEC-Risk Institute Working paper.

Litzenberger, R., Ramaswamy, K., 1982. The Effects of Dividends on Common Stock Prices Tax Effects or Information Effects? The Journal of Finance, 37(2): 429-443.

Mangram, M.E., 2013. A simplified perspective of the Markowitz Portfolio Theory. Global Journal of Business Research, 7(1): 59-70.

Pagano, M., Wagner, C., Zechner, J., 2020. Disaster Resilience and Asset Prices, Working paper.

Reinganum, M., 1981. The Arbitrage Pricing Theory: Some Empirical Results. The Journal of Finance, 36(2): 313-321.

Business analysis

etf.com, 2021. Biggest Size Factor ETF providers.

MSCI Investment Research, 2021. Factor Focus: Size.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Momentum Factor

September 4, 2021 by Youssef LOURAOUI

Momentum Factor

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the momentum factor, which is based on a risk factor that aims to get exposure to stocks that have a winning tendency in the upside and downside assuming that they will continue to do well in the short term.

Another similar concept related to momentum is trend following. It is a trading strategy that seeks to profit on an asset’s momentum in a certain direction. A trend occurs when the price moves in a consistent direction (upward or downward). Momentum investing and trading are based on the premise that prices respond to the strength of their supply and demand sources (at least in part) (Investopedia, 2021). It’s considered as a forward-looking strategy. Momentum manifests itself in a variety of different ways. It might be based on publicly traded firms’ earnings reports, the connection between buyers and sellers in the market, or even the usual pace of price rises and decreases in the past.

This article is structured as follows: we begin by defining the momentum factor and reviewing academic studies. The MSCI Momentum Factor Index, which is well used as a benchmark in the asset management industry, is next presented in terms of performance, risk-return trade-off, and behavior during the Covid-19 crisis. We showcase the ETF market for investors looking to profit from the momentum factor.

Definition

In the world of investing, a factor is any attribute that helps explain an asset’s long-term risk and return performance. In the late 1970s, the portfolio management industry’s objective was to capture the market return on a portfolio. As a result of Markowitz and Tobin’s earlier research, William Sharpe, John Lintner, and Jan Mossin independently developed the Capital Asset Pricing Model (CAPM). Because it enabled investors to properly value assets in terms of systematic risk, the CAPM was a significant evolutionary step forward in the theory of capital market equilibrium (Mangram, 2013).

Eugene Fama and Kenneth French, following the CAPM’s original work, developed the Fama-French three-factor model in 1993 to solve the CAPM’s inadequacies. It claims that, in addition to the market risk component of the CAPM, two other factors have an effect on the returns on securities and portfolios: market capitalization (called the “size” factor) and the book-to-market ratio (referred to as the “value” factor).
Other factor characteristics were developed to capture some additional performance as financial research advanced and significant contributions were made.

The Momentum factor refers to a winning stock’s tendency to continue doing well in the short term (Jegandeesh and Titman, 1993).

Academic research

The momentum premium was originally found by academics in 1993, when UCLA researchers Narasimhan Jegadeesh and Sheridan Titman proved that buying well-performing equities and selling underperforming ones provided large positive returns over three to twelve-month holding periods. The study finds that these techniques are profitable not because of their systematic risk or delayed stock price responsiveness to common causes. However, a portion of the anomalous returns achieved in the first year following portfolio creation fade away during the next two years. A similar pattern of returns is often observed around the earnings releases of previous winners and losers (Jegandeesh and Titman, 1993).

Empirical studies

Numerous subsequent research have established that the momentum factor exists across stock sectors, nations, and, more broadly, asset classes. Momentum is not as well understood as other variables, even though several theories seek to explain it. Some feel it is remuneration for taking on a high degree of risk, while others believe it is a result of market inefficiencies caused by delayed pricing reactions to firm-specific information.

While contrarian strategies have garnered much attention in recent academic research, the early work on market efficiency concentrated on relative strength strategies that invest in previous winners and sell past losers. Notably, Levy (1967) asserts that a trading method that purchases equities at prices significantly higher than their average price over the previous 27 weeks generates considerable anomalous profits. Jensen and Bennington (1970), on the other hand, note that Levy developed his trading rule after evaluating 68 alternative trading rules in his dissertation and express reservations about his results as a result (Jegandeesh and Titman, 1993). Jensen and Rennington examine the profitability of Levy’s trading rule over a lengthy period that falls mostly outside of Levy’s initial sample period. They discover that Levy’s trading rule does not outperform a buy and hold strategy throughout their sample period, and so ascribe Levy’s outcome to selection bias (Jegandeesh and Titman, 1993).

Economical interpretation

While the scholarly discussion has shifted away from relative strength trading rules, a lot of practitioners continue to utilize relative strength as a stock selection criterion. For example, Grinblatt and Titman (1989, 1991) found that most mutual funds purchased equities that had grown in price over the preceding quarter (Jegandeesh & Titman, 1993).

MSCI Momentum Factor Index

Risk-adjusted excess return – that is, return that surpasses the benchmark – during a 6-month period
Risk-adjusted excess return that outperforms the benchmark over a 12-month period

These findings conclude in the research paper of Moskowitz et all (1999) hold up to a variety of criteria and treatments and provide critical practical insights into the profitability of momentum investing (Moskowitz, 1999). For example, these findings suggest that momentum strategies are not very well diversified, as both winners and losers typically come from the same industry. Additionally, if trading on momentum is desired, industry-based techniques tend to be more profitable and implementable. Unlike individual stock momentum techniques, which appear to be primarily driven by the sell side, industry momentum generates as much or more profit on the purchase side as on the sell side. Additionally, unlike individual stock momentum, sector momentum earnings continue to be robust among the largest, most liquid companies (Moskowitz, 1999).

Performance of the MSCI Momentum Factor Index

Figure 1 compares MSCI Momentum Factor Index performance to those of other factors from May 1999 to May 2020. All indices are rebalanced on a 100-point scale to ensure consistency in performance and to facilitate factor comparisons.

Figure 1. Performance of the MSCI Momentum Factor Index from 1999-2020.

Source: MSCI Factor research (2021).

According to MSCI research, the momentum component has historically been one of the most effective generators of excess returns, consistently excelling in macro conditions characterized by a prolonged cycle in underlying market trends. As per the figure below, the MSCI World Momentum Index has historically generated excess returns over the long run, outperforming the MSCI World Index by 3.17 percent year since 1999 (MSCI Factor study, 2021).

Risk-return profile of MSCI Momentum Factor Index

Figure 2 shows the MSCI Momentum Factor Index compared to other factors over the period May 1999 – May 2020 in terms of risk/reward. The risk-return tradeoff states that the potential return rises with an increase in risk. Individuals connect low levels of uncertainty about future returns with low potential returns, while high levels of uncertainty or risk are associated with large potential returns. According to the risk-tradeoff trade-off, an investor’s money can generate higher returns only if the investor is willing to endure a higher risk of loss (Figure 2).

Figure 2. Risk-return profile of MSCI Momentum Factor Index compared to a peer group.

Source: MSCI Factor research (2021).

Behavior of the MSCI Momentum Factor Index during the Covid-19 crisis

Origin (01/11/2019 – 01/01/2020): the first instances are reported in Wuhan, China.
Incubation (02/01/2020 – 17/01/2020): during this phase, the number of patients began to rise at a faster rate, raising concerns about the disease’s severity.
Outbreak (20/01/2020 – 21/02/2020): the number of cases rose to the point that the World Health Organization (WHO) decided that this illness may pose a major threat to the world’s population, and the pandemic was proclaimed.
Fever (24/02/2020 – 20/03/2020): markets are extremely volatile, owing to government restrictions aimed at flattening the infection curve, with the decision to impose a lockdown in numerous nations as the most notable measures, among others.
Treatment (23/03/2020 – 15/04/2020): most of this turnaround occurs between March and June 2020, which corresponds with the start of good news about the discovery and widespread use of the vaccine.

Table 1. Performance of the MSCI factor indexes during the Covid-19 crisis.

Source: computation by the author (data source: Thomson Reuters).

Both during the pre-lockdown phase (January 2nd to January 17th 2020) and during the post-lockdown phase (23 March 2020 – 15 April 2021), the momentum component performed well, attaining the second best risk/reward tradeoff (Table 1).

ETFs to capture the Momentum factor

Figure 3 illustrates the overall ETF distribution of the major providers of momentum factor ETFs in terms of percentage of asset under management. By examining the market overview for momentum factor investments, we can observe Blackrock’s dominance (iShares), with assets under management underpinning $27 billion of the overall market value, holding 55% of the overall percentage of the benchmark retained.

Figure 3. Momentum factor ETF market.

Source: etf.com (2021).

Table 2 gives more detailed information about the biggest momentum factor ETF providers: the asset under management (AUM), expense ratio (ER) and the segment for the investments.

Table 2. Ranking of the biggest Momentum ETF providers.

Source: etf.com (2021).

Why should I be interested in this post?

If you are an investor, you may consider adding an exposure to momentum factor to enhance the overall portfolio return.

Useful resources

Academic research

Fama, E.F. and French, K.R. (1992), The Cross-Section of Expected Stock Returns. The Journal of Finance , 47: 427-465.

Jegandeesh, N., Titman, S., 1993. Returns to buying winners and selling losers: Implication for stock market efficiency. The Journal of Finance , 48(1), 1-34.

Jensen, M. C., Benington, G. A. 1970. Random walks and technical theories: Some additional evidence. The Journal of Finance , 25: 469-482

Levy, R. A. 1967. Relative strength as a criterion for investment selection. The Journal of Finance , 22: 595-610.

Mangram, M. E., 2013. A simplified perspective of the Markowitz Portfolio Theory. Global Journal of Business Research , 7(1): 59-70.

Hasaj, M., Sherer, B., 2021. Covid-19 and Smart-Beta: A Case Study on the Role of Sectors. EDHEC-Risk Institute Working Paper, 1-35.

Pagano, M., Wagner, C., Zechner, J. 2020. Disaster Resilience and Asset Prices, Working paper.

Business analysis

etf.com, 2021. Biggest Momentum ETF providers.

MSCI Investment Research, 2021. Factor Focus: Momentum

Investopedia, 2021. The difference between Trends and Momentums

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Yield Factor

September 4, 2021 by Youssef LOURAOUI

Yield Factor

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the yield factor, which is based on a risk factor that aims to get exposure to companies that are regarded to be inexpensive and have a history of consistent and rising dividends.

This article is structured as follows: we begin by defining the yield factor and reviewing academic studies. The MSCI Yield Factor Index, which is well used as a benchmark in the asset management industry, is next presented in terms of performance and risk-return trade-off. We showcase the ETF market for investors looking to profit from the yield factor.

Definition

The yield factor is based on a risk factor that aims to get exposure to companies that are regarded to be inexpensive and have a history of consistent and rising dividends (Arnott and Asness, 2003).

Academic research

Since 1995, and until a recent increase in response to plummeting earnings, market wide dividend-payout ratios in the United States had been in the lowest historical decile, reaching record lows between late 1999 and mid-2001. In other words, earnings retention rates have lately reached or above all-time highs (Arnott and Asness, 2003). Meanwhile, despite the dramatic decline in stock prices since early 2000, price-to-earnings and price-to-dividend ratios remain high by historical standards. With recent valuation ratios so high and dividend payouts so low, the only way future long-term stock returns can approach historical norms is if profits growth accelerates significantly. Certain market analysts, including several prominent Wall Street strategists, do predict extraordinary long-term growth. They attribute this confidence to a variety of factors, including previous policies of low dividend payment ratios. According to the financial literature (Arnott and Asness, 2003), the attractiveness for the yield factor could be explained by the following reasons:

Corporate executives are averse to dividend cuts. Perhaps a high payout ratio reflects managerial confidence in the future stability and increase of earnings, whilst a low payout ratio reflects the reverse. This confidence (or lack thereof) may be founded on public as well as private data
Another explanation compatible with the link we discovered experimentally is that businesses occasionally retain an excessive amount of revenue because of managers’ ambition to construct empires (Jensen, 1986). This conduct does not have to be malicious: A seemingly innocuous coincidence policy of profit retention may end up fostering empire development by accumulating an enticing cash hoard. On the other hand, while funding via share issue and paying significant dividends may be less tax effective, it may subject management to greater scrutiny, eliminate conflicts of interest, and so limit empire building

The article concluded that the empirical evidence supports a world in which managers possess private information that motivates them to pay out a large share of earnings when they are optimistic that dividend cuts will not be necessary and a small share when they are pessimistic, possibly to ensure that dividend payouts are maintained (Arnott and Asness, 2003). Alternatively, the findings match a scenario in which low payment ratios result in inefficient empire building and the backing of less-than-ideal initiatives and investments, resulting in subpar later growth, whereas high payout ratios result in more carefully selected enterprises (Arnott and Asness, 2003). Additionally, the tale of empire-building matches the first macroeconomic facts well. At the moment, these explanations are speculative; further work on distinguishing between conflicting narratives is necessary.

MSCI Yield Factor Index

The MSCI Yield Factor Index concentrate on firms that pay a high dividend yield, but exclude those that lack dividend sustainability, consistency, and quality. It considers securities that fulfill these screening criteria (MSCI Factor research, 2021). Only those having a dividend yield more than 30% of the parent market capitalization index are included.

The yield factor is classified as a “defensive” component, which means that it has historically benefited from economic contraction. For several reasons, investors may be interested in the stock dividend income connected with the yield component. The method has been adopted by institutional investors seeking income outside of the fixed income industry. For example, an insurance business that requires a consistent revenue stream to cover claims may lean its portfolio toward the yield component to accomplish this goal. Additionally, historically, high dividends have accounted for a sizable share of long-term overall portfolio performance (MSCI Factor research, 2021).

Dividend investment is as ancient as stocks, having played a critical part in the growth of firms throughout history. Benjamin Graham and David Dodd, pioneering economists, memorably described dividend distributions as “the primary function of a corporate organisation… A successful business is one that can pay dividends on a consistent basis and, presumably, improve the rate over time” (MSCI Factor research, 2021).

Numerous ideas attempt to explain why high-dividend equities perform so well. One observes that yield investors have favored current dividend payouts above uncertain future capital returns. Additionally, they have viewed dividend increases as a predictor of future success (MSCI Factor research, 2021). Dividend yields have historically been good predictors of profit growth, according to several studies (MSCI Factor research, 2021). A naive high-yielding equity strategy may fall victim to a variety of “yield traps,” including those caused by momentarily high earnings, big dividends, or decreasing stock prices (MSCI Factor research, 2021).

Performance of the MSCI Yield Factor Index

Figure 1 compares the MSCI Yield Factor Index’s performance to those of other factors from May 1999 to May 2020. All indices are rebalanced on a 100-point scale to ensure consistency in performance and to facilitate factor comparisons.

Figure 1. Performance of the MSCI Yield Factor Index from 1999-2020.

Source: MSCI Factor research (2021).

Since 1999, the MSCI Yield Factor Index has consistently earned excess gains of 0.15 percent per year above the MSCI World Index analysed (MSCI Factor research, 2021).

Risk-return profile of MSCI Yield Factor Index

Figure 2 shows the MSCI Yield Factor Index compared to other factors over the period May 1999 – May 2020 in terms of risk/reward. The risk-return trade-off states that the potential return rises with an increase in risk. Individuals connect low levels of uncertainty about future returns with low potential returns, while high levels of uncertainty or risk are associated with large potential returns. According to the risk-return trade-off, an investor’s money can generate higher returns only if the investor is willing to endure a higher risk of loss (Figure 2).

Figure 2. Risk-return profile of MSCI Yield Factor Index compared to a peer group.

Source: MSCI Factor research (2021).

High-yield equity factor investing entails screening for dividends that are sustainable over time. With equity market involvement, it has generated yield income. The MSCI High Dividend Yield Indexes are designed to track the performance of firms that have historically paid steady and rising dividends while avoiding value traps. Outside of fixed income, yield seekers have found the equity yield factor index to have several attractive characteristics, including defensive income, a long-term positive risk premium, and diversification against other factors.

ETFs to capture the Yield factor

Figure 3 illustrates the overall ETF distribution of the major providers of yield factor ETFs in terms of percentage of asset under management. By examining the market overview for minimal volatility factor investments, we can observe Vanguard’s dominance in this factor investing market with 53.46%, representing nearly 164 billion in term of market value in the of the overall yield factor ETF market retained in this benchmark.

Figure 3. Yield factor ETF market.

Source: etf.com (2021).

Table 1 gives more detailed information about the biggest yield factor ETF providers: the asset under management (AUM), expense ratio (ER) and the segment for the investments.

Table 1. Ranking of the biggest Yield ETF providers.

Source: etf.com (2021).

Why should I be interested in this post?

If you are an investor, you may consider adding an exposure to yield factor to enhance the overall portfolio return.

Useful resources

Academic research

Arnott, R. and Asness, C., 2003. Surprise! Higher Dividends = Higher Earnings Growth. Financial Analysts Journal, 59(1): 70-87.

Jensen, M., 1986. Agency Costs of Free Cash Flow, Corporate Finance, and Takeovers. The American Economic Review, 76(2): 323-329.

Hasaj, M., Sherer, B., 2021. Covid-19 and Smart-Beta: A Case Study on the Role of Sectors. EDHEC-Risk Institute Working Paper.

Mangram, M.E., 2013. A simplified perspective of the Markowitz Portfolio Theory.Global Journal of Business Research, 7(1): 59-70.

Pagano, M., Wagner, C., Zechner, J., 2020. Disaster Resilience and Asset Prices, Working paper.

Business analysis

etf.com, 2021. Biggest Yield ETF providers.

MSCI Investment Research, 2021. Factor Focus: Yield.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Value Factor

September 3, 2021 by Youssef LOURAOUI

Value Factor

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the value factor, which is based on a risk factor that aims to get exposure to undervalued firms in relation to their industry competitors in order to benefit from the potential upside.

This article is structured as follows: we begin by defining the value factor and reviewing academic studies. The MSCI Value Factor Index, which is well used as a benchmark in the asset management industry, is next presented in terms of performance, risk-return trade-off, and behavior during the Covid-19 crisis. We showcase the ETF market for investors looking to profit from the value factor.

Definition

In the world of investing, a factor is any aspect that helps explain an asset’s long-term risk and return performance. In the late 1970s, the portfolio management industry’s objective was to capture the market return on a portfolio. As a result of Markowitz and Tobin’s earlier research, William Sharpe, John Lintner, and Jan Mossin independently developed the Capital Asset Pricing Model (CAPM). Because it enabled investors to properly value assets in terms of systematic risk, the CAPM was a significant evolutionary step forward in the theory of capital market equilibrium (Mangram, 2013). Eugene Fama and Kenneth French, following the CAPM’s original work, developed the Fama-French Three-Factor model in 1993 to solve the CAPM’s inadequacies. It claims that, in addition to the market risk component of the CAPM, two other factors have an effect on the returns on securities and portfolios: market capitalization (called the “size” factor) and the book-to-market ratio (referred to as the “value” factor). Other factor characteristics were developed to capture some additional performance as financial research advanced and significant contributions were made.

The value factor is based on a risk factor that aims to get exposure to undervalued firms in relation to their industry competitors in order to benefit from the potential upside (Graham, 1971).

Academic research

The most influential academic studies in the value investing literature may be traced back to Fama and French’s foundational work. In 1993, Eugene Fama and Kenneth French created the Fama-French Three-Factor model in response to the CAPM’s shortcomings. It claims that, in addition to the market risk component introduced by the CAPM, two more variables affect the returns on securities and portfolios: market capitalization (often referred to as “size”) and book-to-market ratio (referred to as the “value” factor). According to Fama and French, the primary justification for include these qualities is that both size and book-to-market (BtM) ratios are related to the business issuing the securities’ economic fundamentals (Fama and French, 1993).

Fama and French assert in 2014 that their initial 1993 three-factor model does not sufficiently explain for some observed discrepancies in anticipated returns. As a result, Fama and French added two more factors to their three-factor model: profitability and investment. These two elements are justified by the dividend discount model’s (DDM) theoretical implications, which assert that profitability and investment contribute to the explanation of the returns obtained from the HML element in the first model (Fama & French, 2015).

Business investors analysis

Benjamin Graham’s book: “The intelligent investor”

The cornerstone of value investing is the belief that low-cost stocks beat higher-cost firms over time. Value is a “pro-cyclical” element, which means that it has tended to gain during periods of economic boom. The seminal work on the value factor is undoubtedly the contribution of Benjamin Graham in his work “The intelligent investor”, one of the most adored and glorified books in finance and considered as a menhir of modern investment (Graham, 1971). According to the value investment approach, he considers that intelligence is not the most important parameter in investing. There’s evidence that a high IQ and a college degree aren’t enough to create a smart investor. Long-Term Capital Management L.P., a hedge fund operated by a squadron of mathematicians, computer scientists, and two Nobel Laureates in Economics (Myron Scholes and Robert C. Merton), lost more than $2 billion in a couple of weeks in 1998 on a massive bet that the bond market would return to “normal.” However, the bond market continued to become increasingly anomalous, and LTCM had borrowed so much money that its failure threatened to capsize the entire financial system. Graham’s work deconstructs several interesting notions that allow one to make a well-reasoned investment decision and to escape from the various cognitive biases that can lead to taking more dangerous positions in the markets (Graham, 1971). In a nutshell, among the most important points for a value investor are (Graham, 1971):

A stock is more than a ticker symbol; it’s a share of ownership in a real firm with a value apart from its share price. The stock market is a pendulum that swings back and forth between unjustified optimism (which pushes up stock prices) and unjustified pessimism (which drives down stock prices) (which makes them too cheap)
A savvy investor buys from pessimists and sells to optimists. The present price of an investment determines its future value. The higher the price you pay, the lower your return
No investor, no matter how careful they are, will ever eliminate the possibility of making a mistake. Only by adhering to Graham’s “margin of safety,” that is, never overpaying for an investment, no matter how attractive it seems, can you decrease your odds of making a mistake
The key to financial success is personal growth in terms of how an investor reacts to market events without including emotions in the decision-making process, as this has a negative impact

Benjamin Graham and David Dodd’s book: “Security Analysis”

With the release of Security Analysis in 1934, Benjamin Graham and David Dodd permanently altered the philosophy and practice of investing. The United States, and indeed the rest of the globe, was engulfed in the Great Depression, a period of unprecedented financial turmoil (Graham & Dodd, 2010). The authors replied with a thorough modification in 1940. Many investors regard the second edition of Security Analysis to be the ultimate word from the most prominent investing philosophers of our time. Security Analysis is still considered the standard text for stock and bond analysis across the world. The work of Graham with “The Intelligent Investor” and “Security Analysis” is regarded as the “bible” of value investing. In a nutshell, the book describes the following aspects (Graham & Dodd, 2010):

The purpose of security analysis is to provide critical information about a stock or bond in an informative and useful manner to a prospective owner; and to make accurate judgments about a security’s safety and attractiveness relative to its current price range based on facts and criteria.
Graham and Dodd describe investing as follows: “An investment activity is one that, after careful analysis, guarantees the safety of money and an acceptable rate of return.” Speculative operations are those that do not comply with these requirements”.
Investors are classified into two types: those who are defensive and those who are adventurous. The former’s portfolio is comprised of a diverse selection of high-price stocks purchased at a discount. The entrepreneurial investor understands the value between market and intrinsic value, which enables him or her to analyze specific stocks in type of and profit from price-to-value discrepancies.
An analysis of a security involves two distinct types of factors: quantitative and qualitative. The former domain should encompass capital structure, earnings power, dividend distributions, and operational effectiveness. The qualitative domain is more ‘fluffy’; it encompasses the ‘character’ of the business, its market position(s), and an appraisal of the management team, among other things. Quantitative data is only useful when accompanied by qualitative analysis.
The most critical word in the book is “earnings power.” The authors emphasize the significance of estimating a company’s real future earnings based on its historical earnings (adjusted for one-time events) as well as its vulnerability to factors such as cyclical swings.

Example of a “value” stock

A value stock is one that trades at a lower price than the company’s actual performance. Because the price of the underlying shares may not reflect the company’s performance, value stock investors seek to profit from market inefficiencies (Investopedia, 2021). Value stocks, for example, include big money center banks. JPMorgan Chase & Co. (JPM) is a value stock that trades at a substantial discount to the market based on earnings.

MSCI Value Factor Index

MSCI Factor Indexes are rules-based, transparent indexes that target equities with favorable factor qualities, as determined by academic discoveries and empirical outcomes, and are designed for easy implementation, replicability, and usage in both standard indexed and active portfolios. The stock price as a multiple of business earnings, the price as a multiple of dividends paid, the price as a multiple of book value, and other “ratio descriptors” are all examples of value. Academics and investors disagree on which business best symbolizes a value company, resulting in a market potential for a range of investment products. On a sector-by-sector basis, the MSCI Enhanced Value Index uses three valuation ratio descriptors:

Forward price to earnings (Fwd P/E)
Enterprise value/operating cash flows (EV/CFO)
Price to book value (P/B)

The index tries to avoid the problems of value investing, such as “value traps,” or stocks that look inexpensive but do not grow in value. The research demonstrates that whole-firm valuation metrics like enterprise value have decreased concentration in highly leveraged businesses (those that have taken on a lot of debt).

Performance of the MSCI Value Factor Index

Figure 1 compares the MSCI Value Factor Index’s performance to those of other factors from May 1999 to May 2020. All indices are rebalanced on a 100-point scale to ensure consistency in performance and to facilitate factor comparisons.

Figure 1. Performance of the MSCI Value Factor Index from 1999-2020.

Source: MSCI Factor research (2021).

Since 1999, the MSCI World Enhanced Value Index has achieved excess returns above the MSCI World Index, with a 1.99 percent annual return over the MSCI World Index as seen above. (MSCI Factor research, 2021).

Risk-return profile of MSCI Value Factor Index

Figure 2 shows the MSCI Value Factor Index compared to other factors over the period May 1999 – May 2020 in terms of risk/reward. The risk-return tradeoff states that the potential return rises with an increase in risk. Individuals connect low levels of uncertainty about future returns with low potential returns, while high levels of uncertainty or risk are associated with large potential returns. According to the risk-tradeoff trade-off, an investor’s money can generate higher returns only if the investor is willing to endure a higher risk of loss (Figure 2).

Figure 2. Risk-return profile of MSCI Value Factor Index compared to a peer group.
Performance of the MSCI Value Factor Index from 1999-2020
Source: MSCI Factor research (2021).

The basis of value investing is identifying stocks whose prices appear to understate their fundamental worth. While many institutional investors may agree, value-index strategies are executed in a number of ways. Incorporating the value factor into a portfolio might potentially boost returns and function as a well-researched performance vector (MSCI Factor research, 2021).

Behavior of the MSCI Value Factor Index during the Covid-19 crisis

Origin (01/11/2019 – 01/01/2020): the first instances are reported in Wuhan, China.
Incubation (02/01/2020 – 17/01/2020): during this phase, the number of patients began to rise at a faster rate, raising concerns about the disease’s severity.
Outbreak (20/01/2020 – 21/02/2020): the number of cases rose to the point that the World Health Organization (WHO) decided that this illness may pose a major threat to the world’s population, and the pandemic was proclaimed.
Fever (24/02/2020 – 20/03/2020): markets are extremely volatile, owing to government restrictions aimed at flattening the infection curve, with the decision to impose a lockdown in numerous nations as the most notable measures, among others.
Treatment (23/03/2020 – 15/04/2020): most of this turnaround occurs between March and June 2020, which corresponds with the start of good news about the discovery and widespread use of the vaccine.

Table 1. Performance of the MSCI factor indexes during the Covid-19 crisis.

Source: computation by the author. Data source: Thomson Reuters.

The value factor has performed not quite well in comparison to the other factors, finishing fourth out of five throughout the time period studied. Additionally, our study demonstrates that the value factor was the poorest performer during the incubation and outbreak stages and the second worst performer during the fever stage. This demonstrates the value factor’s instability during the Covid-19 crisis, which acted as a stress test.

ETFs to capture the Value factor

Figure 3 gives the overall ETF distribution of the major providers of value factor ETFs in terms of asset under management. By examining the market overview for minimal volatility factor investments, we can observe Blackrock (iShares) and State Street Global Advisors as the most dominant players in this segment. They hold nearly 50% and 34% respectively of the overall value factor ETF market, which underpins nearly 117B$ of the overall 138B$ in terms of market value for the value factor ETF market retained in this benchmark.

Figure 3. Value factor ETF market.

Source: etf.com (2021).

Table 2 gives more detailed information about the biggest value factor ETF providers: the asset under management (AUM), expense ratio (ER) and the segment for the investments.

Table 2. Ranking of the biggest Value ETF providers.

Source: etf.com (2021).

Why should I be interested in this post?

If you are an investor, you may consider adding an exposure to value factor to enhance the overall portfolio return.

Useful resources

Academic articles

Fama, E.F. French, K.R., 1992. The Cross-Section of Expected Stock Returns. The Journal of Finance , 47: 427-465.

Fama, E.F. French, K.R., 2015. A five-factor asset pricing model, Journal of Financial Economics , 116(1): 1-22.

Graham, B., Dodd, D., 1934. Security Analysis. 6th Edition, McGraw Hill.

Graham, B., 1949. The Intelligent Investor. 4th edition, Harper Business Essentials.

Hasaj, M., Sherer, B., 2021. Covid-19 and Smart-Beta: A Case Study on the Role of Sectors”. EDHEC-Risk Institute Working Paper.

Mangram, M.E., 2013. A simplified perspective of the Markowitz Portfolio Theory. Global Journal of Business Research, 7(1): 59-70.

Pagano, M., Wagner, C., Zechner, J. 2020. Disaster Resilience and Asset Prices, Working paper.

Business analysis

etf.com, 2021. Biggest Value Factor ETF providers.

MSCI Investment Research, 2021. Factor Focus: Value

Investopedia, 2021. Value Stock Definition.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Minimum Volatility Factor

September 3, 2021 by Youssef LOURAOUI

Minimum Volatility Factor

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the Minimum Volatility Factor, which is based on a risk factor that aims to get exposure to securities with a low volatility profile as measured by beta compared to the market, as well as a low correlation with other assets.

This article is structured as follows: we begin by defining the minimum volatility factor and reviewing academic studies. The MSCI Minimum Volatility Factor Index, which is well used as a benchmark in the asset management industry, is next presented in terms of performance, risk-return trade-off, and behavior during the Covid-19 crisis. We showcase the ETF market for investors looking to profit from the minimum volatility factor.

Definition

Minimum volatility is based on a risk factor that aims to get exposure to securities with a low volatility profile as measured by beta compared to the market, as well as a low correlation with other assets (MSCI Factor research, 2021).

Academic research

In the late 1970s, the portfolio management industry aimed to capture the market portfolio return, but as financial research advanced and certain significant contributions were made, this gave rise to other factor characteristics to capture some additional performance. The financial literature has long advocated for taking on more risk to get a better rate of return. This, however, is a widespread misunderstanding among investors. While extremely volatile equities can deliver spectacular gains, scholarly research has consistently demonstrated that low-volatility companies deliver superior risk-adjusted returns over time. This phenomenon is referred to as the “low volatility anomaly”, and that is why many long-term investors include low volatility factor strategies in their portfolios. This strategy is consistent with Henry Markowitz’s famous 1952 article, in which he preaches the virtues of asset diversification to construct a portfolio that provides the greatest balanced return in a risk-reward framework.

Empirical studies

Figure 1 represents the Markowitz Efficient Frontier, where all the efficient portfolios lie on the upper line. The efficient frontier is a collection of optimum portfolios that provide the highest expected return for a specified level of risk or the lowest risk for a specified level of return. Portfolios that fall below the efficient frontier are suboptimal because they do not provide a sufficient rate of return relative to the degree of risk (Figure 1).

Figure 1. Markowitz Efficient Frontier
Minimum volatility and Markowitz Efficient Frontier
Source: calculations done by the author

Economic interpretation

The term ‘Risk-Reward trade-off’ alludes to Markowitz’s core principle that the riskier an investment, the greater the required potential return. Investors will typically keep a risky investment only if the anticipated return is sufficiently high to compensate them for incurring the risk. Risk is the risk that the actual return on an investment will be less than expected, which is technically defined by standard deviation. A higher standard deviation indicates a greater risk and, thus, a greater potential return. Investors that are willing to take on risk expect to receive a risk premium. The term “risk premium” refers to “the expected return on an investment that is more than the risk-free rate of return”. The greater the risk, the greater the risk premium required by investors.

MSCI Minimum Volatility Factor Index

MSCI Factor Indexes are rules-based, transparent indexes that target equities with favorable factor qualities, as determined by academic discoveries and empirical outcomes, and are designed for easy implementation, replicability, and usage in both standard indexed and active portfolios. The MSCI Minimum Volatility Indexes are created by optimizing a set of sector, country, and factor restrictions to generate an index with the least overall volatility while also maintaining index replicability and investability. The major ways to executing a minimal volatility strategy fall into two categories in terms of methodology: (1) straightforward rank and selection and (2) optimization-based solutions (MSCI Factor research, 2021).

A straightforward technique rates the universe of stocks by anticipated volatility, then picks a subset of the members from the universe and applies a weighting mechanism. The connection between stock returns, which can have a major influence on the overall volatility strategy, is typically ignored in these techniques. While a basic rank and selection technique represents individual stock volatility, optimization-based approaches take into consideration both volatility and correlation effects, or the size and degree to which stocks move in lockstep (MSCI Factor research, 2021).

A naïve unconstrained minimal volatility strategy, on the other hand, has its own set of difficulties, including biases toward certain sectors and nations, undesirable factor exposures, and possibly excessive rebalancing turnover. However, well-designed optimizations with properly defined restrictions may be able to compensate for these flaws. Minimum volatility is classified as a conservative factor, which means that it has tended to benefit from periods of economic contraction. This type of strategy is more concerned with managing volatility than maximizing gains. In this sense, this strategy has produced a premium over the market for long periods, contradicting the principle that investors should not be rewarded with higher risk-adjusted returns for taking less risk than the market (MSCI Factor research, 2021).

The key objective of a minimum volatility strategy is to capture regional and global exposure to potentially less risky stocks. Historically, the MSCI Minimum Volatility Factor Index, for instance, have achieved lower volatility and lower drawdowns (peak-to-trough declines) relative to their factor counterparts during major market downturns (MSCI Factor research, 2021).

Tactical investors have employed MSCI Minimal Volatility Factor Index to decrease risk during market downturns while maintaining equity exposure. The minimum volatility premium was found in the early 1970s by economist Fischer Black coupled with the pioneer work of Portfolio construction of Henry Markowitz in 1952. and built on by others subsequently. After that, according to one idea, investors underpay for low volatility equities because they perceive them to be less lucrative, while overpaying for high volatility equities because they are seen as long-shot prospects for bigger profits. An alternative scholarly argument contends that investors might be overconfident in their abilities to predict the future, and that their views diverge more for high volatility equities, which have fewer predictable outcomes, resulting in increased volatility and poorer returns (MSCI Factor research, 2021).

Performance of the MSCI Minimum Volatility Factor Index

Figure 2 compares the MSCI Minimum Volatility Factor Index’s performance to those of other factors from May 1999 to May 2020. All indices are rebalanced on a 100-point scale to ensure consistency in performance and to facilitate factor comparisons.

Figure 2. Performance of the MSCI Minimum Volatility Factor Index from 1999-2020.

Source: MSCI Factor research, 2021.

With a 1.16% percent yearly return over the MSCI World Index since 1999, the MSCI World Minimum Volatility (USD) Index has consistently provided excess profits over the long run while maintaining a profile of risk among the most conservative of the peer group analysed (MSCI Factor research, 2021).

Risk-return profile of MSCI Minimum Volatility Factor Index

Figure 3 shows the MSCI Minimum Volatility Factor Index compared to other factors over the period May 1999 – May 2020 in terms of risk/reward. The risk-return tradeoff states that the potential return rises with an increase in risk. Individuals connect low levels of uncertainty about future returns with low potential returns, while high levels of uncertainty or risk are associated with large potential returns. According to the risk-tradeoff trade-off, an investor’s money can generate higher returns only if the investor is willing to endure a higher risk of loss (Figure 3).

Figure 3. Risk-return profile of MSCI Minimum Volatility Factor Index compared to a peer group.

Source: MSCI Factor research, 2021.

Behavior of the MSCI Minimum Volatility Factor Index during the Covid-19 crisis

Origin (01/11/2019 – 01/01/2020): the first instances are reported in Wuhan, China.
Incubation (02/01/2020 – 17/01/2020): during this phase, the number of patients began to rise at a faster rate, raising concerns about the disease’s severity.
Outbreak (20/01/2020 – 21/02/2020): the number of cases rose to the point that the World Health Organization (WHO) decided that this illness may pose a major threat to the world’s population, and the pandemic was proclaimed.
Fever (24/02/2020 – 20/03/2020): markets are extremely volatile, owing to government restrictions aimed at flattening the infection curve, with the decision to impose a lockdown in numerous nations as the most notable measures, among others.
Treatment (23/03/2020 – 15/04/2020): most of this turnaround occurs between March and June 2020, which corresponds with the start of good news about the discovery and widespread use of the vaccine.

Table 1. Performance of the MSCI factor indexes during the Covid-19 crisis.

Source: computation by the author (Data source: Thomson Reuters).

One conclusion that can be drawn from our research supports the reason for the minimal volatility strategy, namely, to minimize portfolio volatility by keeping limited exposure to highly volatile stocks. In this respect, the Covid-19 pandemic period served as a significant stress test for this strategy, which outperformed the other return factors in the period preceding and following worldwide containment, with a risk-reward trade-off much higher than the average of the chosen factors.

ETFs to capture the Minimum Volatility factor

Figure 4 gives the overall ETF distribution of the major providers of minimal volatility factor ETFs in terms of asset under management. By examining the market overview for minimal volatility factor investments, we can observe Blackrock ETFs (iShares) dominance, with 78.43% of the overall minimum volatility factor ETF market. This represents roughly 47B$ of the overall minimum volatility market retained for this benchmark.

Figure 4. Minimum Volatility factor ETF market.

Source: etf.com, 2021.

Table 2 gives more detailed information about the biggest minimum volatility factor ETF providers: the asset under management (AUM), expense ratio (ER) and 3-month total return (3-Mo TR) and the segment for the investments.

Table 2. Ranking of the biggest Minimum Volatility ETF providers.
Minimum Volatility factor ETF market actors
Source: etf.com, 2021.

Why should I be interested in this post?

You may have seen the CAPM linked to the market factor in your 101 finance course if you are an undergraduate or graduate student at a business school or university. This article raises awareness of the presence of other additional risk factors.

If you’re an investor, you might want to explore increasing your exposure to the minimum volatility factor to boost your portfolio’s total return.

Useful resources

Academic research

Markowitz, H., 1952. Portfolio Selection. The Journal of Finance, 7(1): 77-91.

Mangram, M.E., 2013. A simplified perspective of the Markowitz Portfolio Theory. Global Journal of Business Research, 7(1): 59-70

Hasaj, M., Sherer, B., 2021. Covid-19 and Smart-Beta: A Case Study on the Role of Sectors. EDHEC-Risk Institute Working Paper.

Pagano, M., Wagner, C., Zechner, J. 2020. Disaster Resilience and Asset Prices, Working paper.

Business analysis

etf.com, 2021. Biggest Minimum Volatility ETF providers.

MSCI Investment Research, 2021. Factor Focus: Volatility.

About the author

The article was written in September 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

Is smart beta really smart?

April 3, 2021 by Youssef LOURAOUI

Is smart beta really smart?

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021) presents the concept of smart beta used in the asset management industry.

Mutual funds and Exchange traded funds (ETF) based on the smart beta approach have increased in size during the recent years. As Burton Malkiel (2014), we also wonder if the smart beta approach is really smart.

The smart beta industry

Smart beta funds have experienced a significant growth with total assets under management approaching market 620 billion dollar in the U.S. as shown in Figure 1 (Morningstar Reseach, 2017).

Figure 1. Smart Beta Exchange Traded Products growth in the US market (2000-2017).

Source: Morningstar Research (2017).

Traditional approach in portfolio management

The traditional approach to build asset portfolio is to define asset weights based on the market capitalization. The framework of this traditional approach is based on the Capital Asset Pricing Model (CAPM) introduced by the work of Henry Markowitz and William Sharpe in 1964. The CAPM is based on a set of hypotheses about the market structure and investors:

No intermediaries
No constraints (possibility of short selling)
Supply and demand equilibrium
Inexistence of transaction cost
Investors seeks to maximise its portfolio value by optimizing the mean associated with expected returns while minimizing variance associated with risk
Investors are considered as “rational” with a risk averse profile
Investors have access to the information simultaneously in order to execute their investment ideas

Under this framework, Markowitz developed a model relating the expected return of a given asset and its risk:

Relation between expected return and risk

where E(r) represents the expected return of the asset, r_f the risk-free rate, β a measure of the risk of the asset and E(r_m) the expected return of the market.

In this model, the beta (β) parameter is a key parameter and is defined as:

Beta

where Cov(r,r_m) represents the covariance of the asset with the overall market, and σ(r_m)² is the variance of market return.

The beta represents the sensibility of the asset to the fluctuations of the market. This risk measure helps investors to predict the movements of their asset according to the movement of the market overall. It measures the asset volatility in comparison with the systematic risk inherent to the market. Statistically, the beta represents the slope of the line through a regression of data points between the stock returns in comparison to the market returns. It helps investors to explain how the asset moves compared to the market.

More specifically, we can consider the following cases for beta values:

β = 1 indicates a fluctuation between the asset and its benchmark, thus the asset tends to move in a similar rate than the market fluctuations. A passive ETF replicating an index will present a beta close to 1 with its associated index.
0 < β < 1 indicates that the asset moves in a slower rate than market fluctuations. Defensive stocks, stocks that deliver consistent returns without regarding the market state like P&G or Coca Cola in the US, tend to have a beta with the market lower than 1.
β > 1 indicates a more aggressive effect of amplification between the asset price movements with the market movements. Call options tend to have higher betas than their underlying asset.
β = 0 indicates that the asset or portfolio is uncorrelated to the market. Govies, or sovereign debt bonds, tend to have a beta-neutral exposure to the market.
β < 0 indicates an inverse effect of market fluctuation impact in the asset volatility. In this sense, the asset would behave inversely in terms of volatility compared to the market movements. Put options and Gold typically tend to have negative betas.

In order to better monitor the performance of an actively managed fund, active fund managers seek to improve the performance of their fund compared to the market. This additional performance is measured by the “alpha” (Jensen, 1968) defined by:

Alpha Jensen

where E(r) is the average return of the fund over the period studied, r_f the risk-free rate, E(r_m) the expected return of the market, and β×(E(r_m)-r_f) represents the systematic risk of the fund.

Jensen’s alpha (α) represents the abnormal returns of the fund.

The Smart beta approach

The smart beta approach is based on the construction of a portfolio of assets using several different yield enhancement “factors”. BlackRock Investment Solutions (2021) lists the following factors mainly used in the smart beta approach:

Quality, which aims to study the financial environment of the underlying asset.
Volatility which aims to filter assets according to their risk.
Momentum, which aims to identify trends in the selection of assets to be retained by focusing on stocks that have performed strongly in the short term.
Growth is the approach that aims to select securities that have strong return expectations in the medium to long term.
Size which aims to classify according to the size of the assets.
Value that seeks to denote undervalued assets that are close to their fundamental values.

The smart beta approach is opposed to the traditional portfolio approach where a portfolio is constructed using the weights defined by the market capitalization of its assets. The smart beta approach aims to position the portfolio sensitivity or “beta” according to the market environment expectation of the asset manager. For a bull market, the fund manager will select a set of factors to achieve a pronounced exposure of his portfolio. Symmetrically, for a bear market, the fund manager will select another set of factors opting for a beta neutral approach to protect the sensitivity of his portfolio against bear market fluctuations.

Performance and impact factor

S&P Group (2016) studied the performance of different factors (volatility, momentum, quality, value, dividend yield, growth and size) on the S&P500 index for 1994-2014 broken down into sub-sectors (see Table 1). This study finds that each sector is impacted differently by choosing one factor over another. For example, in the energy sector, the strategies of value and growth has led to a positive performance with respectively 1.22% and 2.56%, while in the industrial sector, the strategies of size were the only factor with a positive performance of 1.66%. In practice, there are two approaches: focusing on a single factor or finding a combination of factors that offers the most interesting risk-adjusted return to the investor in view of his/her investment strategy.

Table 1. Sector exposures to smart beta factors (1994-2014).

Source: S&P Research (2014).

S&P Group (2016) also studies the performance of the factors according to the market cycles (bull, bear or recovery markets), business cycles (expansion or contraction) and investor sentiment (neutral, bullish and bearish). The study shows how each factor has been mostly effective for every market condition.

Table 2. Performance of factors according to different market cycles, business cycles and investor sentiment.

Source: S&P Research (2014).

In summary, the following characteristics of the different approaches discussed in this article can be identified:

The CAPM approach aims to give a practical configuration of the relationship between the return of an asset with the market return as well as the return considered as risk-free.
Alpha is an essential metric in the calculation of the portfolio manager’s return in an actively managed fund. In this sense, alpha and CAPM are linked in the fund given the nature of the formulas used.
Smart beta or factor investing follows an approach that straddles the line between active and passive management where the manager of this type of fund will use factors to filter its source of return generation which differs from the common approach based on CAPM reasoning (Fidelity, 2021).
The conductive link of these three reasoning is closely related to the fact that historically the CAPM model has been a pillar in financial theory, the smart beta being a more recent approach that tries to disrupt the codes of the so-called market capitalization based investment by integrating factors to increase the sources of return. Alpha is related to smart beta in the sense that the manager of this type of fund will want to outperform a benchmark and therefore, alpha allows to know the nature of this out-performance of the manager compared to a benchmark.

Is smart beta really smart?

Nevertheless, the vision of this smart beta approach has raised criticisms regarding the relevance of the financial results that this strategy brings to a portfolio’s return. Malkiel (2014) questioned the smartness of smart beta and found that the performance of this new strategy is only the result of chance in the sense that the persistence of performance is dependent in large part on the market configuration.

In his analysis of the performance of the smart ETF fund called FTSE RAFI over the period 2009-2014, he attributed the out-performance to luck. The portfolio allocation was highly exposed to two financial stocks, Citigroup and Bank of America, which accounted for 15% of the portfolio allocation. Note that Citigroup and Bank of America were prosecuted by the American courts for post-crisis financial events and interest rate manipulation operations related to the LIBOR scandal. This smart beta fund outperformed the passive managed US large cap ETF (SPY). Malkiel associated the asset selection of the FTSE RAFI fund with a bet on Bank of America that with another market configuration it could have ended in a sadder way.

Figure 2. FTSE RAFI ETF (orange) compared with its benchmark (FTSE RAFI US 1000) and with SPY ETF (green).

Source: Thomson Reuters Datastream.

We can conclude that the smart beta strategy can allow, as outlined in Blackrock’s research (BlackRock Investment Solutions, 2021), an opportunity to improve portfolio performance while seeking to manage variables such as portfolio out-performance, minimizing its volatility compared to the market or seeking diversification to reduce the risk of the investor’s portfolio. It is an instrument that must be taken judiciously in order to be able to affirm in fine if it is smart in the end, as Malkiel would say.

Useful resources

Academic articles

Malkiel, B. (2014). Is Smart Beta smart? The Journal of Portfolio Management 40, 5: 127-134

El Lamti N. (2017) Are smart beta strategies really smart? HEC Paris.

Business resources

BlackRock Investment Solutions (2021) What is Factor Investing

Fidelity (2021) Smart beta

S&P Global Research (2016) What Is in Your Smart Beta Portfolio? A Fundamental and Macroeconomic Analysis

Morningstar Research (2017) A Global Guide to Strategic-Beta Exchange-Traded Products

Fidelity (2021) Smart beta

About the author

The article in April 2021 was written by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2017-2021).

ETFs in a changing asset management industry

February 3, 2021 by Youssef LOURAOUI

ETFs in a changing asset management industry

In this article, Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2016-2020) talks about his research conducted in the field of investing.

As a way of introduction, ETFs have been captivating investors’ attention in the last 20 years since their creation. This financial innovation has shaped how investors place their capital.

Definition

An ETF can be defined as a financial product that is based on a basket of different assets, to replicate the actual performance of each selected investment. An ETF has more or less the same proportion of the underlying components of the basket, depending on the style of management of the asset manager. ETFs represent nearly 90% of the asset under management of the global Exchange Traded Products (ETP).

History

The first ETF was the Standard and Poor’s Depository Receipts (SPDR) introduced in 1993. It appears to be an optimized product that enables investors to trade it like a stock, with a price that fluctuates during the day (not like mutual funds whose value is known at the end of the day only). The main advantage of ETFs for investors is to diversify their investment with lower fees than buying each underlying asset separately. The most important ETFs in the market are the ones with the lowest expense ratio as it is a crucial point to attract money from investors in the fund.

Types of ETF

ETFs can be segmented in different types according to the asset class, geography, sector, investment style among other criteria. According to Blackrock’s classification (2021), the overall ETF market can be divided into the following classes:

Stock ETFs track a certain stock market index, such as the S&P 500 or NASDAQ.
Bond ETFs offer exposure to a wide selection of fixed income instruments.
Sector and industry ETFs invest in a particular industry such as technology, healthcare, or financials.
Commodity ETFs track the price of a commodity such as oil, gold, or wheat.
Style ETFs are devoted to an investment style or market capitalization focus such as large-cap value or small-cap growth.
Alternative ETFs offer exposure to the alternative asset classes and invest in strategies such as real estate, hedge funds and private equity.
Foreign market ETFs follow non-U.S. markets such as the United Kingdom’s FTSE 100 index or Japan’s Nikkei index.
Actively managed ETFs aim to provide a certain outcome to maximize income or outperform an index, while most ETFs are designed to track an index.

Figure 1. Volume of the ETF market worldwide 2003-2019.

Source: Statista (2021).

Figure 1 represents the volume of the ETF market worldwide over the period 2003-2019. With over 6,970 ETFs globally as of 2019 (Statista, 2021), the ETF industry is growing at an increasing pace, recording a thirty-fold increase in terms of market capitalization in the 17-year timeframe of the analysis. It reflects the growing appetite of investors towards this kind of financial instruments as they offer the opportunity for investors to invest virtually in every asset class, geographical region, sector, theme, and investment style (BlackRock, 2021).

iShares (BlackRock), Xtrackers (DWS) and Lyxor (Société Générale) can also be highlighted as key players of the ETF industry in Europe. As shown in Figure 2, Lyxor (a French player) is ranked 3rd most important player with nearly 9% of the overall European ETF market (Refinitiv insights, 2019). iShares represents nearly eight times the weight of Lyxor, which is slightly above the average of the overall European ETF volume in dollars.

Figure 2. Market share at the promoter level by Assets Under Management (March 31, 2019)

Source: Refinitiv insights (2019).

It goes without saying that the key player worldwide remains BlackRock with nearly 1/3 of the global ETF market capitalization. According to Arte documentary, BlackRock is without a doubt a serious actor of the ETF industry as shown in Figure 2 with an unrivaled market share in the European and global ETF market. With more than 7 trillion of asset under management, BlackRock is the leading powerhouse of the asset management industry.

Benefits of ETF

The main benefits of investing in ETFs is the ability to invest in a diversified and straightforward manner in financial markets by owning a chunk of an index with a single investment. It allows investors to position their wealth in a reference portfolio based on equities, bonds or commodities. It also helps them to create a portfolio that suits their needs or preferences in terms of expected return and risk and also liquidity as ETFs can be bought and sold at any moment of the day. Finally, ETFs also allow investors to implement long/short strategies among others.

Risks

Market risk is an essential component to fully understand the risk of owning an ETF. According to the foundations of the modern portfolio theory (Markowitz, 1952), an asset can be deconstructed into two risk factors: an idiosyncratic risk inherent to the asset and a systematic risk inherent to the market. As an ETF are composed of a basket of different assets, the idiosyncratic risk can be neutralized by the effect of diversification, but the systematic risk, also called the market risk is not neutralized and is still present in the ETF.

In terms of risk, we can mention the volatility risk arising from the underlying assets or index that the ETF tries to replicate. In this sense, when an ETF tries to emulate the performance of the underlying asset, it will also replicate its inherent risk (the systematic and non-systematic risk of the underlying asset). This will have a direct impact on the overall risk-return characteristic of investors’ portfolio.

The second risk, common to all funds and that can have a significant impact on the overall performance, concerns the currency risk when the ETF owned doesn’t use the same currency as the underlying asset. In this sense, when owning an ETF that tracks another asset that is quoted in another currency is inherently, investors bears some currency risk as the fluctuations of the pair of currencies can have a significant impact on the overall performance of the position of the investor.

Liquidity risk arises from the difficulty to buy and sell a security in the market. The more illiquid the market, the wider the spreads to compensate the market maker for the task of connecting buyers and sellers. Liquidity is an important concern when picking an ETF as it can impact the performance of the portfolio overall.

Another risk particular to this instrument, is what is called the tracking error between the ETF value and its benchmark (the index that the ETF tries to replicate). This has a significant impact as, depending on the overall dispersion, the mismatch in terms of valuation between the ETF and the benchmark can impact the returns of investors’ portfolio overall.

Passive management and the concept of efficient market

Most ETFs corresponds to “passive” management as the objective is just to replicate the performance of the underlying assets or the index. Passive management is related to the Efficient Market Hypothesis (EMH), assuming that the market is efficient. Passive fund managers aim to replicate a given benchmark believing that in efficient markets active fund management cannot beat the benchmark on the long term.

Passive fund managers invest their funds by:

Pure replication of the benchmark by investing in each component of the basket (vanilla ETF)
Synthetic reproduction of the benchmark by replicating the basket with derivatives products (like futures contracts).

An important concept is market efficiency (also known as the informational efficiency), which is defined as the ability of the market to incorporate all the available information. Efficient market is a state of the market where information is rationally processed and quickly incorporated in the market price.

It is in the heart of the preoccupations of fund managers and analysts to unfold any efficiency in the market because the degree of efficiency impacts their returns directly (CFA Institute, 2011). Fama (1970) proposed a framework analyzing the degree of efficiency in a market. He distinguishes three forms of market efficiency (weak, semi-strong and strong) which correspond to the degree in which information is incorporated in the prices. Earning consistently abnormal returns based on trading with information is the opposite view of what an efficient market is.

The weak form of market efficiency refers to information composed of past market data (past transaction prices and volumes). In a weakly efficient market, past market information is already included in the current market price, and investors will not be able to distinguish any pattern or prediction of future prices based on past data.
The semi-strong of market efficiency refers to publicly available information. This includes market data (as in the week form) and financial disclosed data (financial accounts published by firms, press articles, reports by financial analysts, etc.). If a market is considered in the semi-strong sense, then it must be in a weak sense as well. In this context, there is no additional gain in determining under or overvalued security as all the public data is already incorporated in the asset price.
The strong of market efficiency refers to all information (both public and private). Markets are strongly efficient when they reflect all the available information at any time in the asset prices.

Useful resources

Academic resources

Fama, E. (1970) “Efficient Capital Markets: A Review of Theory and Empirical Work” Journal of Finance 25(2), 383–417.

Business

Arte documentary (2014) “Ces financiers qui dirigent le monde: BlackRock”.

BlackRock (January 2021) ETF overview.

Refinitiv insights (2019) Concentration of the major players in the European ETF market.

About the author

The article was written in February 2021 by Youssef LOURAOUI (ESSEC Business School, Global Bachelor of Business Administration, 2016-2020).

Arbitrage Pricing Theory (APT)

Introduction

Assumptions of the APT model

Capital Asset Pricing Model (CAPM)

Mathematical foundations

Application

Applying APT

Excel file for the APT application

Why should I be interested in this post?

Related posts on the SimTrade blog

Useful resources

Academic research

Business Analysis

Time series

About the author

Introduction

Mathematical foundation of Black-Litterman model

Black-Litterman asset allocation in practice

Why should I be interested in this post?

Related posts on the SimTrade blog

Useful resources

Academic research

About the author

Overview of the market

BlackRock dominance

Why should I be interested in this post?

Related posts on the SimTrade blog

Factor investing

Factors

Useful resources

Business analysis

About the author

Definition

Factor

Performance analysis

MSCI Factor Index

Performance of factors over time

Why should I be interested in this post?

Related posts on the SimTrade blog

Factor investing

Factors

Useful resources

Business analysis

About the author

Definition

Characteristics of smart beta 2.0 strategies

Why should I be interested in this post?

Related posts on the SimTrade blog

Factor investing

Factors

Useful resources

About the author

Definition

Empirical study: monkeys vs passive mangers

Why should I be interested in this post?

Related posts on the SimSrade blog

Factor investing

Factor

Useful resources

Academic research

About the author

Introduction

Heuristic-based weighting strategies

Equal-weighting

Fundamental-weighting

Low beta weighting

Reverse-capitalization weighting

Maximum diversification

Optimization-based weighting strategies

Minimum Variance

Maximum Sharpe ratio

Why should I be interested in this post?

Related posts on the SimTrade blog

Factor investing

Factors

Useful resources

Academic research

About the author

Markowitz Modern Portfolio Theory

Modern Portfolio Theory