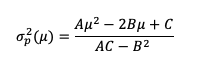In this article, Youssef LOURAOUI (Bayes Business School, MSc. Energy, Trade & Finance, 2021-2022) elaborates on the concept of Minimum Volatility Portfolio, which is derived from Modern Portfolio Theory (MPT) and also in practice to build investment funds.
This article is structured as follows: we introduce the concept of Minimum Volatility Portfolio. Next, we present some interesting academic findings, and we finish by presenting a theoretical example to support the explanations given in this article.
Introduction
The minimum volatility portfolio represents a portfolio of assets with the lowest possible risk for an investor and is located on the far-left side of the efficient frontier. Note that the minimum volatility portfolio is also called the minimum variance portfolio or more precisely the global minimum volatility portfolio (to distinguish it from other optimal portfolios obtained for higher risk levels).
Modern Portfolio Theory’s fundamental notion had significant implications for portfolio construction and asset allocation techniques. In the late 1970s, the portfolio management business attempted to capture the market portfolio return. However, as financial research progressed and some substantial contributions were made, new factor characteristics emerged to capture extra performance. The financial literature has long encouraged taking on more risk to earn a higher return. However, this is a common misconception among investors. While extremely volatile stocks can produce spectacular gains, academic research has repeatedly proved that low-volatility companies provide greater risk-adjusted returns over time. This occurrence is known as the “low volatility anomaly,” and it is for this reason that many long-term investors include low volatility factor strategies in their portfolios. This strategy is consistent with Henry Markowitz’s renowned 1952 article, in which he embraces the merits of asset diversification to form a portfolio with the maximum risk-adjusted return.
Academic Literature
Markowitz is widely regarded as a pioneer in financial economics and finance due to the theoretical implications and practical applications of his work in financial markets. Markowitz received the Nobel Prize in 1990 for his contributions to these fields, which he outlined in his 1952 Journal of Finance article titled “Portfolio Selection.” His seminal work paved the way for what is now commonly known as “Modern Portfolio Theory” (MPT).
In 1952, Harry Markowitz created modern portfolio theory with his work. Overall, the risk component of MPT may be evaluated using multiple mathematical formulations and managed through the notion of diversification, which requires building a portfolio of assets that exhibits the lowest level of risk for a given level of expected return (or equivalently a portfolio of assets that exhibits the highest level of expected return for a given level of risk). Such portfolios are called efficient portfolios. In order to construct optimal portfolios, the theory makes a number of fundamental assumptions regarding the asset selection behavior of individuals. These are the assumptions (Markowitz, 1952):
- The only two elements that influence an investor’s decision are the expected rate of return and the variance. (In other words, investors use Markowitz’s two-parameter model to make decisions.) .
- Investors are risk averse. (That is, when faced with two investments with the same expected return but two different risks, investors will favor the one with the lower risk.)
- All investors strive to maximize expected return at a given level of risk.
- All investors have the same expectations regarding the expected return, variance, and covariances for all hazardous assets. This assumption is known as the homogenous expectations assumption.
- All investors have a one-period investment horizon.
Only in theory does the minimum volatility portfolio (MVP) exist. In practice, the MVP can only be estimated retrospectively (ex post) for a particular sample size and return frequency. This means that several minimum volatility portfolios exist, each with the goal of minimizing and reducing future volatility (ex ante). The majority of minimum volatility portfolios have large average exposures to low volatility and low beta stocks (Robeco, 2010).
Example
To illustrate the concept of the minimum volatility portfolio, we consider an investment universe composed of three assets with the following characteristics (expected return, volatility and correlation):
- Asset 1: Expected return of 10% and volatility of 10%
- Asset 2: Expected return of 15% and volatility of 20%
- Asset 3: Expected return of 22% and volatility of 35%
- Correlation between Asset 1 and Asset 2: 0.30
- Correlation between Asset 1 and Asset 3: 0.80
- Correlation between Asset 2 and Asset 3: 0.50
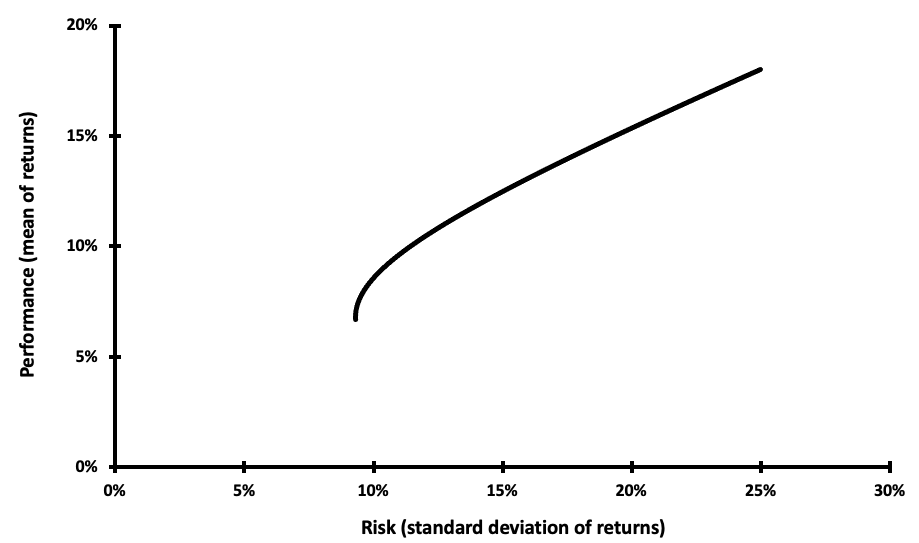
The first step to achieve the minimum variance portfolio is to construct the portfolio efficient frontier. This curve represents all the portfolios that are optimal in the mean-variance sense. After solving the optimization program, we obtain the weights of the optimal portfolios. Figure 1 plots the efficient frontier obtained from this example. As captured by the plot, we can see that the minimum variance portfolio in this three-asset universe is basically concentrated on one holding (100% on Asset 1). In this instance, an investor who wishes to minimize portfolio risk would allocate 100% on Asset 1 since it has the lowest volatility out of the three assets retained in this analysis. The investor would earn an expected return of 10% for a volatility of 10% annualized (Figure 1).
Figure 1. Minimum Volatility Portfolio (MVP) and the Efficient Frontier.
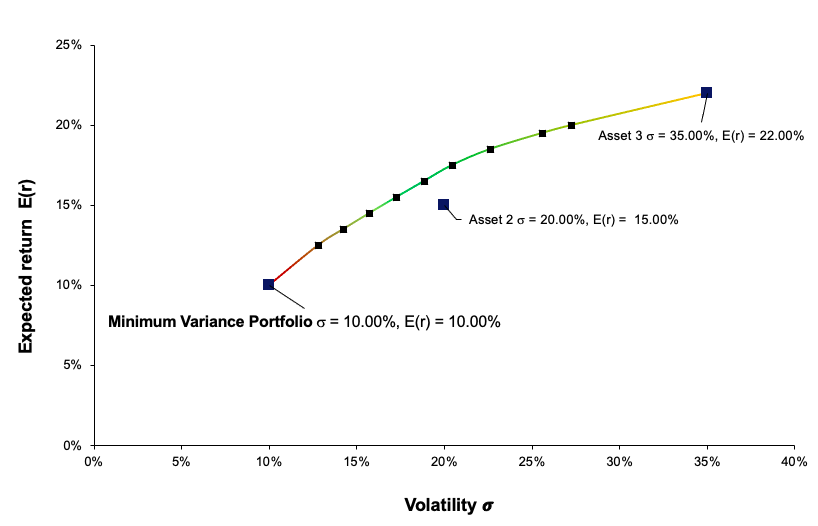
Source: computation by the author.
Excel file to build the Minimum Volatility Portfolio
You can download below an Excel file in order to build the Minimum Volatility portfolio.
Why should I be interested in this post?
Portfolio management’s objective is to optimize the returns on the entire portfolio, not just on one or two stocks. By monitoring and maintaining your investment portfolio, you can accumulate a sizable capital to fulfil a variety of financial objectives, including retirement planning. This article helps to understand the grounding fundamentals behind portfolio construction and investing.
Related posts on the SimTrade blog
▶ Youssef LOURAOUI Markowitz Modern Portfolio Theory
▶ Jayati WALIA Capital Asset Pricing Model (CAPM)
▶ Youssef LOURAOUI Origin of factor investing
▶ Youssef LOURAOUI Minimum Volatility Factor
▶ Youssef LOURAOUI Beta
▶ Youssef LOURAOUI Portfolio
Useful resources
Academic research
Lintner, John. 1965a. Security Prices, Risk, and Maximal Gains from Diversification. Journal of Finance, 20, 587-616.
Lintner, John. 1965b. The Valuation of Risk Assets and the Selection of Risky Investments in Stock Portfolios and Capital Budgets.Review of Economics and Statistics 47, 13-37.
Markowitz, H., 1952. Portfolio Selection. The Journal of Finance, 7, 77-91.
Sharpe, William F. 1963. A Simplified Model for Portfolio Analysis. Management Science, 19, 425-442.
Sharpe, William F. 1964. Capital Asset Prices: A Theory of Market Equilibrium under Conditions of Risk. Journal of Finance, 19, 425-442.
Business analysis
Robeco, 2010 Ten things you should know about minimum volatility investing.
About the author
The article was written in January 2023 by Youssef LOURAOUI (Bayes Business School, MSc. Energy, Trade & Finance, 2021-2022).