Fixed-income arbitrage strategy

In this article, Youssef LOURAOUI (Bayes Business School, MSc. Energy, Trade & Finance, 2021-2022) presents the fixed-income arbitrage strategy which is a well-known strategy used by hedge funds. The objective of the fixed-income arbitrage strategy is to benefit from trends or disequilibrium in the prices of fixed-income securities using systematic and discretionary trading strategies.

This article is structured as follow: we introduce the fixed-income arbitrage strategy principle. Then, we present a practical case study to grasp the overall methodology of this strategy. We also present a performance analysis of this strategy and compare it a benchmark representing all hedge fund strategies (Credit Suisse Hedge Fund index) and a benchmark for the global equity market (MSCI All World Index).

Introduction

According to Credit Suisse (a financial institution publishing hedge fund indexes), a fixed-income arbitrage strategy can be defined as follows: “Fixed-income arbitrage funds attempt to generate profits by exploiting inefficiencies and price anomalies between related fixed-income securities. Funds limit volatility by hedging out exposure to the market and interest rate risk. Strategies include leveraging long and short positions in similar fixed-income securities that are related either mathematically or economically. The sector includes credit yield curve relative value trading involving interest rate swaps, government securities and futures, volatility trading involving options, and mortgage-backed securities arbitrage (the mortgage-backed market is primarily US-based and over-the-counter)”.

Types of arbitrage

Fixed-income arbitrage makes money based on two main underlying concepts:

Pure arbitrage

Identical instruments should have identical price (this is the law of one price). This could be the case, for instance, of two futures contracts traded on two different exchanges. This mispricing could be used by going long the undervalued contract and short the overvalued contract. This strategy uses to work in the days before the rise of electronic trading. Now, pure arbitrage is much less obvious as information is accessible instantly and algorithmic trading wipe out this kind of market anomalies.

Relative value arbitrage

Similar instruments should have a similar price. The fundamental rationale of this type of arbitrage is the notion of reversion to the long-term mean (or normal relative valuations).

Factors that influence fixed-income arbitrage strategies

We list below the sources of market inefficiencies that fixed-income arbitrage funds can exploit.

Market segmentation

Segmentation is of concern for fixed-income arbitrageurs. In financial institutions, the fixed-income desk is split into different traders looking at specific parts of the yield curve. In this instance, some will focus on very short, dated bonds, others while concentrate in the middle part of the yield curve (2-5y) while other while be looking at the long-end of the yield curve (10-30y).

Regulation

Regulation has an implication in the kind of fixed-income securities a fund can hold in their books. Some legislations regulate actively to have specific exposure to high yield securities (junk bonds) since their probability of default is much more important. The diminished popularity linked to the tight regulation can make the valuation of those bonds more attractive than owning investment grade bonds.

Liquidity

Liquidity is also an important concern for this type of strategy. The more liquid the market, the easier it is to trade and execute the strategy (vice versa).

Volatility

Large market movements in the market can have implications to the profitability of this kind of strategy.

Instrument complexity

Instrument complexity can also be a reason to have fixed-income securities. The events of 2008 are a clear example of how banks and regulators didn’t manage to price correctly the complex instruments sold in the market which were highly risky.

Application of a fixed-income arbitrage

Fixed-income arbitrage strategy makes money by focusing on the liquidity and volatility factors generating risk premia. The strategy can potentially generate returns in both rising and falling markets. However, understanding the yield curve structure of interest rates and detecting the relative valuation differential between fixed-income securities is the key concern since this is what makes this strategy profitable (or not!).

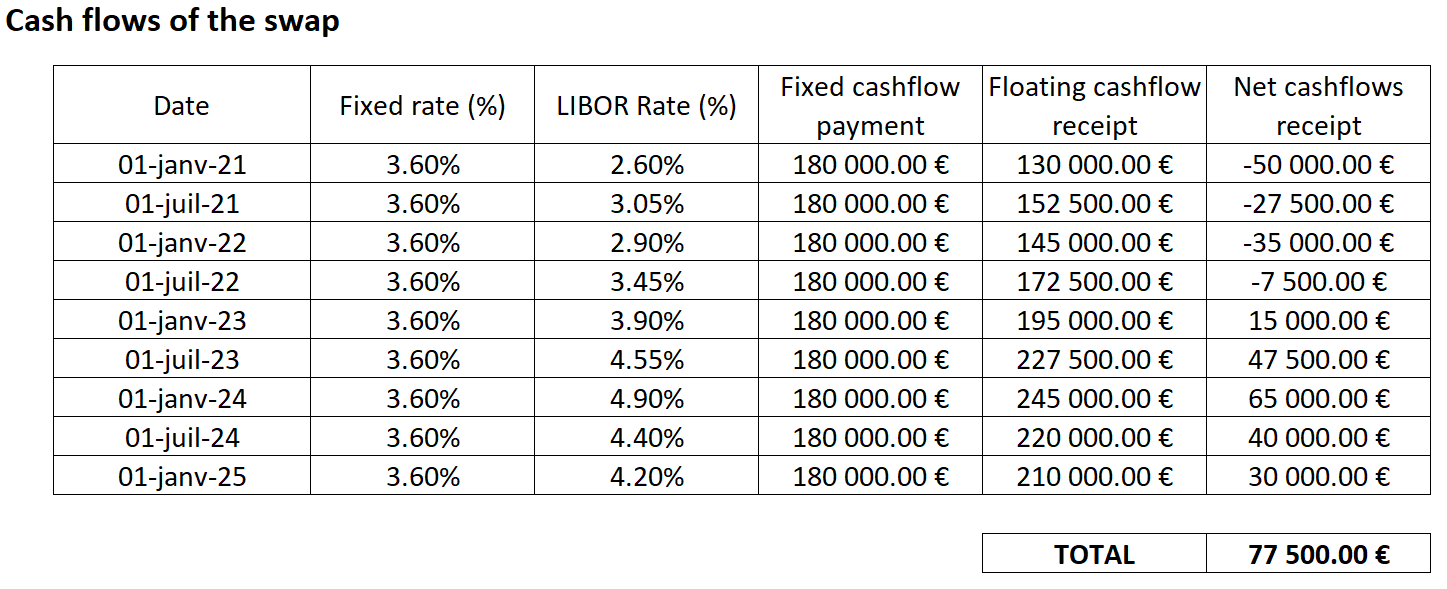
We present below a case study related tot eh behavior of the yield curves in the European fixed-income markets inn the mid 1990’s

The European yield curve differential during in the mid 1990’s

The case showed in this example is the relative-value trade between Germany and Italian yields during the period before the adoption of the Euro as a common currency (at the end of the 1990s). The yield curve should reflect the future path of interest rates. The Maastricht treaty (signed on 7th February 1992) obliged most EU member states to adopt the Euro if certain monetary and budgetary conditions were met. This would imply that the future path of interest rates for Germany and Italy should converge towards the same values. However, the differential in terms of interest rates at that point was nearly 350 bps from 5-year maturity onwards (3.5% spread) as shown in Figure 1.

Figure 1. German and Italian yield curve in January 1995.

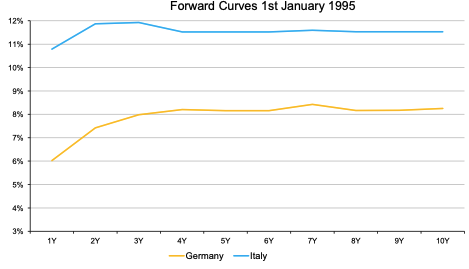
Source: Motson (2022) (Data: Bloomberg).

A fixed-income arbitrageur could have profited by entering in an interest rate swap where the investor receives 5y-5y forward Italian rates and pays 5y-5y German rates. If the Euro is introduced, then the spread between the two yield curves for the 5-10y part should converge to zero. As captured in Figure 2, the rates converged towards the same value in 1998, where the spread between the two rates converged to zero.

Figure 2. Payoff of the fixed-income arbitrage strategy.

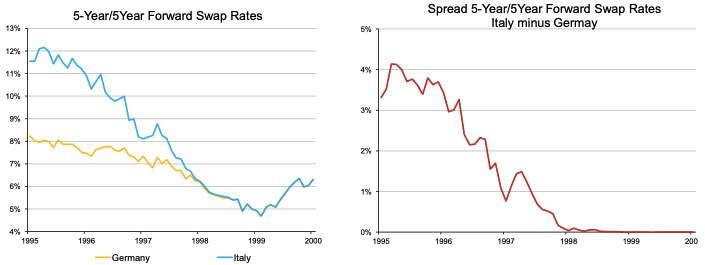
Source: Motson (2022) (Data: Bloomberg).

Performance of the fixed-income arbitrage strategy

Overall, the performance of the fixed-income arbitrage between 1994-2020 were smaller on scale, with occasional large drawdowns (Asian crisis 1998, Great Financial Crisis of 2008, Covid-19 pandemic 2020). This strategy is skewed towards small positive returns but with important tail-risk (heavy losses) according to Credit Suisse (2022). To capture the performance of the fixed-income arbitrage strategy, we use the Credit Suisse hedge fund strategy index. To establish a comparison between the performance of the global equity market and the fixed-income arbitrage strategy, we examine the rebased performance of the Credit Suisse index with respect to the MSCI All-World Index.

Over a period from 2002 to 2022, the fixed-income arbitrage strategy index managed to generate an annualized return of 3.81% with an annualized volatility of 5.84%, leading to a Sharpe ratio of 0.129. Over the same period, the Credit Suisse Hedge Fund index Index managed to generate an annualized return of 5.04% with an annualized volatility of 5.64%, leading to a Sharpe ratio of 0.197. The results are in line with the idea of global diversification and decorrelation of returns derived from the global macro strategy from global equity returns. Overall, the Credit Suisse fixed-income arbitrage strategy index performed better than the MSCI All World Index, leading to a higher Sharpe ratio (0.129 vs 0.08).

Figure 3 gives the performance of the fixed-income arbitrage funds (Credit Suisse Fixed-income Arbitrage Index) compared to the hedge funds (Credit Suisse Hedge Fund index) and the world equity funds (MSCI All-World Index) for the period from July 2002 to April 2021.

Figure 3. Performance of the fixed-income arbitrage strategy.
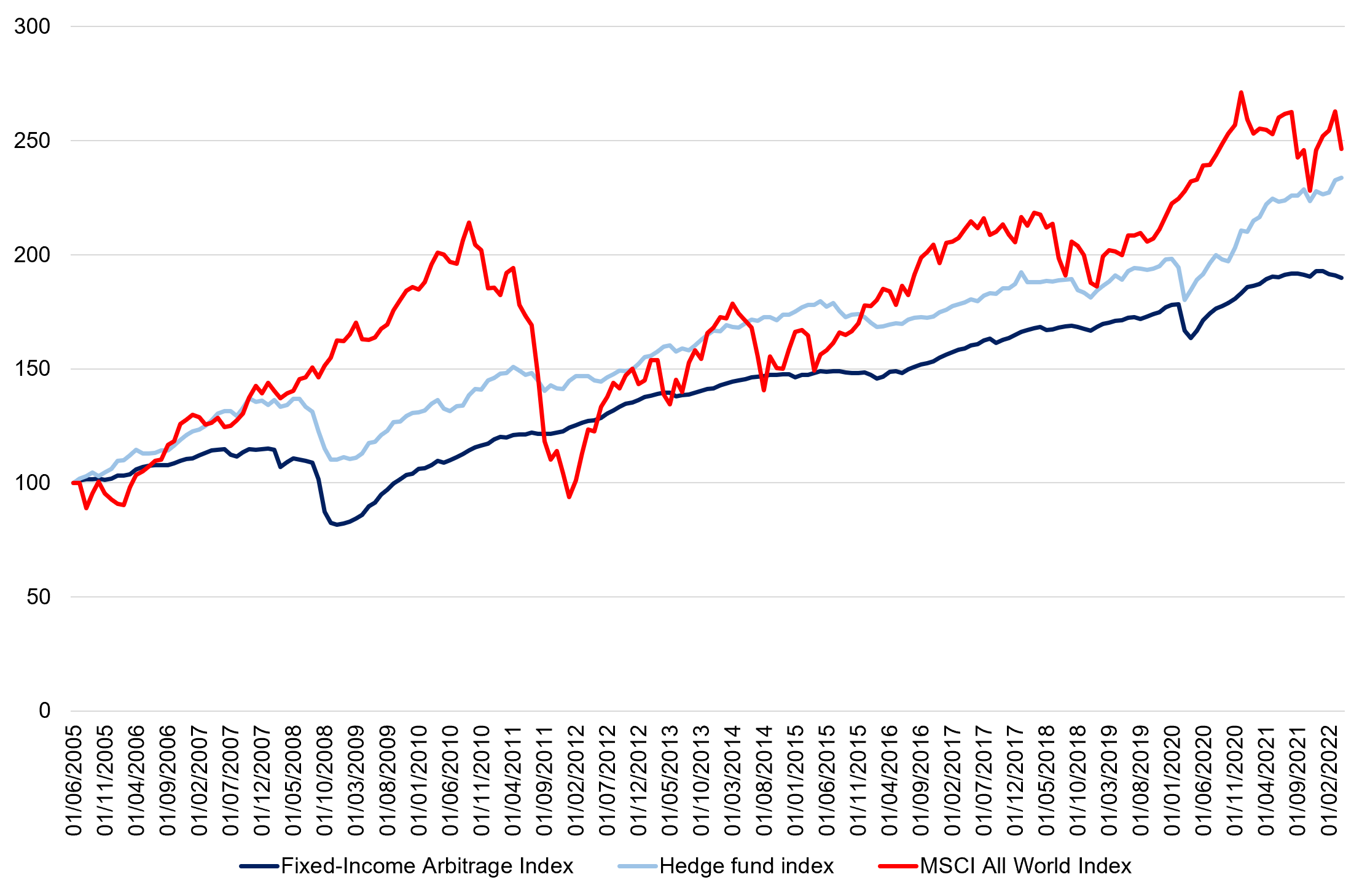
Global macro performance
Source: computation by the author (Data: Bloomberg).

You can find below the Excel spreadsheet that complements the explanations about the fixed-income arbitrage strategy.

Why should I be interested in this post?

The fixed-income arbitrage strategy aims to profit from market dislocations in the fixed-income market. This can be implemented, for instance, by investing in inexpensive fixed-income securities that the fund manager predicts that it will increase in value, while simultaneously shorting overvalued fixed-income securities to mitigate losses. Understanding the profits and risks associated with such a strategy may aid investors in adopting this hedge fund strategy into their portfolio allocation.

Useful resources

Academic research

Pedersen, L. H., 2015. Efficiently Inefficient: How Smart Money Invests and Market Prices Are Determined. Princeton University Press.

Motson, N. 2022. Hedge fund elective. Bayes (formerly Cass) Business School.

Business Analysis

Credit Suisse Hedge fund strategy

Credit Suisse Hedge fund performance

Credit Suisse Fixed-income arbitrage strategy

Credit Suisse Fixed-income arbitrage performance benchmark

About the author

The article was written in January 2023 by Youssef LOURAOUI (Bayes Business School, MSc. Energy, Trade & Finance, 2021-2022).

This article written by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022) presents the derivative contract of interest rate swaps used to hedge interest rate risk in financial markets.

Introduction

In financial markets, interest rate swaps are derivative contracts used by two counterparties to exchange a stream of future interest payments with another for a pre-defined number of years. The interest payments are based on a pre-determined notional principal amount and usually include the exchange of a fixed interest rate for a floating interest rate (or sometimes the exchange of a floating interest rate for another floating interest rate).

While hedging does not necessarily eliminate the entire risk for any investment, it does limit or offset any potential losses that the investor can incur.

Forward rate agreements (FRA)

To understand interest rate swaps, we first need to understand forward rate agreements in financial markets.

In an FRA, two counterparties agree to an exchange of cashflows in the future based on two different interest rates, one of which is a fixed rate and the other is a floating rate. The interest rate payments are based on a pre-determined notional amount and maturity period. This derivative contract has a single settlement date. LIBOR (London Interbank Offered Rate) is frequently used as the floating rate index to determine the floating interest rate in the swap.

The payoff of the contract is as shown in the formula below:

(LIBOR – Fixed Interest Rate) * Notional amount * Number of days / 100

Interest rate swaps (IRS)

An interest rate swap is a hedging mechanism wherein a pre-defined series of forward rate agreements to buy or sell the floating interest rate at the same fixed interest rate.

In an interest rate swap, the position taken by the receiver of the fixed interest rate is called “long receiver swaps” and the position taken by the payer of the fixed interest rate is called “long payer swaps”.

How does an interest rate swap work?

Interest rate swaps can be used in different market situations based on a counterparty’s prediction about future interest rates.

For example, when a firm paying a fixed rate of interest on an existing loan believes that the interest rate will decrease in the future, it may enter an interest rate swap agreement in which it pays a floating rate and receives a fixed rate to benefit from its expectation about the path of future interest rates. Conversely, if the firm paying a floating interest rate on an existing loan believes that the interest rate will increase in the future, it may enter an interest rate swap in which it pays a fixed rate and receives a floating rate to benefit from its expectation about the path of future interest rates.

Example

Let’s consider a 4-year swap between two counterparties A and B on January 1, 2021. In this swap, counterparty A agrees to pay a fixed interest rate of 3.60% per annum to counterparty B every six months on an agreed notional amount of €10 million. Counterparty B agrees to pay a floating interest rate based on the 6-month LIBOR rate, currently at 2.60%, to Counterparty A on the same notional amount. Here, the position taken by Counterparty A is called long payer swap and the position taken by Counterparty B is called the long receiver swap. The projected cashflow receipt to Counterparty A based on the assumed LIBOR rates is shown in the below table:

Table 1. Cash flows for an interest rate swap.

Source: computation by the author

In the above example, a total of eight payments (two per year) are made on the interest rate swap. The fixed rate payment is fixed at €180,000 per observation date whereas the floating payment rate depend on the prevailing LIBOR rate at the observation date. The net receipt for the Counterparty A is equal to €77,500 at the end of 5 years. Note that in an interest rate swap the notional amount of €10 million is not exchanged between the counterparties since it has no financial value to either of the counterparties and that is why it is called the “notional amount”.

Note that when the two counterparties enter the swap, the fixed rate is set such that the swap value is equal to zero.

Excel file for interest rate swaps

You can download below the Excel file for the computation of the cash flows for an interest rate swap.

▶ Jayati WALIA Derivative Markets

▶ Akshit GUPTA Forward Contracts

▶ Akshit GUPTA Options

Useful resources

Hull J.C. (2015) Options, Futures, and Other Derivatives, Ninth Edition, Chapter 7 – Swaps, 180-211.

www.longin.fr Pricer of interest swaps

About the author

Article written in December 2022 by Akshit GUPTA (ESSEC Business School, Grande Ecole Program – Master in Management, 2019-2022).